Abstract

Uniform Expansion of the Thermonuclear Reaction Rate Formula^* * Supported in part by the CNPq.

M.S. Hussein and M.P. Pato

Nuclear Theory and Elementary Particle Phenomenology Group

Instituto de Física, Universidade de São Paulo

CP 66318, 05389-970 São Paulo, SP, Brazil

Received April 19, 1997

The nuclear reaction rate formula used in stellar evolution calculation is carefully examined. A uniform expansion is performed up to fourth order in the relevant physical parameter. The convergence of the series is assessed and a semianalytical expression is derived, valid for all values of the expansion parameter. The formulae with electron screening and plasma dissipative collision effects are also evaluated. The agreement of the approximate analytical formulae with the exact result is excellent over a wide range of values of the parameters.

I. Introduction

To obtain the rate of nuclear energy production in the stellar interior one integrates the nuclear reaction cross section over the thermal distribution of nuclei. Closed expressions for the rate formula are desirable for speedy calculation of the stellar evolution and element abundances. It is a common practice to obtain these expressions within the Gaussian approximation [1]. Recently, Anderson, Haubold and Mathai [2] have developed a series expansion of the rate integrals using the gamma function and complex integration. In this paper we develop a new type of series based on a judicious choice of Gaussian mapping and using the method of uniform approximation. The series we obtain are rapidly convergent and avoid several of the singularities encountered in [2]. Further, the different terms in the series are explicitly given in terms of the parameters of the integral (electron screening energy, Gamow energy, etc.).

Our results are certainly relevant for the calculation of the rate of the reaction ¹²C (a, g)¹⁶O ® in massive stars with . This last reaction is known to be dominated by nearby above-threshold and sub-threshold resonances making the astrophysical S -factor deviate considerably from a second order expansion in energy. It is known that the rate of this reaction, which dominates the scenario of the evolution of a massive star into a type II supernova, should be known to better than 20% [1]. Thus more precise analytical closed formulae are welcome here.

The paper is organized as follows. In Section II the reaction rate formula for bare nuclei are calculated to fourth-order in the Gamow energy. The effect of electron screening and plasma collision loss are then considered and the corresponding integrals are evaluated in Section III. Finally in Section V we discuss our result in connection with the rates of several reactions and give some concluding ramarks.

II. II. Expressions for the thermonuclear reaction rate formula

The reaction rate formula is given by

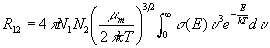
where N_i is the number density of nucleus i , m_m is the reduced mass of the two-nucleus system, kT is the thermal energy and v is the relative velocity. Changing variables from v to E through , and using the usual low-energy form of the cross section,

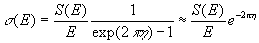where is the Sommerfeld parameter, we have, with

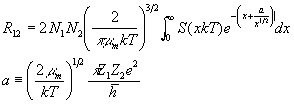
For non-resonant reactions, S(E) is slowly varying and may be expanded

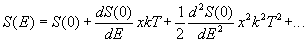Therefore Eq. (3) may be written as

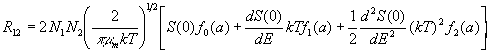where the functions f_n(a) are defined by

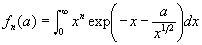Setting y º x ^{n + 1}, we can rewrite (6) as

.

Thus an exact series representation of R₁₂ is

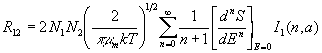In the following, we develop the uniform series for f_n(a) . For this purpose we recall some known facts about the uniform expansion of integrals of the general form [3],

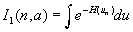
where H_n(u) is given by

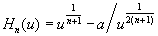which has an extremum at . Introducing the mapping

where t = 0 correspondents to with u_n being the position of the extremum, given above, we can now write

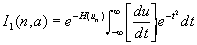Expanding in powers of t and integrating, we find

where the first four terms in the sum are

, (14)

where . From Eq. (10), we then find

(15)

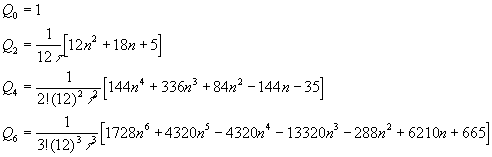
The integral of Eq. (6) can now be evaluated straightforwardly using the above formulae. The functions f₀(a), f₁(a) and f₂(a) are then easily found, up to the fourth order term,

(16)

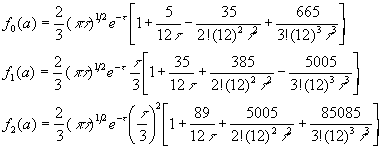
where

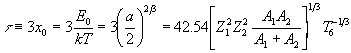where Eis the Gamow energy.

In Fig. 1 we show the relative error of (16) in comparison with the exact numerical result. It is clear that (16) is an excellent approximation.

The expression for f₀(a) up to the third term has been evaluated by Salpeter [4] and is quoted in textbooks [1]. The first term in f₁(a) was calculated by Bahcall [5]. The other terms in fand f₁ and the function f₂(a) are new results. We feel that f₁ and f₂ (and possibly other integrals) may be important for stellar evolution calculation involving nuclear reactions near threshold when S(E) would have significant energy variation.

Figure 1. The relative error defined as |f_{n, exact}(t) - f_{n, Eq. (16)}(t)|/f_{n, exact}(t) vs. t. The small oscillations are numerical uncertainty in the evaluation of f_{n, exact}(t) .

In order to assess the convergence of the series in (6), we consider the complex solutions of the equation that determines the position of the maximum in the integrand of Eq. (6). The following discussion applies to any n . We therefore consider the case n = 0 . The extremum condition reads

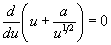We find one real solution, which was used previously in evaluating the integral, , and two complex conjugate solutions given by

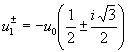The function, H(u) , acquires the following values at uand

(20) . (21)

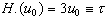
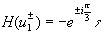The radius of convergence is measured by [3]

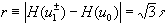Thus, the series is convergent up to the term of order , which is usually large for typical values of the parameters t (say, 10). Recent work of Berry [6], shows how to estimate the rest of the series starting at the 'th term.

Figure 2. The integral I₁(n,a) vs. a . The upper curve is that exact result which is reproduced with our formula. The lower curve in the result of Ref. [2].

In Fig. 2 we show the result of our calculation of I₁ , for S = S(0) , based on Equation (13), taking up to the fourth order terms in f₀(a) , compared to the exact numerical integration result (difficult to distinguish from the approximate one) for a wide range of value of t. The lower curve in the figure is the result of Ref. [2]. It is clear that our procedure is quite accurate.

III. Effect of electron screening and plasma dissipation

We turn now to the more realistic case that includes the effect of electron screening. This effect can be taken into account by a shift in the energy of the fusing nucleus, E_c.m.® E_c.m. + U_e when U_e is roughly given by , with R_a being the atomic radius. The shielded nuclei clearly feel a lower Coulomb barrier when they fuse, which is equivalent to the energy shift above. The value of U_e varies with the system. For p + p , U_e ~ 29eV, when as for a + ¹²C, U_e = 2.07 keV.

With screening, the thermonuclear reaction rate integral becomes (using the rotation of Ref. [2])

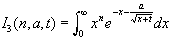where . The evaluation of (23) proceeds in manner similar to that used for I₁ albeit with a different mapping procedure. The details can be found in Appendix I. The final result which we quote here is

where

In the above, erfc is the complementary error function and

In Appendix I we give explicit forms of the first two coefficients, band b₁ . Note that if t > whereas for t < y₀ . Further, in the limit of no screening t = 0 and x = - ¥, which gives the desired check of Eq.(24)

The above form for I₃ is calculated for n = 0 , up to second order in the series of Eq. (24). The result is compared with the exact (again not distinguishable) result in Fig. 3. Also shown is the lowest order approximation which is given by

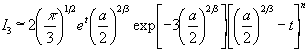
From Fig. 3 we see clearly that by performing the integral using the uniform approximation and keeping only the first two leading terms one approaches very closely the exact result. The rough estimate of Eq. (25), diverges at .

Before ending this section we mention that dissipative collision processes in the stellar plasma result in a cut off of the high energy part of the Maxwell-Boltzamann distribution at a certain high energy E_d = kTd . This means that the integral I₁ , is modified to

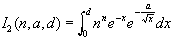
The above integral is easily related to I₁ , and I'₃ through

or

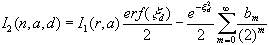where erf is the error function.

Figure 3. The integral I₃(n,a,t) vs. a and t . See text for details. The asymptotic result is shown as the broken (lower) line. The upper full curve is the exact and our results including band b₁ . They are practically indistinguishable. The result with bonly is the lower curve.

IV. Discussion and conclusions

The mehod of calculation developed here allows a fast computation of the thermonuclear reaction rate using analytical formulae and a simple way of estimating the relative error when compared to the exact numerical calculation of the integral. Further, our method may allow a way of treating the wide resonance cases encountered in, e.g., the ¹²c(a, g)¹⁶0 reaction in massive stars.

We first consider two reactions of great importance to the solar neutrino problem and the supernova event: p + p ® d + e ⁺ + v (T₆ = 15) and ³He + ⁴He ® ⁷Be + g (T₆ = 5000) In the first reaction the Gamow energy which gives for , while the calculated S -factor is given by [1]

The Q -value is 1.44 MeV.

In the second reaction, the Gamow energy is and then a = 106.455 while the measured S -factor in [1]

We have used our approximate formulae for the non-resonant reaction rate, Eq. (5) with f₀(a) and f₁(a) given by Eq. (16).

The values we obtain for the rate R₁₂ are 1.1995 x 10^{- 43}cm ³/s and 8.81 x 10^{- 35}cm ³/s respectively. It is instructive to measure the relative error from Fig. 1. For the p + p reaction, a = 19.63 and f₀(19.63) = 5.101 x 10^{- 6} and f₁(19.63) = 2.758 x 10^{- 5} . The relative error in f₀ is (t = 13.691) about 8 x 10^{- 6} , whereae in f₁ it is 2 x 10^{- 4} , quite small. In the ³He + ⁴He reaction, a = 106.455 and f₀(106.455) = 2.85 x 10^{- 18}, f₁(106.455) = 4.7 x 10^{- 17} . The relative error in fis (t = 42.45) is 10^{- 6} and f₁ it is 2 x 10^{- 5} , again quite small. The great accuracy of our approximate formulae may be of value in the solar neutrino problem [7] where it is expected that better accuracy with which S(0),S'(0) and S''(0) are extracted from the data will be attained in the future.

A more stringest test of our formulae is supplied to the so-called wide resonance case such as the one encountered in the ¹⁷C(a, g)¹⁶0 reaction in massive stars. Here the S -factor deviates considerably from the polynomial of Eq. (4). In fact the measurement [8] shows that over the energy range 1.3 < E_c.m.(MeV) < 3.5 the S -factor has a Lorentzian shape. When extrapolated to the relevant astrophysical energy (Gamow energy) of 0.3 KeV (T₉ = 0.1 - 0.2 ) using, reasonable theory, the final resulting S -factor has the shape of a Lorentzian peaked at E_c.m. = 2.4 MeV which sits on a background that has the shape of a Gaussian e ^{- bE2} . The Gaussian background can be handeled using the method of consecutive mappings while the Lorentzian can be evaluated using the method of residues. We leave full discussion of this problem to a future work.

Appendix -- asymptotic series of the integral

Although the value of the screening energy, t , relevant for stellar calculation is small compared t the Gamow energy, it is still useful to find an analytical form for I₃ (n,a,t) which is valid for a large range of values of t . The result for I₃ which we derived and discussed in Section,

is derived in this Appendix. The sum is rapidly convergent. In fact the result shown in Fig. (3) was obtained with only the first two terms.

The integral we wish to calculate is

A change of variable y º x + t renders I₃ into

. (A.3)

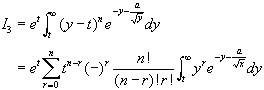Thus what is needed is the calculation of

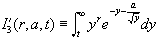
As was done in the calculation of I₁ we introduce the following mapping

where

and F(y₀) = 3y₀ . Then

Let us call the function . We introduce now another mapping

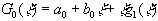
with g₁(x) = 0 . As we show below, explicit knowledge of the function g₁(x) is not required. With (A.9 ) we have

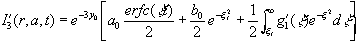where an integration by parts has been carried out which allowed writing the last term in its present form. By repeated application of the mapping and integration by parts one can generate the series, vis

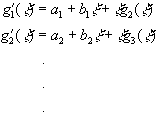
with g₂(x) = g₃(x) = ... = 0 .

Thus

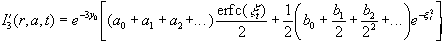Clearly in the limit t = 0, x_t = - ¥ and the above function becomes

I '₃(r,a,0) = e ^{- 3y}0(a₀ + a₁ + a₂ + ... ) ,

which is nothing but I₁(r,a) . Accordingly we obtain Eq. (24). Now we turn for the calculation of the coefficients a₀,a₁,... and b₀,b₁,... .

Clearly

and

(A.9)

where G ⁽ⁿ⁾ = d ⁿG/dxⁿ which can be calculated from the defining relations

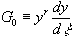
and

For example, to evaluate a₀,a₁,band b₁ one needs to know G ⁽⁰⁾(0), G ⁽⁰⁾(x), G ⁽¹⁾₀(x_t) and G ⁽²⁾(0) . Then

. (A.11)

The derivatives can be obtained from F(y) . Since by definition, we have

In the above equations, are given by

and

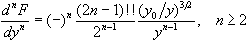The above formulae were employed in the derivation of the series expansion for I₁(n,a) (Section II) and I₂(n,a,d ) and I₃(n,a,t) (Section III).

References

[1] See e.g., Claus E. Rolfs and William S. Rodney, "Cauldrons in the Cosmos: Nuclear Astrophysics'', The University of Chicago Press (1988) Chapter 4 (pages 150-189).

[2] W.J. Anderson, M.J. Haubold, and A.M. Mathai, Astr. and Space Science 214, 49 (1994).

[3] R.B. Dingle, "Asymptotic Expansions: their derivation and interpretation'', New York & London, Academic Press (1973).

[4] E.E. Salpeter, Phys. Rev. 88, 547 (1952).

[5] J.N. Bahcall, Ap. J. 143, 259 (1966).

[6] M.V. Berry, Proc. R. Soc. London A422, 7 (1989); M.V. Berry and C.J. Howls, Proc. R. Soc. London A434, 657 (1991).

[7] J.N. Bahcall, "Neutrino Astrophysics'' Cambridge V. Press (1990).

[8] A. Redder et al. Nucl. Phys. A462, 385 (1987)

Publication Dates

History