Abstract
Equilibrium constant and mass transfer parameters are needed for the study of amino acid separation in any process involving adsorption in fixed beds. The adsorption constants, effective diffusion coefficients, and axial dispersion coefficients for two amino acids, L-phenylalanine (Phe) and L-tyrosine (Tyr), are determined from a series of pulse tests in a fixed bed packed with PVP (poly-4-vinylpyridine) resin. Total bed voidage at different flow rates is estimated from NaCl pulse test data. The effective pore diffusivities of Phe, Tyr, and NaCl are estimated from moment analysis of pulse data. A detailed rate model is then solved numerically and adsorption constants, effective diffusion coefficients, axial dispersion coefficients are determined by moment analysis and compared with the pulse data. The advantage of this method is that the effective intraparticle diffusivities can be determined without the influence of extracolumn dispersion or intracolumn axial dispersion effects.
Equilibrium constant; mass transfer parameters; moment analysis of pulse data
PARAMETERS ESTIMATION FOR AMINO ACIDS ADSORPTION IN A FIXED BED BY MOMENT ANALYSIS
M.A.Cremasco1, B.J.Hritzko2, Yi Xie2, and N.H. L.Wang2
1School of Chemical Engineering, State University of Campinas,
PO Box 6066, 13083-970, Campinas, Brazil
E-mail: cremasco@feq.unicamp.br 2Chemical Engineering Department, Purdue University
47907-1283, West Lafayette, U.S.A.
E-mail: wangn@ecn.purdue.edu
(Received: January 2, 2001; Accepted: June 13, 2001)
Abstract - Equilibrium constant and mass transfer parameters are needed for the study of amino acid separation in any process involving adsorption in fixed beds. The adsorption constants, effective diffusion coefficients, and axial dispersion coefficients for two amino acids, L-phenylalanine (Phe) and L-tyrosine (Tyr), are determined from a series of pulse tests in a fixed bed packed with PVP (poly-4-vinylpyridine) resin. Total bed voidage at different flow rates is estimated from NaCl pulse test data. The effective pore diffusivities of Phe, Tyr, and NaCl are estimated from moment analysis of pulse data. A detailed rate model is then solved numerically and adsorption constants, effective diffusion coefficients, axial dispersion coefficients are determined by moment analysis and compared with the pulse data. The advantage of this method is that the effective intraparticle diffusivities can be determined without the influence of extracolumn dispersion or intracolumn axial dispersion effects.
Keywords: Equilibrium constant ,mass transfer parameters, moment analysis of pulse data.
INTRODUCTION
Amino acids are the basic structure of proteins. L-phenylalanine (Phe), C6H5CH2CH(NH2)CO2H, and L-tyrosine (Tyr), C6H4OHCH2CH(NH2)CO2H, for example, are present in human milk and hemoglobin, in egg albumin, and in structure of insulin (Fruton and Simmonds, 1963; McGilvery, 1970). Usually, amino acids are separated by batch ion-exchange chromatography (Dechow, 1989). However, recent studies have shown that the simulated moving bed (SMB) system obtains higher throughput, recovery, and purity than the batch chromatography system. SMB systems can separate similar solutes with low solvent consumption and high sorbent utilization. Hashimoto et al. (1989) have used a three zone SMB to separate NaCl and Phe using the Amberlite XAD-7 resin. Wu et al. (1998) have separated Phe and L-tryptophan (Trp) using poly-4-vinylpyridine (PVP) resin in a pilot-scale SMB system with four zones. Independent of separation process applied to amino acids, it is essential to know the basic parameters associated with any process, such as isotherms and mass transfer parameters.
Moment analysis is a useful tool for determining axial diffusion coefficients and effective pore diffusivities from pulse experiments. This technique consists of analyzing the solute concentration as a function of time at the outlet of a fixed bed in response to the solute concentration pulse at the entrance of the bed. For dilute solutions, the solid-liquid equilibrium curve can be represented by a straight line (Henrys law). The slope of this straight line can be determined from the first moment of a solute pulse, which is associated with solute retention time (Miyabe and Suzuki, 1992). Mass transfer parameters can be obtained from the second moment (Schneider and Smith, 1968; Suzuki and Smith, 1971; Ruthven, 1984). This technique was used for gaseous mixtures (Heynes Jr., 1975; Kumar et al., 1982) and was later applied to liquid mixtures (Ho et al., 1987; Miyabe and Suzuki, 1992). Koh et al. (1998) applied this technique to the study of adsorption of Phe on an ion exchange resin. These authors calculated the intracolumn dispersion coefficient was from the Chung and Wen correlation (1968), and non ideal packing effects were neglected in the estimation of the effective diffusion coefficient.
In this paper the moment analysis method is used to determine adsorption constants, effective diffusion coefficients, and axial dispersion coefficients of two amino acids, L-phenylalanine and L-tyrosine, in dilute aqueous solutions in a fixed bed of PVP (poly-4-vinylpyridine) resin. Particle porosity is obtained from the manufacturers literature. Total bed voidage is determined from the first moments of eluted peaks of a non absorbing specie (3 g/l NaCl in feed) at different flow rates. The mass transfer parameters are found by simultaneous analysis of the first and second moments of the amino acids elution peaks. Experimental chromatograms at different flow rates agree closely with numerical solutions of a detailed rate model based on the estimated parameters.
THEORY
Model
In order to apply moment analysis to a pulse response curve to estimate linear adsorption constants and mass transfer parameters, the following assumptions are made:
i) The mobile phase is a dilute solution.
ii) Velocity is constant throughout column cross-section.
iii) No chemical reactions occur.
iv) Henrys law describes solute uptake. Adsorption of NaCl is negligible.
v) Intraparticle diffusion is described by pore diffusion. For a linear isotherm system, the flux due to surface diffusion, if important, can be lumped together with the pore diffusion flux (Ma et al., 1996).
vi) External mass transfer from the bulk liquid to the pores is described by film mass transfer.
vii) Axial dispersion effects are considered.
Based on these hypotheses the following equations are obtained (Schneider and Smith, 1968):
Solute mass balance in the mobile phase:
Solute mass balance in the pore phase:
The axial dispersion coefficient, Eb, and the effective pore diffusivity, Dp, are the same as defined by Ruthven (1984).
The initial and boundary conditions that describe a pulse injection into a chromatographic column are given as follows (Schneider and Smith, 1968):
The first and the second absolute moments are associated with the height and the width of an elution peak, respectively. These parameters are defined as follows (Miyabe and Suzuki, 1992):
Many authors (Schneider and Smith, 1968; Heynes, 1975) applied the Laplace-Carson transform to the solute continuity equations, Eqs.(1) and (2), and obtained analytical solutions for pulse elution curves. By substituting the analytical solution into Eqs.(10) and (11), one can obtain the explicit expressions for the first and second moments, respectively,
where
Determination of Bed Porosity, Adsorption Constants, and Mass Transfer Parameters
For a system with linear adsorption isotherms, the efficiency of a chromatographic column can be evaluated by a height equivalent to that of a theoretical plate (HETP), which is defined according to the Van Deemter equation as follows:
where
where m0 and s0 include, respectively, the delay in retention and dispersion due to extra column dead volume. Substituting Eqs.(12) and (13) into definition (14), one obtains:
in which
Rearranging Eq.(17), one obtains the following expression:
where the modified HETP is defined by
with
From the slop of the straight line (a) and the intercept (b) of Eq.(19) one can obtain the effective diffusion coefficient and the axial dispersion coefficient, respectively. The film mass transfer coefficient in Eq.(20) is obtained using the Wilson and Geankoplis correlation, which is valid for liquid systems in which 0.0015< Re p <55 (Wilson and Geankoplis, 1966):
It is important to note that the experimental values of the first and second absolute moments are obtained by numerical integration of the chromatographic elution curve (Eqs.(9) and (10)). However, the value for bed voidage is needed in Eqs.(20) and (22). In this work, total bed voidage is determined by a pulse test of NaCl (which does not adsorb on PVP) moving through the packed bed, and particle porosity is obtained from the literature (Wu et al., 1998). The relation between the retention time of NaCl and bed voidage is as follows:
where retention time is equal to the first moment. Partition coefficients for Phe and Tyr are then obtained from the following equation:
EXPERIMENTAL
The experimental set-up is illustrated in Figure 1. The system consists of two Pharmacia P-500 low-pressure pumps, a Pharmacia LCC-500 controller, and a Pharmacia MV-7 injection valve. For data acquisition a Waters 990 photodiode array detector was used, and the data were processed using the Waters 900 software. A Pharmacia glass column with a length of 12.5 cm and an internal diameter of 1.5 cm was used. The column was packed with Reillex.-HP resin, which is poly-4-vinylpyridine cross-linked with divinylbenzene (PVP), purchased from Reilly Industries, Inc., Indianapolis, USA. The average particle diameter and average particle porosity are 0.036 cm and 0.55, respectively. The PVP resin was chosen because it is physically stable and easy to regenerate. The solutes studied were Phe and Tyr, with purities of 99% and 98%, respectively.
Chromatographic elution curves were obtained using injection of small pulses (0.50 ml) of aqueous solution of 3.0 g/l of NaCl, 2.0 g/l of Phe, and 0.35 g/l of Tyr, respectively. All experiments were performed at 1 atm and 25ºC. The solution was injected into the column using a rotary valve and an injection loop (Figure 1). The superficial velocity of the eluent varied from 0.57 cm/min to 1.41 cm/min, which corresponded to particle Reynolds numbers from 0.035 to 0.087. The effluent was monitored in the ultraviolet wavelength range: 200 nm for NaCl, 260 nm for Phe, and 300 nm for Tyr.
RESULTS AND DISCUSSION
Determination of Bed Porosity and Partition Coefficients.
Four experiments were performed at different flow rates for each solute. However, before these experiments could be performed it was necessary to determine the m0 value related to extracolumn dead volume, which is the volume associated with connecting tubes from the sample injector up to the detector inlet in the absence of a column. A pulse of 2.0 g/l Phe was introduced into the "dead volume" at 0.5 ml/min. The procedure was repeated with 0.35 g/l Tyr. The dead volume was determined by
where tm is the retention time of the pulse at mass center, which is equal to 1.30 min. for Phe, and 1.38 min. for Tyr. For future comparison between experimental data and simulations the retention time is corrected by (Miyabe and Suzuki, 1992)
An aqueous solution of NaCl was used to find the bed porosity, e . Four experiments were run in order to obtain chromatographic elution curves at different flow rates. The first moment, which is the retention time of each pulse, was found by integration of the experimental pulse response curve. A graph of ( ) vs. v0 (Figure 2) was constructed, according to Eq. (24). Bed porosity was determined from the slope of the straight line associated with this graph. The correlation coefficient of this line is r2 = 0.9992. One can calculate from Eq.(24) that e = 0.37.
) vs. v0 (Figure 2) was constructed, according to Eq. (24). Bed porosity was determined from the slope of the straight line associated with this graph. The correlation coefficient of this line is r2 = 0.9992. One can calculate from Eq.(24) that e = 0.37.
The Phe and Tyr adsorption constants were determined using a similar procedure. In this case, Eq.(25) was used instead of Eq.(24). The retention time results are shown in Figure 2. The Phe and Tyr correlation coefficients were r2 =0.99999 and r2 = 0.99994, respectively. The calculated partition coefficients were kp =1.947 for Phe and kp =3.229 for Tyr. The Phe value found in this work is 21% higher than the value ( kp =1.61 ) reported by Wu et al. (1998). This could be due to a lower concentration range in this study. The outlet concentrations in this paper range from 0.17 to 0.50 g/L, compared to those from 0.8 to 2.0 g/L in Wu et al. (1998). For an asymmetric solute, the competition between energetic factors and steric factors leads to a gradual transition from side-on orientation at low coverage to end-on orientation at high coverage. As a result, the apparent Henrys constant of an asymmetric solute can decrease with increasing solution concentration as reported by Jin et al. (1999). Heterogeneous adsorption sites can also result in an apparent decrease in the Henrys constant with increasing solution concentration (Myers and Byington, 1986).
Determination of Mass Transfer Parameters
The second moments can be determined from the experimental elution curves, as shown in Eq.(10). In order to obtain the HETP defined in Eq.(14), the contribution related to extracolumn dispersion was subtracted from the second moment. Since the particle Reynolds number in this study is small, ranging from 0.035 to 0.087, Eq.(23) was used to estimate kf.
The diffusion coefficient of NaCl (3 g/l) in water was estimated using the procedure described by Cremasco (1998) for the calculation of electrolyte diffusion coefficients in liquid solutions. The value was found to be 9.2´10-4 cm2
/ min. In calculating the diffusion coefficients of the amino acids, it was assumed that they were in dilute aqueous solutions and that their diffusivity values were independent of concentration . The dilute diffusion coefficient of Phe in water was reported by Paduano et al. (1990) as 4.24´10
-4 cm
2 / min. To estimate the diffusion coefficient of Tyr, the Wilke and Chang correlation was used, and it was assumed that the solute concentration does not influence solution viscosity. Since both solutions are at the same temperature,
The values for molar volume at the normal boiling temperature,  and
and  , were calculated from La Bas's volume (Cremasco, 1998). The diffusion coefficient calculated for Tyr was 4.12´10-4 cm2
, were calculated from La Bas's volume (Cremasco, 1998). The diffusion coefficient calculated for Tyr was 4.12´10-4 cm2
/ min.
After calculating the film mass transfer resistance, one can determine the modified HETP using Eq.(20) and construct a graph of (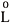 vs. v ) for NaCl, Phe, and Tyr, as shown in Figure 3. The results from these graphs are straight lines; the correlation coefficients are r2 = 0.985, r2 =0.996, and r2 = 0.996 for NaCl, Phe, and Tyr, respectively. These results show the good agreement between the modified HETP experimental results and those from the model proposed in this work. From the slopes and intercepts of the straight lines in Figure 3 the effective diffusion coefficients and axial dispersion coefficients can be determined independently, and their values are shown in Table 1.
vs. v ) for NaCl, Phe, and Tyr, as shown in Figure 3. The results from these graphs are straight lines; the correlation coefficients are r2 = 0.985, r2 =0.996, and r2 = 0.996 for NaCl, Phe, and Tyr, respectively. These results show the good agreement between the modified HETP experimental results and those from the model proposed in this work. From the slopes and intercepts of the straight lines in Figure 3 the effective diffusion coefficients and axial dispersion coefficients can be determined independently, and their values are shown in Table 1.
Figure 4 shows that the linear dependence of the Dp values for the three solutes, NaCl, Phe, and Tyr, are linearly proportional to their diffusion coefficients, with r2 =0.9998.
Eq.(29) can be compared with a commonly used equation that describes ordinary diffusion in macropores (Ruthven, 1984):
The tortuosity factor for all three solutes is tp = 4, which is within the usual range from 2 to 6 (Miyabe and Suzuki, 1992).
The effective diffusion coefficient values can be compared with those from the Mackie and Meares correlation (1955). This correlation was developed from the effective diffusivities of ions in membranes.
Observe that product ep Dp in Eq.(31) is equivalent to the effective diffusivity in the original Mackie and Meares correlation (1955). In this paper, Ruthvens definition of Dp has been used for diffusion flux in Eq.(2). The values for Dp calculated from Eq.(31) are in good agreement with the experimental values (Table 1).
The axial dispersion coefficients presented in Table 1 show their dependence on solution interstitial velocity and solute characteristics and that they are related to the diffusion coefficients (9.2´10-4 cm2
/ min for NaCl, 4.24´10
-4 cm
2 / min for Phe, and 4.12´10
-4 cm
2 / min for Tyr). The following relationship can be written:
The relationship presented in Eq.(32) is generally found in many correlations in the literature (Table 2), except for the Chung and Wen correlation, which does not account for the influence of DAB on axial dispersion in packed beds. Figure 5 compares Eb from experiments and Eb estimated from the correlations. All Eb values estimated from the correlations are smaller than the experimental Eb values. This difference could be caused by non uniform packing. When one uses moment analysis to determine Dp, dispersion effects due to mixing in extracolumn volume and non ideal packing are independently assessed and subtracted. Therefore, Dp value is less affected by dispersion due to extra column mixing and non ideal packing. For this reason, this method is more rigorous than the methods for amino acids in the literature (Wu et al., 1998; Koh et al., 1998), where Eb values are estimated by the Chung and Wen correlation and dispersion due to non ideal packing and extracolumn effects is ignored.
VALIDATION OF THE RESULTS
In order to validate the parameters estimated using the method of moment analysis, simulations based on the parameters were compared with the experimental chromatograms. The governing equations, Eqs.(1) and (2), were solved by the method of orthogonal collocation on finite elements in the spatial direction, and the DASPK solver was used in the time domain (Whitley, 1990; Berninger et al., 1991). This numerical method has been used in the studies of many adsorption systems (Ma et al., 1996; Ernest et al., 1997; Koh et al., 1998). The numerical convergence was checked by increasing the number of axial elements from 50 to 100 and by particle points from 2 to 4 and by improving the absolute tolerance from 1´10-3 to 1´10-5 and relative tolerance from 1´10-4 to 1´10-6. The simulation results were the same for 50 axial elements, 4 collocation points in each element and 2 collocation points within the particle, and the absolute tolerance was 1´10-4, while relative tolerance was 1´10-5. The experimental and numerical results of the dimensionless elution curves are shown in Figures (6) to (8).
CONCLUSIONS
Moment analysis is an appropriate method for finding bed porosity, adsorption constants, and mass transfer parameters for linear isotherm systems. A pulse elution curve from the non adsorbing compound NaCl was analyzed to obtain bed porosity, using the first moment of the elution curve. Because the amino acids were in dilute solutions, the adsorption constants for the amino acids could be estimated from the first moment. Mass transfer parameters were determined from the first and second moments. The effective diffusion coefficient, Dp, and the dilute-diffusion coefficient, DAB, could be correlated by a tortuosity factor, Eq.(30), or calculated by the Mackie and Meares correlation, Eq.(31). The value of the axial dispersion coefficient was shown to be dependent on solution interstitial velocity and solute characteristics, which are related to the diffusion coefficient of the solute in solution. The experimental data are in close agreement with numerical simulation results, especially at high flow rates. The advantage of this method is that it minimize the dispersion effects due to extracolumn dead volume and no ideal packing in the determination of effective intraparticle diffusivities.
ACKNOWLEDGEMENTS
Prof. Cremasco acknowledge the financial assistance of FAPESP (under grant 98/03206-7) during the development of the present research.
NOMENCLATURE
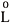
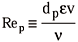
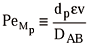
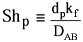
- Athalye, A.M; S.J. Gibbs and E.N. Lightfoot, Predictability of Chromatographic Protein Separations. Study of Size-exclusion Media with Narrow Particle Size Distributions, J. Chromatogr., 589, 71-85 (1992).
- Berninger, J.A.; R.D. Whitley; X. Zhang and L. N.-H Wang, A Versatile Model for Simulation of Reaction and Nonequilibrium Dynamics in Multicomponent Fixed-Bed Adsorption Processes, Comput. Chem. Eng., 15, 749-768 (1991).
- Chung, S.F. and C.Y. Wen, Longitudinal Dispersion of Liquid Flowing Through Fixed and Fluidized Beds. AIChE J, 14, 857-866 (1968).
- Cremasco, M.A., Fundamentals of Mass Transfer (in Portuguese). Unicamp Press, Campinas, Brazil, 741p. (1998).
- Dechow, F.J., Separation and Purification Techniques in Biotechnology. Noyes Publication, NJ (1989).
- Ernest, M.V. Jr., R.D. Whitley, Z. Ma, and L. N.-H. Wang, Effect of Mass Action Equilibria on Fixed Bed Multicomponent Ion Exchange Dynamics, Ind. Eng. Chem. Res., 36, 212-226 (1997).
- Fruton, J.F. and S. Simmonds, General Biochemistry, 2nd Edition, John Wiley & Sons, New York (1963).
- Gunn, D.J., Axial and Radial Dispersion in Fixed Beds, Chem. Eng. Sci., 42, 363-373 (1987).
- Hashimoto, K.; M. Yamada; S. Adachi and Y. A. Shirai, Simulated Moving-Bed Adsorber with Three Zones for Continuous Separation of L-Phenylalanine and NaCl, J. Chem. Eng. Japan, 22, 432-434 (1989).
- Heynes, H.W. Jr., The Determination of Effective Diffusivity by Gas Chromatography. Time Domain Solution. Chem. Eng. Sci., 30, 955-961 (1975).
- Ho, C.; C.B. Ching and D.M. Ruthven, A Comparative Study of Zeolite and Resin Adsorbents for the Separation of Fructose-Glucose Mixtures. Ind. Eng. Chem. Res., 26, 1407-1412 (1987).
- Jin, X.; Z. Ma; J. Talbot and L. N-H. Wang, A Model for Adsorption Equilibria of Solutes with Multiple Adsorption Orientation. Langmuir, 15 (9), 3321-3333 (1999).
- Koch, D.L. and J. F. Brady, Dispersion in Fixed Beds, J. Fluid Mech., 154, 399-427 (1985).
- Koh, J-H.; P.C. Wankat and L. N.-H. Wang, Pore and Surface Diffusion and Bulk-Phase Mass Transfer in Packed and Fluidized Beds. Ind. Eng. Chem. Res., 37, 228-239 (1998).
- Kumar, R.; R.C. Duncan and D.M. Ruthven, A Chromatography Study of Diffusion of Single Components and Binary Mixtures of Gases in 4A and 5A Zeolites. The Canadian J. of Chem. Eng., 60, 493-499 (1982).
- Ma, Z.; R.D Whitley and L. N.-H. Wang, Pore and Surface Diffusion in Multicomponent Adsorption and Liquid Chromatography Systems. AIChE J., 42 (5), 1244-1262(1996).
- Mackie, J.S. and P. Meares, The Diffusion of Electrolytes in a Cation-Exchange Resin Membrane. Proc. Roy. Soc. London, Ser. A, 267, 498-506 (1955).
- McGilvery, R.W., Biochemistry, a Functional Approach, Saunders Co, Philadelphia (1970).
- Miyabe, K. and M. Suzuki, Chromatography of Liquid-Phase Adsorption on Octadecylsilyl-Silica Gel. AIChE J., 38 (6), 901-909 (1992).
- Myers, A.L. and S. Byington, Thermodynamics of Ion Exchange: Prediction of Multicomponent Equilibria from Binary Data, in A.E. Rodrigues (ed.), Ion Exchange Science and Technology, NATO ASI Series, Serie E., No 107, Martinus Nijhoff Publ., Dordrecht, 1986.
- Paduano, L.; R. Sartoric; V. Vitagliano and L. Constantino, Transport and Thermodynamics Properties of (D.L) Norleucine-Water and (L) Phenylalanine-Water, at 25oC. J. Mol. Liq., 47, 193-202 (1990).
- Ruthven, D.M., Principles of Adsorption and Adsorption Processes. Wiley-Interscience, New York, 1984.
- Schneider, P. and J. Smith, Adsorption Rate Constant from Chromatography. AIChE J., 14, 762-771 (1968).
- Suzuki, M. and J.M.Smith, Kinetic Study by Chromatography. Chem. Eng. Sci., 26, 221-235 (1971).
- Whitley, R.D., Dynamics of Nonlinear Multicomponent Chromatography-Interplay of Mass Transfer, Intrinsic Sorption Kinetics, and Reaction, Ph.D. diss., Purdue University, West Lafayette, U.S.A (1990).
- Wilson, E.J. and C.J. Geankoplis, Liquid Mass Transfer at Very Low Reynolds Numbers in Packed Beds. Ind. Eng. Chem. Fundam., 5, 9 (1966).
- Wooley, R.; Z. Ma and L. N.-H. Wang, A Nine Zone Simulating Moving Bed for the Recovery of Glucose and Xylose from Biomass Hydrolyzate. Ind. Eng. Chem. Res., 37, 3699-3709 (1998).
- Wu, D-J.; Y. Xie; Z. Ma and L. N.-H. Wang, Design of Simulated Moving Bed Chromatography for Amino Acid Separations, Ind. Eng. Chem. Res., 37, 4023-4035 (1998).
Publication Dates
-
Publication in this collection
30 July 2001 -
Date of issue
June 2001
History
-
Accepted
13 June 2001 -
Received
02 Jan 2001



































