Abstract
The arbitrary angular momentum solutions of the Schrödinger equation for a diatomic molecule with the general exponential screened coulomb potential of the form V(r) = (- a / r){1+ (1+ b )e-2b } has been presented. The energy eigenvalues and the corresponding eigenfunctions are calculated analytically by the use of Nikiforov-Uvarov (NU) method which is related to the solutions in terms of Jacobi polynomials. The bounded state eigenvalues are calculated numerically for the 1s state of N2 CO and NO
Nikiforov-Uvarov method; Eigenvalues; Eigenfunctions; General Exponential Screened Coulomb Potential
ARTICLE
Bound state solutions of schrödinger equation for a more general exponential screened coulomb potential via Nikiforov-Uvarov method
Benedict I. ItaI; P. EkuriI; Idongesit O. IsaacII; Abosede O. JamesIII
IQuantum Chemistry Group, Department of Pure and Applied Chemistry, University of Calabar, P. O. Box 3700, Calabar, CRS, Nigeria
IIDepartment of Mathematics/Statistics and Computer Science, University of Calabar, Calabar, Cross River State, Nigeria
IIIDepartment of Pure and Industrial Chemistry, University of Port Harcourt, Nigeria
ABSTRACT
The arbitrary angular momentum solutions of the Schrödinger equation for a diatomic molecule with the general exponential screened coulomb potential of the form V(r) = (- a / r){1+ (1+ b )e-2b } has been presented. The energy eigenvalues and the corresponding eigenfunctions are calculated analytically by the use of Nikiforov-Uvarov (NU) method which is related to the solutions in terms of Jacobi polynomials. The bounded state eigenvalues are calculated numerically for the 1s state of N2 CO and NO
Keywords: Nikiforov-Uvarov method, Eigenvalues, Eigenfunctions, General Exponential Screened Coulomb Potential.
Introduction
The exact analytic solutions of the wave equations (non-relativistic and relativistic) are only possible for certain potentials of physical interest under consideration since they contain all the necessary information on the quantum system [1]. It is known that for certain potentials, the Schrödinger equation can be solved for the angular momentum quantum numbers  =0 [2]. However, in some cases, like for the
=0 [2]. However, in some cases, like for the  ≠ 0 states, some approximations are often used to obtain analytic solutions of the Schrödinger equation [3 - 5].
≠ 0 states, some approximations are often used to obtain analytic solutions of the Schrödinger equation [3 - 5].
A more general exponential screened coulomb (MGESC) potential used in this paper is of the form [6]:
where a is the strength coupling constant and b is the screened parameter. The potential in equation (1) is known to describe adequately the effective interaction in many-body environments of a variety of fields [6]. In this paper, we have decided to explore the possibility of also using it in obtaining bound state solutions of the Schrödinger equation for diatomics using Nikiforov-Uvarov (NU) method.
Overview of Nikiforov-Uvarov (NU) Method
The NU method is based on the solutions of general second order linear differential equations with some orthogonal functions [7]. For the given potential, the Schrödinger equation in the spherical coordinates is reduced to a generalized equation of hypergeometric type with an appropriate s =s(r) coordinate transformation. Thus, it has the form [8]:
where σ(s) and  (s) are polynomials, at most , second-degree, and
(s) are polynomials, at most , second-degree, and  (s) is a first-degree polynomial. To find a particular solution of equation (2), we use the following transformation [9]:
(s) is a first-degree polynomial. To find a particular solution of equation (2), we use the following transformation [9]:
This reduces Schrödinger equation (2) to an equation of hyper-geometric type:
where Φ(s) satisfies
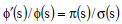 y(s) is the hyper-geometric type function whose polynomial solutions are given by the Rodrigues relation:
y(s) is the hyper-geometric type function whose polynomial solutions are given by the Rodrigues relation:
where Bn is a normalization constant and the weight function ρ must satisfy the condition [9]:
The function π and the parameter λ required for this method are defined as:
and
Here, π(s) is a polynomial with the parameter s and the determination of k is necessary for π(s) to be obtained. To find k, the expression under the square root must be square of a polynomial. A new eigenvalue equation for the Schrödinger equation thus becomes:
where
and τ'(s) must be negative.
Bound State Solutions via Nikiforov-Uvarov (NU) Method
The potential in equation (1) is substituted into the radial Schrödinger equation given as:
where n denotes the radial quantum number which together with  are both named as the vibration-rotation quantum numbers in molecular chemistry, r is the internuclear separation, En
are both named as the vibration-rotation quantum numbers in molecular chemistry, r is the internuclear separation, En is the exact bound state energy eigenvalues and V(r) is the internuclear potential energy function and we obtain:
is the exact bound state energy eigenvalues and V(r) is the internuclear potential energy function and we obtain:
Equation (12) can be rearranged to give:
Introducing the following dimensional parameters:
equation (13) is written as:
A comparison of equations (2) and (17) reveals the following polynomials:
Substituting these polynomials into equation (7), we get π(r) as:
and σ'(r) is taken equal to 1. The discriminant of the expression under the square root in equation (19) has to be zero for it to have equal roots. Therefore, we get:
On solving equation (20) for k we get:
where
and
Substituting k± into equation (19), gives the following four possible solutions obtained for π(r) as:
From the four possible forms of π(r) in equation (24), we select the one for which the function τ(s) in equation (10) has a negative derivative. τ(s) satisfies these requirements with:
and
From equation (8), we obtain:
and also
We then obtain the parameters ε2 as:
Substituting the values of ε2 , β and γ from equations (14) - (16) into equation (29), yields:
To find y(r), we first obtain ρ(r) from equation (6) as:
Substituting this into the Rodrigues relation given in equation (5), we get:
Bn is the normalization constant. The polynomial solutions of yn
is the normalization constant. The polynomial solutions of yn (r) in equation (32) are expressed in terms of the associated Laguerre polynomials, which is one of the orthogonal polynomials. We write:
(r) in equation (32) are expressed in terms of the associated Laguerre polynomials, which is one of the orthogonal polynomials. We write:
where v= 2iεr, therefore,
By substituting π(r) and σ(r) into the expression  and solving the resulting differential equation, the other part of the wave function in equation (3) is obtained as:
and solving the resulting differential equation, the other part of the wave function in equation (3) is obtained as:
or in terms of v,
Combining the Laguerre polynomials and φ(v) in equation (3), enables the radial wave function to be constructed as:
If we introduce the variable
To find An , a new normalization constant, we write:
, a new normalization constant, we write:
Therefore,
The above integral can be evaluated by using the recursion relation for Laguerre polynomials and An is found to be:
is found to be:
Therefore, Rn (r) becomes:
(r) becomes:
Conclusion
The analytical solutions of the Schrödinger equation for the general exponential screened coulomb potential has been presented. The Nikiforov-Uvarov method employed in the solutions enables us to explore an effective way of obtaining the eigenvalues and corresponding eigenfunctions of the Schrödinger equation for any  - state.
- state.
Finally, we calculate the energies of the exponential screened coulomb potential for diatomic molecules by means of equation (30) for the  - state. The explicit values of the energy at different values of the screened parameter are shown in Table 1.
- state. The explicit values of the energy at different values of the screened parameter are shown in Table 1.
Corresponding author: e-mail: iserom 2001@yahoo.com
References
- [1] S. M. Ikhdair and R. Sever (2008). Improved analytical approximation to arbitrary - state solutions of the Schrödinger equation for the hyperbolical potentials. Personal Communication.
- [2] C. Berkdemir and J. Han (2005). Chem. Phys. Lett. 409, 203 - 207.
- [3] E. Aydmer and C. Orta (2008). Quantum information entropies of the eigenstates of the eigenvalues of the Morse potential. Personal Communication.
- [4] H. Taseli (1997). Int. J. Quantum Chemistry, 63(5), 949 - 959.
- [5] M. W. Kermode, M. L. J. Allen, J. P. McTavish and A. Kervell (1984). J. Phys. G: Nucl. Phys. 773 - 783.
- [6] S. M. Ikhdair and R. Sever (2008). Bound states of a more general exponential screened coulomb potential. Personal Communication.
- [7] A. F. Nikiforov and U. B. Uvarov (1988). Special Functions of Mathematical Physics, Birkhauser: Basel.
- [8] C. Tezcan and R. Sever (2008). Quantum Physics. 15, 1 - 20.
- [9] S. Ikhdair and R. Sever (2008). Cent. Eur. J. Physics. 6, 141 - 152.
Publication Dates
-
Publication in this collection
09 Dec 2010 -
Date of issue
Sept 2010

 Bound state solutions of schrödinger equation for a more general exponential screened coulomb potential via Nikiforov-Uvarov method
Bound state solutions of schrödinger equation for a more general exponential screened coulomb potential via Nikiforov-Uvarov method





































 equation (38) becomes:
equation (38) becomes:





