Abstract
Working within the domain of inviscid incompressible MHD theory, we found that a tangential discontinuity (TD) separating two uniform regions of different density, velocity and magnetic field may be Kelvin-Helmholtz (KH) stable and yet a study of a transition between the same constant regions given by a continuous velocity profile shows the presence of the instability with significant growth rates. Since the cause of the instability stems from the velocity gradient, and since a TD may be considered as the ultimate limit of such gradient, the statement comes as a surprise. In fact, a long wavelength (lambda) boundary for the KH instability does not exist in ordinary liquids being instead a consequence of the presence of magnetic shear, a possibility that has passed unnoticed in the literature. It is shown that KH modes of a magnetic field configuration with constant direction do not have the long lambda boundary. A theoretical explanation of this feature and examples of the violation of the TD stability condition are given using a model that can be solved in closed form. Stability diagrams in the (kd, MA) plane are given (where kd = 2pid/lambda, 2d is the velocity gradient length scale, and MA is the Alfvénic Mach number) that show both the well-known limit at small lambdas and the boundary for large but finite lambdas noted here. Consequences of this issue are relevant for stability studies of the dayside magnetopause as the stability condition for a TD should be used with care in data analysis work.
On the MHD boundary of Kelvin-Helmholtz stability diagram at large wavelengths
F. T. GrattonI; G. GnaviI; C. J. FarrugiaII; L. BenderI
IInstituto de Física del Plasma, Facultad de Ciencias Exactas y Naturales, Universidad de Buenos Aires y Consejo Nacional de Investigaciones Científicas y Técnicas, Ciudad Universitaria, 1428 Buenos Aires, Argentina
IISpace Science Center, University of New Hampshire, Durham, NH, 03824, USA
ABSTRACT
Working within the domain of inviscid incompressible MHD theory, we found that a tangential discontinuity (TD) separating two uniform regions of different density, velocity and magnetic field may be Kelvin-Helmholtz (KH) stable and yet a study of a transition between the same constant regions given by a continuous velocity profile shows the presence of the instability with significant growth rates. Since the cause of the instability stems from the velocity gradient, and since a TD may be considered as the ultimate limit of such gradient, the statement comes as a surprise. In fact, a long wavelength (l) boundary for the KH instability does not exist in ordinary liquids being instead a consequence of the presence of magnetic shear, a possibility that has passed unnoticed in the literature. It is shown that KH modes of a magnetic field configuration with constant direction do not have the long l boundary. A theoretical explanation of this feature and examples of the violation of the TD stability condition are given using a model that can be solved in closed form. Stability diagrams in the (kd, MA) plane are given (where kd = 2pd/l, 2d is the velocity gradient length scale, and MA is the Alfvénic Mach number) that show both the well-known limit at small ls and the boundary for large but finite ls noted here. Consequences of this issue are relevant for stability studies of the dayside magnetopause as the stability condition for a TD should be used with care in data analysis work.
1 Introduction
The importance of the Kelvin-Helmholtz (KH) instability of parallel flows in laboratory, geophysical, or astrophysical systems, recognized many years ago, has generated a huge literature. An internet search covering only the year 2003 yielded over 280 entries. Theoretical works on the KH instability are numerous. For overviews, among many others see Chandrasekhar [1], Drazin and Reid [2], Belmont and Chanteur [3], Huerre and Rossi [4], Farrugia, Gratton and Torbert [5], and the references quoted in these surveys.
Working within the domain of inviscid incompressible MHD theory, we find that a tangential discontinuity between two uniform regions of different density, velocity and magnetic field may be Kelvin-Helmholtz stable, and yet a smooth transition (modeled with continuous profiles) between the same constant regions shows the existence of unstable modes (with equal orientation of the  -vector) with significant growth rates. Since the cause of the instability stems from the velocity gradient, and since a tangential discontinuity (TD) may be considered as the ultimate limit of such gradient, the statement may be surprising at first sight.
-vector) with significant growth rates. Since the cause of the instability stems from the velocity gradient, and since a tangential discontinuity (TD) may be considered as the ultimate limit of such gradient, the statement may be surprising at first sight.
The assumption that a TD is the worst case from the point of view of stability may lead to the erroneous inference that to decide on the stability of a particular parallel flow it is sufficient to examine the criterion of the related TD (also called the thin model). The tangential discontinuity is a simple planar interface across which the unperturbed fields, velocity  , magnetic strength,
, magnetic strength,  , and density r0, suffer sudden changes. According to incompressible MHD theory, a TD is Kelvin-Helmholtz stable when the following relation is satisfied:
, and density r0, suffer sudden changes. According to incompressible MHD theory, a TD is Kelvin-Helmholtz stable when the following relation is satisfied:
Here the indices 1 and 2 refer to quantities on either side of the interface, and Vk, Bk are the projections of  and
and  on the direction of the wave vector
on the direction of the wave vector  , respectively. The criterion depends on the direction of
, respectively. The criterion depends on the direction of  but not on its absolute value, k = |
but not on its absolute value, k = | | , and hence it is valid for all wavelengths, l = 2p/k associated to modes with a fixed
| , and hence it is valid for all wavelengths, l = 2p/k associated to modes with a fixed  orientation.
orientation.
The assumption that the stability of a parallel flow is ensured by the stability criterium of the associated TD does not hold in general for MHD. In a magnetohydrodynamic flow, and for a fixed orientation of the  -vector, besides the well-known short wavelength stability boundary ls (such that modes with l < ls are stable) there are configurations in which also a long wavelength limit ll exists (such that all modes with l > ll are KH stable). In other words, the unstable l interval may be finite, ls< l < ll, instead of semi-infinite, ls< l < ¥, as it is widely assumed.
-vector, besides the well-known short wavelength stability boundary ls (such that modes with l < ls are stable) there are configurations in which also a long wavelength limit ll exists (such that all modes with l > ll are KH stable). In other words, the unstable l interval may be finite, ls< l < ll, instead of semi-infinite, ls< l < ¥, as it is widely assumed.
The long wavelength (l) stability boundary is a consequence of the presence of magnetic shear in MHD flows, a possibility that seems to have been passed unnoticed in the literature. Indeed, a long l boundary for the KH instability does not exist in ordinary non conducting fluids, where there is no process equivalent to the magnetic field stabilization. The surface tension operates only at the interface of two liquids, and it is not similar to the action of a magnetic field, the latter being an effect operating in the whole volume of the flow.
In this paper we give examples and a theoretical explanation of the violation of the stability condition 1 at moderate ls, using a model that can be solved in closed form in which the fields have idealized profiles. We present stability diagrams in the (kd, MA) plane (where kd = 2pd/l, 2d = D is the scale length of the velocity gradient, and MA is the Alfvénic Mach number) which shows both the well-known limit at small ls and the new boundary for large, but finite l values. We also show, by solving numerically the stability problem of a similar model built with smooth (hyperbolic tangent) profiles, that a MHD flow with a uniformly oriented magnetic field does not have the long l stability boundary.
Among the possible applications of this feature of the KH instability perhaps one of the most important is found in space physics, related to the data analysis of spacecraft crossings of the terrestrial magnetopause A study of the KH instability of configurations that become stable at large ls due to the presence of magnetic shear, relevant to magnetospheric physics has been published elsewhere Gratton et al. [6]. Here we present a different set of theoretical results of general nature, not aimed at a particular application.
2 The basic equation of the MHD Kelvin-Helmholtz instability for incompressible flows
Our study of the Kelvin-Helmholtz instability is based on the perturbative linear equation derived from the set of ideal MHD equations (see, e.g., reference [1])
Given a set of Cartesian axes, (x, y, z), such that the main stream is lying on (x, z) planes, all equilibrium fields  ,
,  , r0 are stratified across the flow and depend on y only,
, r0 are stratified across the flow and depend on y only,
The y component of the amplitude of a Lagrangian displacement of an infinitesimal plasma element is denoted by  =
=  (x, y, z, t). The displacement
(x, y, z, t). The displacement  is related to the y component of the perturbative velocity by
is related to the y component of the perturbative velocity by  = d
= d /dt . The dependence of the modes is assumed to be of the form
/dt . The dependence of the modes is assumed to be of the form
The real wavevector  = (kx, 0, kz) lies on the (x, z) plane, the coordinate y is in the direction transversal to the motion, and k =
= (kx, 0, kz) lies on the (x, z) plane, the coordinate y is in the direction transversal to the motion, and k =  , the absolute value of
, the absolute value of  , defines the wavelength of the mode.
, defines the wavelength of the mode.
A derivation of the equation that governs the mode amplitude z(y) (omitted here for brevity) can be found, for instance, in Farrugia et al. [7]. The equation is
and is obtained under the condition div( ) = 0 that corresponds to the incompressible approximation. Given
) = 0 that corresponds to the incompressible approximation. Given  , the characteristic boundary value problem for equation 5 determines both the complex frequency w and the mode eigenfunction z(y). The imaginary part of w, g = Á(w), gives the growth rate of the instability (when g > 0) and the real part, Â(w), gives the frequency of oscillation of the wave.
, the characteristic boundary value problem for equation 5 determines both the complex frequency w and the mode eigenfunction z(y). The imaginary part of w, g = Á(w), gives the growth rate of the instability (when g > 0) and the real part, Â(w), gives the frequency of oscillation of the wave.
The function 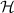 =
= 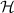 (y;
(y;  , w) in eq.5 is defined by
, w) in eq.5 is defined by
where c is the (complex) phase velocity
In eq.6 , as already mentioned in the introduction, r0 = r0(y) represents the equilibrium density across the flow, Vk(y) =  ·
·  is the projection of the equilibrium velocity
is the projection of the equilibrium velocity  =
=  (y) on the wavevector direction
(y) on the wavevector direction  =
=  /k, while Bk = Bk(y) =
/k, while Bk = Bk(y) =  ·
·  is the projection of the equilibrium magnetic field. Finally, we have introduced the projection of the Alfvén velocity on
is the projection of the equilibrium magnetic field. Finally, we have introduced the projection of the Alfvén velocity on  , VAk, defined by
, VAk, defined by
The reader must note that equation 5 is valid for modes with all ls, but with a fixed  . A change of the wavevector direction leads to a different set of field projections, Vk(y), Bk(y) and so to a different eq. 5 . Thus, a complete stability analysis of a given parallel flow, requires not only a study of all ls modes, but also an examination of all the orientations of
. A change of the wavevector direction leads to a different set of field projections, Vk(y), Bk(y) and so to a different eq. 5 . Thus, a complete stability analysis of a given parallel flow, requires not only a study of all ls modes, but also an examination of all the orientations of  on the (x, z) plane. In the following we ordinarily consider only fixed
on the (x, z) plane. In the following we ordinarily consider only fixed  modes, and therefore the analysis is restricted to a particular direction of the wavevector.
modes, and therefore the analysis is restricted to a particular direction of the wavevector.
The theory based on eq.5 is restricted to incompressible perturbations. It can be shown (e.g., Contin et al. [8], Gratton et al. [9]) that the relative change of density due to plasma advection, (dr/r0)a, for KH modes, is much larger than the variation due to compressibility, (dr/r0)c, when the velocity U = | | is much smaller than the local speed of sound, Cs. In fact, it turns out that one can estimate the ratio of density changes caused by the two mentioned processes as
| is much smaller than the local speed of sound, Cs. In fact, it turns out that one can estimate the ratio of density changes caused by the two mentioned processes as
Thus, compressibility is a minor correction in the perturbative analysis of subsonic flows when
We may note that in y intervals where 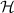 is constant there are two simple exponential solutions exp(±ky) of eq. 5 . These solutions represent the KH perturbation on both sides of a layer that separates two regions of constant field with different values. When the wavelength l becomes much larger than the thickness D of the interface, the field profiles r0, Bk, or Vk can be approximated by step functions with a discontinuity (TD) at the position y = y0 where the transition occurs. Thus, when l >> D, the KH modes do not contain any information on the structure of the transition, and are forced to be piecewise exponential functions, symmetric with respect to y0. Qualitatively speaking, the long-l modes have lost functional "plasticity" compared with solutions corresponding to l ~ D.
is constant there are two simple exponential solutions exp(±ky) of eq. 5 . These solutions represent the KH perturbation on both sides of a layer that separates two regions of constant field with different values. When the wavelength l becomes much larger than the thickness D of the interface, the field profiles r0, Bk, or Vk can be approximated by step functions with a discontinuity (TD) at the position y = y0 where the transition occurs. Thus, when l >> D, the KH modes do not contain any information on the structure of the transition, and are forced to be piecewise exponential functions, symmetric with respect to y0. Qualitatively speaking, the long-l modes have lost functional "plasticity" compared with solutions corresponding to l ~ D.
From eq.5 one can see that the matching conditions at y = y0 are
where the bracket is a shorthand for [Q] = limή0[Q(y0 + Î) -Q(y0 - Î)] . This is the basis of the TD model, which leads to the dispersion relation
where the suffixes 1 and 2 indicate the values of the physical quantities on either side of the discontinuity.
3 The unstable spectra and the mode weight analogy
The square of the absolute value of the Lagrangian displacement
and the square of the absolute value of the derivative of z play an important role in the determination of the spectrum properties of the characteristic values c = cr + ici. In fact, to discuss the spectral properties of the KH instability we may introduce a quantity analogous to a density distribution, which we may call the mode weight, defined by
In this conceptual analogy M(y) is associated not only to the equilibrium density r0(y) but depends also on the amplitude and the localization of the perturbative mode via the bracket containing |z| 2 and |dz/dy| 2. The mode weight is reminiscent of techniques used in the Rayleigh-Ritz method for the study of the Sturm-Liouville spectral theory, although the characteristic value dealt here is not an eigenvalue problem of the latter family, and therefore some powerful classic properties do not hold.
Starting from equation 5 , multiplying by z* and integrating over y, we find after integration by parts the following condition
We have assumed that z ® 0 as y ® ±¥, which is certainly the case for configurations with two asymptotic regions with constant fields, separated by a velocity gradient region where all the fields change. In mathematical terms, the formula 15 is of the class of "energy integrals".
Separating the real and the imaginary part of equation 15 , it is easy to show that the real and imaginary part of the phase velocity, c = cr + ici, for the unstable spectrum ci º Á(c) > 0, are given by the expressions indicated in the following. The real part of the phase velocity is equal to the speed of a fictitious center of mass
computed with the mode weight. Clearly, the weight distribution decides which value of the Vk(y) profile is being emphasized by a physically admissible perturbation, and becomes the phase velocity of the growing wave. The importance of equation 16 is that it shows that the phase velocity is restricted to the range Vkm < cr < VkM, where Vkm, VkM are the minimum and maximum values of Vk(y), respectively, and thus the excited wave is resonant with some plasma layer of the velocity gradient region. We must stress that this holds only when ci ¹ 0.
The growth rate of the instability, instead, is given by the difference of two positive terms. The first, the driving term of the instability, is the quadratic spread of the velocity projection Vk(y) with respect to the center of mass velocity. The second term represents the stabilizing influence of magnetic tensions, and is equal to the quadratic mean of the projected Alfvén velocity
The formula reminds at a distance a similar Rayleigh quotient of the mathematical literature of spectral problems.
It is convenient, starting from the mode weight M(y), to define also a normalized mode distribution
satisfying
We can then rely on the analogy of f(y) with probability functions, also positive definite, and normalized to unity. Thus, we may define the average of any physical quantity Q(y) with respect to the mode distribution as,
in the customary way averages are defined in the theory of probability.
The spectral theorem, embodied in equations 16 and 17 , can then be rewritten in the following form
using the first and second order moments of the mode distribution f(y). We noted already the meaning of the first equation, when the average áVkñ has been determined by a mode distribution, the growth of the instability given by the second equation 21 depends on the quadratic deviation of Vk(y) with respect to the mean value, but is reduced by the average of  . The first term in
. The first term in  relies on the presence of a region of velocity gradient for Vk(y) (i.e., vorticity, rot(
relies on the presence of a region of velocity gradient for Vk(y) (i.e., vorticity, rot( )) because it is zero when Vk is a constant. On the other hand, the stabilizing term is due to the magnetic tensions that arise from the perturbation z(y), which modifies the curvature of the magnetic lines that are straight in equilibrium.
)) because it is zero when Vk is a constant. On the other hand, the stabilizing term is due to the magnetic tensions that arise from the perturbation z(y), which modifies the curvature of the magnetic lines that are straight in equilibrium.
To favor the excitation of the instability, a perturbation should have a peak of f(y) at a y position that gives a significant spread to (Vk - áVkñ)2, while the same location and peak should produce only a small average  . It is evident that very strong magnetic fields may not permit the existence of such a perturbation. However, in general, because of the difference of the profile functions Vk(y) and VAk(y), it may be possible to have y intervals where the former dominates over the latter. In that case, when the mode is physically admissible, a peak of f(y) in that specific interval may emphasize the quadratic spread of Vk- áVkñ over the average
. It is evident that very strong magnetic fields may not permit the existence of such a perturbation. However, in general, because of the difference of the profile functions Vk(y) and VAk(y), it may be possible to have y intervals where the former dominates over the latter. In that case, when the mode is physically admissible, a peak of f(y) in that specific interval may emphasize the quadratic spread of Vk- áVkñ over the average  , and produce the amplification of the perturbation.
, and produce the amplification of the perturbation.
As an example of application of the spectral eqs.21 , we show how the dispersion relation for a TD can be derived from this theorem. The TD is defined by two sets of parameters,  , and r1,2 for regions 1, y > 0, and 2, y < 0, separated by an interface at y = 0, where the physical quantities suffer jumps. The solution of eq.5 for this case, taking into account the continuity of z at y = 0, is
, and r1,2 for regions 1, y > 0, and 2, y < 0, separated by an interface at y = 0, where the physical quantities suffer jumps. The solution of eq.5 for this case, taking into account the continuity of z at y = 0, is
so that the mode function f(y) for this case is given by
Averaging Vk with 23 we immediately obtain the phase velocity of the modes,
provided ci ¹ 0 .Computing  with eq.21 we find
with eq.21 we find
completing an alternative deduction of the well-known dispersion relation for a TD. The stability criterium 1 is a direct consequence of eq.25 .
In section 5 we use the mode distribution function and the spectral theorem to explain the existence of the long wavelength boundary of the KH instability.
4 A stability analysis solved in closed form
In this model a sharp density drop and a variation of Bk occur over a distance d, much smaller than the thickness, D = 2d, where the gradient of the velocity Vk occurs. When there is a wide difference between the two scales, d << D, we can obtain an exact solution of eq. 5 assuming step functions for r0 , Bk, and a linear velocity profile for Vk in the interval -d < y < d. The model ignores the field structure within d, which is possible when the wavelength of the perturbation is such that the condition d << l holds. Thus, this model represents the changes of r0 and Bk with discontinuous jumps through an interface of zero thickness (d ® 0) located at y = -d, where matching conditions like eq.11 apply.
The schematic for this model is given in Fig. 1, which shows step functions for both r0 and Bk, while Vk is represented by a continuous, albeit broken profile. The latter has a linear transition of width 2d between two constant velocity values, the one on the left chosen to be zero. In Fig. 1 the horizontal y axis is normalized with d, but the vertical scale is only qualitative, and indicates the trend of the three quantities, r0, Bk, and Vk. The reader should note that the configuration does not require  to be zero in the interval -d < y < ¥, but only that the magnetic field
to be zero in the interval -d < y < ¥, but only that the magnetic field  is unidirectional there. We are considering modes with a
is unidirectional there. We are considering modes with a  -vector normal to the magnetic field (
-vector normal to the magnetic field ( ^
^  ) in that region. At y = -d a current sheet exists and the direction of
) in that region. At y = -d a current sheet exists and the direction of  rotates sharply, so that Bk¹ 0 when -¥ < y < -d, an interval in which the magnetic field becomes unidirectional again but with a different orientation.
rotates sharply, so that Bk¹ 0 when -¥ < y < -d, an interval in which the magnetic field becomes unidirectional again but with a different orientation.
The purpose of this model is only to prove with an exact solution, easy to handle and discuss, the thesis of the paper. It does not intend to represent realistic features for any particular application.
With the change of variable
eq.5 is transformed into
We note that, since Bk = 0 in the range of -d < y < ¥, then 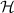 = r1(c-Vk(y))2, where r1 is constant there. Within the region of constant density and linear dependence of Vk with y (constant dVk/dy) we see that
= r1(c-Vk(y))2, where r1 is constant there. Within the region of constant density and linear dependence of Vk with y (constant dVk/dy) we see that
Then, the solution of eq.5 for the slab -d < y < d is given by
where C1,2 are constants, and
where we have written U1 = Vk(d) and set Vk(-d) = 0.
For the interval 1, d < y, the solution can be written as
whereas in the interval 2, y < -d, the solution is given by
with constant density r2 in that region
The dispersion relation is derived from two sets of matching conditions: one set is for y = -d,
where there are jumps of r0 and Bk, so that, 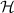 s = r1c2, for the value of
s = r1c2, for the value of 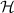 on the slab side, and
on the slab side, and 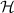 2 = r2c2 -
2 = r2c2 - 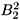 /4p for the opposite side. The quantity B2 = Bk(-d) is the constant value of the magnetic field projection on
/4p for the opposite side. The quantity B2 = Bk(-d) is the constant value of the magnetic field projection on  in region 2. The other boundary conditions are at y = d
in region 2. The other boundary conditions are at y = d
where all the fields are continuous (and Bk = 0). There are four coefficients, A1,2 and C1,2, in the solution and four boundary conditions, which are linear homogeneous equations for these coefficients. The compatibility condition for this set of equations requires a 4×4 determinant to be zero, which leads to an equation for w, as is customary in this type of problems.
The algebra is simple although laborious, omitting details the resulting polynomial in  can be written as
can be written as
which gives the dispersion relation for the model with a linear velocity slab and discontinuities in density and magnetic field. The meaning of the symbols in eq.34 is the following
The nondimensional parameter P is the inverse of a particular type of Alfvénic Mach number, MA, defined with the velocity projection U1 and density r1 of region 1, and the magnetic field projection of region 2.
In eq.34 l and D = 2d may take arbitrary values. The limit d ® 0 when a ® 0, requires the expansion of eq.34 in a power series of a. The lower orders a0, a1, are satisfied identically. The lowest significant order is a2, and leads to
which is the same polynomial that can be derived from eq.12 when
1 = r1(c - U1)2 and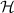 2 = r2c2 -
2 = r2c2 - 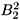 /4p. In this limit the slab -d < y < d reduces to a TD, so that both eq.25 and the roots of eq.36 in this case give
/4p. In this limit the slab -d < y < d reduces to a TD, so that both eq.25 and the roots of eq.36 in this case give
Thus, the condition for KH stability in the long wavelength limit of the model of Fig. 1 is
which corresponds to the condition
Next, we assume that condition 38 is satisfied, and holding the same parameters for regions 1 and 2, i.e., for equal MA and rd, we analyze the dispersion relation for finite D = 2d as a function of a = 2kd. Thus, we proceed to compute numerically the roots of eq. 34 , which are shown in Fig. 2. As an example, for a density ratio rd = r2/r1 = 0.1, and P = 0.43 that corresponds to MA = 2.3, P is about 1.44 times larger than the critical stability value for the corresponding TD, Pc = 0.3015, so that condition 1 is satisfied. Nevertheless, as we can see from Fig. 2 the system is unstable with a maximum growth rate gm » 0.16U1/D at about kd = 0.7. In the range kd  0.22, which is equivalent to l > ll
0.22, which is equivalent to l > ll  14.3 × D, all the modes (with the same orientation
14.3 × D, all the modes (with the same orientation  ) are stable, so that the result is consistent with the TD criterium that predicts stability at very long ls.
) are stable, so that the result is consistent with the TD criterium that predicts stability at very long ls.
In Fig. 2, the growth rate is normalized with U1/d, and the plot shows 5 × Á(wd/U1) versus kd, so that the real part of w may be shown in the same diagram. On the same vertical scale, the normalized phase velocity Â(w)/kU1 is given too. The figure gives the roots of (34) for rd = 0.1, and seven values of MA = (1.3, 1.4, 1.5, 1.7, 1.9, 2.3, 4). The upper line segments of the plot give the phase velocity of the modes for which 5 × gd/U1 is represented by the curves in the lower part. The maximum growth rate of these curves increases steadily with increasing MA. We may also note that the curves for 5 × Á(wd/U1) are shifted from right to left as MA increases, while the unstable kd intervals also grow systematically. Finally, between MA = 2.3 and MA = 4 , more precisely for  = 3.317, the long wavelength boundary disappears, in coincidence with the fact that the TD becomes unstable. For MA >
= 3.317, the long wavelength boundary disappears, in coincidence with the fact that the TD becomes unstable. For MA >  both the TD and the model with a finite thickness are unstable, however the growth rates computed with eq.25 are reasonable estimates only when l >> D, otherwise they are unreliable.
both the TD and the model with a finite thickness are unstable, however the growth rates computed with eq.25 are reasonable estimates only when l >> D, otherwise they are unreliable.
The phase velocity of the unstable modes is nearly constant at the smaller values of MA, but shows more variation when MA is large, with Â(w)/k as a decreasing function of k, so that the group velocity is smaller than the phase velocity.
Of course, the results and comments of this section are for a particular choice of  , the study of a different orientation of the
, the study of a different orientation of the  -vector would need new computations for different functions Vk and Bk, as already noted.
-vector would need new computations for different functions Vk and Bk, as already noted.
5 The equivalent weight of unstable modes
We show now why it may be possible that the modes are stable at long ls, and yet be unstable at smaller wavelengths. Since the modes are known from the solutions of section 4 we can compute z in closed form. As an example, Fig. 3 shows the shape of Â(z), the real part of the y-component of the Lagrangian displacement (see eq.5) for a particular unstable mode of the model of section 4, corresponding to rd = 0.1, MA = 2.3, and kd = 0.6, with g = 0.156 U1/D. For the same  orientation, modes with very long ls are stable since MA <
orientation, modes with very long ls are stable since MA <  = 3.317. For kd = 0.6 we note that Â(z) has a neat peak near y ~ 0.5d in a range of y where Bk = 0. At y = 0.5d the value of Vk is 0.75, while the phase velocity, equal to < Vk > according to equation 21 , is cr = 0.7. The peak of Â(z) does not extend (with significant amplitude) into the y interval (y < -d) where the magnetic tension generated by Bk¹ 0 provides a stabilizing effect. The imaginary part, Á(z) (not shown) has similar features.
= 3.317. For kd = 0.6 we note that Â(z) has a neat peak near y ~ 0.5d in a range of y where Bk = 0. At y = 0.5d the value of Vk is 0.75, while the phase velocity, equal to < Vk > according to equation 21 , is cr = 0.7. The peak of Â(z) does not extend (with significant amplitude) into the y interval (y < -d) where the magnetic tension generated by Bk¹ 0 provides a stabilizing effect. The imaginary part, Á(z) (not shown) has similar features.
Figure 4 gives the corresponding mode distribution function f(y) computed from eq.14 . We can see that the normalized M(y) is strongly localized in a y interval where (Vk- < Vk >)2 is significantly different from zero, while  = 0, and that M(y) is very small in the range of y where
= 0, and that M(y) is very small in the range of y where  is different from zero. The peak of M(y) in Fig. 4 appears much enhanced with respect to that of Â(z) in Fig. 3. This is not only due to |z|2, but mainly to the contribution of |dz/dy|2.
is different from zero. The peak of M(y) in Fig. 4 appears much enhanced with respect to that of Â(z) in Fig. 3. This is not only due to |z|2, but mainly to the contribution of |dz/dy|2.
The possibility of such a strong localization of the mode distribution f(y) for moderate ls allows the inequality á(Vk - áVkñ)2ñ > á ñ to be satisfied, and hence the development of the instability, in agreement with eq.21 . Conversely, modes with very long wavelengths, such that l >> D, are broadly spread over and much beyond the interval D = 2d. In fact, at these ls, the solution z becomes necessarily close to exponential functions that decay symmetrically on both sides of the transition, as can be seen from equation 22 . Flexibility in localization is not possible at these long ls, the mode distribution has a fixed shape, a broad symmetric peak about y = 0, where the velocity gradient region is concentrated. This kind of mode distribution cannot avoid to give a significant contribution to the average of
ñ to be satisfied, and hence the development of the instability, in agreement with eq.21 . Conversely, modes with very long wavelengths, such that l >> D, are broadly spread over and much beyond the interval D = 2d. In fact, at these ls, the solution z becomes necessarily close to exponential functions that decay symmetrically on both sides of the transition, as can be seen from equation 22 . Flexibility in localization is not possible at these long ls, the mode distribution has a fixed shape, a broad symmetric peak about y = 0, where the velocity gradient region is concentrated. This kind of mode distribution cannot avoid to give a significant contribution to the average of  , arising from intervals where
, arising from intervals where  ¹ 0. Therefore, at long wavelengths the inequality may be reversed, á(Vk - áVkñ)2ñ < á
¹ 0. Therefore, at long wavelengths the inequality may be reversed, á(Vk - áVkñ)2ñ < á ñ, leading to stability as in the case of the TD criterium.
ñ, leading to stability as in the case of the TD criterium.
6 Hyperbolic tangent profiles and the case of uniform magnetic field.
In this section we briefly comment on results of numerical integrations of eq.5 using continuous smooth profiles for all the fields, i.e., with finite values for the length scales D and d defined in section 4. On one hand, the calculations show the significance of the idealized model of section 4, ascertaining that the existence of a long wavelength stability boundary is confirmed working with smooth field profiles. On the other hand, it is found that a configuration without magnetic shear (uniform magnetic field) does not have the long l stability boundary for modes with Bk ¹ 0.
We have considered fields represented by hyperbolic tangent functions, with a length scale, D, for Vk(y), and another scale, d, for both Bk and r. As an alternative, we examine also another configuration in which Bk = B0 is a constant, r = r0 also remains constant, and only Vk varies as an hyperbolic tangent function. The equilibrium fields for both configurations are given by
where Y = y/d. It is worth noting that these steady state field profiles, as well as those of the analytic model (Fig. 1) of a previous section , are exact solutions of the steady state equations of ideal magnetohydrodynamics, eqs. 2 . The hyperbolic tangent function was chosen only as a simple smooth function, continuous together with all its derivatives, that joins two intervals of constant value.
The numerical work was performed with a method of finite differences, applied to a set of first order differential equations equivalent to the second order eq.5 . For the numerical computation with the two-scale model we have taken d/D = 0.2. The configuration with hyperbolic tangents for all the fields was intended to be close to the idealized example of Fig. 1.
We omit figures here for brevity, and report only that the trends revealed by the model of section 4 are qualitatively confirmed by the numerical computations. The hyperbolic tangent model also has a critical value ll, such that modes with l > ll are stable, corresponding to a cutoff of growth rates at small values of kd, similar to that shown in Fig. 2 for the idealized model. The maximum growth rate decreases with diminishing MA as expected, although with values somewhat smaller than those of Fig. 2. Also, the shift of the unstable intervals towards larger values of kd for decreasing values of MA is much reduced in the case of the hyperbolic tangent model. The interested reader may visually compare the results of the hyperbolic tangent profiles with those of the model of section 4 in Gratton et al. [6] where the matter is discussed.
Here, we focus on the results for the alternative configuration with uniform magnetic field. Of course, in this case we may have flute modes with  ^
^  : these are always unstable, except at small ls, a range in which the velocity gradient operates as a stabilizing agent reversing its action as driver of the instability typical of longer wavelengths (see Gratton et al. [9]). Let us consider, instead, modes with Bk = B0¹ 0, for all y. A simple analytic solution for the linear velocity profile, like that of section 4, is not possible in this case in spite of the simplicity of the magnetic field profile, and a numerical treatment is required.
: these are always unstable, except at small ls, a range in which the velocity gradient operates as a stabilizing agent reversing its action as driver of the instability typical of longer wavelengths (see Gratton et al. [9]). Let us consider, instead, modes with Bk = B0¹ 0, for all y. A simple analytic solution for the linear velocity profile, like that of section 4, is not possible in this case in spite of the simplicity of the magnetic field profile, and a numerical treatment is required.
We solve the stability problem for the second option of eqs. 41 . Fig. 5 gives the normalized growth rate Á(w)d/U1 as a function of kd for a set of sixteen values of MA =  U1/B0, ranging from 8.2 down to 2.2 with steps of 0.375. The stabilizing effect of the increasing magnetic field is evident, with the diminution of the growth rates and the shrinking of the unstable interval. More interestingly, Fig. 5 also clearly shows that in this case a long-l stability boundary does not appear.
U1/B0, ranging from 8.2 down to 2.2 with steps of 0.375. The stabilizing effect of the increasing magnetic field is evident, with the diminution of the growth rates and the shrinking of the unstable interval. More interestingly, Fig. 5 also clearly shows that in this case a long-l stability boundary does not appear.
Although the cause of the stabilization at long ls is always the magnetic tension embodied in  ¹ 0, nevertheless the possibility of finding unstable modes at moderate ls that become stable at long ls, is due to the presence of magnetic shear. This is because in the presence of magnetic shear there is a chance that modes may be localized in y intervals of small or zero
¹ 0, nevertheless the possibility of finding unstable modes at moderate ls that become stable at long ls, is due to the presence of magnetic shear. This is because in the presence of magnetic shear there is a chance that modes may be localized in y intervals of small or zero  , as we have discussed in sections 4 and 5.
, as we have discussed in sections 4 and 5.
7 Stability Diagrams
The flow with a constant magnetic field (second alternative in eqs. 41) has a stability diagram shown in Fig. 6. The line gives the critical value of kd that separates stable from unstable modes (for a fixed orientation  ) as a function of MA. For strong magnetic fields, MA < 2, the modes are stable for all wavelengths. When MA is larger than 2 but remains close to this value, MA
) as a function of MA. For strong magnetic fields, MA < 2, the modes are stable for all wavelengths. When MA is larger than 2 but remains close to this value, MA  2, the kd interval of instability is small and the upper kd limit corresponds to rather long wavelengths. However, the instability range starts always at kd = 0. As MA increases significantly above 2 the stability boundary tends to the value kd = 1 and clearly there is only a short ls stability limit. A long-l stability boundary does not exist for this configuration.
2, the kd interval of instability is small and the upper kd limit corresponds to rather long wavelengths. However, the instability range starts always at kd = 0. As MA increases significantly above 2 the stability boundary tends to the value kd = 1 and clearly there is only a short ls stability limit. A long-l stability boundary does not exist for this configuration.
The stability diagrams for the model of section 4, obtained from the dispersion relation 34 , are shown in Figs. 7 and 8 and are very different from the diagram of Fig. 6. In Fig. 7 we present the stability diagram for a density ratio rd = 0.1 in a kd vs. MA plane. The white area corresponds to stable modes, while the shaded regions are unstable. The curves that separate different gray areas correspond to contours of constant Á(w)d/U1. The critical kd line is for Á(w)d/U1 = 0, and it encloses three contour lines of increasing growth rates, with Á(w)d/U1 = (0.03, 0.06, 0.09). In Fig. 7 there are two stability limits kd for each MA value in the range 1.2  MA
MA  3.3. These correspond to the short ls and long ll boundaries mentioned in the introduction. For MA > 3.3 only ls exists and the instability range starts at kd = 0. When MA < 1.2 the magnetic field is sufficiently strong to stabilize the configuration for all ls.
3.3. These correspond to the short ls and long ll boundaries mentioned in the introduction. For MA > 3.3 only ls exists and the instability range starts at kd = 0. When MA < 1.2 the magnetic field is sufficiently strong to stabilize the configuration for all ls.
In Fig. 8 a stability diagram is given for rd = 1 with the same format of Fig. 7. The shape of the contour lines change with the density ratio. The unstable area in Fig. 7 looks like the bow profile of a boat. In Fig. 8 the bow profile has straightened up. The range of MA for which the unstable interval for rd = 1 has both short ls and long ll limits is much smaller than the one corresponding to rd = 0.1.
8 Conclusions
We have shown with a theoretical example that a stable tangential discontinuity may turn out to be unstable when examined at short wavelengths, corresponding to modes that can explore the finite width structure of the interface. Although the configuration is not intended to be realistic, the example has the advantage that it can be solved in closed form and analyzed in detail. Numerical computation of the instability with more realistic hyperbolic tangent profiles, for the same parameters of the idealized model, confirms the results of the analytic study. This is only briefly stated here, but it is discussed in detail in Gratton et al. [6].
The fact that there are cases in which a long ll stability boundary for KH exists, besides the well-known short ls stability limit, as far as we know has not been noted in the literature. The possibility of the existence of a long l stability range relies on the presence of shear in the magnetic field configuration. It is absent for both flute and non flute modes of a unidirectional magnetic field, or in ordinary non conducting fluids. The well-known short wavelength stability interval of KH, instead, is always present and occurs even in the absence of magnetic fields. We have explained the possibility of the existence of the long wavelength limit in MHD parallel flows, by means of a spectral theorem that allows a discussion of the physical effects operating on the instability, and their enhancement or reduction due to the possibility of strong localization of the modes at intermediate ls. We have shown also that this possibility is a MHD property, facilitated by configurations with magnetic shear, which is absent when the magnetic field is uniform.
The KH instability that amplifies surface waves, produces billows and vortices, and finally cascades into turbulence (e.g., Belmont and Chanteur [3]). These processes lead to diffusion and broadening of the velocity gradient, so that the thickness of the transition region increases (see, for instance, Miura [10]). The KH excitation, therefore, is important for the transport of momentum into the terrestrial magnetosphere, particularly during periods of northward orientation of the IMF (Farrugia et al.[5]). In relation to data analysis of spacecraft crossings, the reader should realize that the TD condition 1 is not sufficient to asses the KH stability of the magnetopause. To determine the theoretical stability of a KH configuration the finite thickness structure of the interface must be taken into account. On the other hand, when the TD is unstable, a more precise analysis will also confirm the instability. In that case, however, one should remember that the TD equation grossly overestimates the growth rate for l ~ D and completely ignores the stabilization at short ls. Readers interested to see cases of practical application of the long stability limit to the study of the stability of the magnetopause are referred to Gratton et al. [6] where the subject is treated in detail.
The theory presented here is limited to subsonic flows. An extension of the stability diagrams for supersonic flows requires the inclusion of compressibility effects in the perturbative equations and remains a subject for future studies.
Acknowledgments
Research funded by the Argentinean grants UBACyT X059, X291, and CONICET PIP 2013. Also partially supported by USA NASA grants NAG5-13116, NAG5-11803. LB was a postdoc fellow of Fundación Bunge y Born, Buenos Aires, Argentina, during part of the work.
Received on 08 February, 2004; revised version received on 14 May, 2004
- [1] S. Chandrasekhar, Hydrodynamic and hydromagnetic stability, Oxford University Press, New York, 1961.
- [2] P.G. Drazin, and W. H. Reid, Hydrodynamic stability, Cambridge University Press, Cambridge, 1981.
- [3] G. Belmont, and G. Chanteur, Physica Scripta, 124, 124 (1989).
- [4] P. Huerre, and M. Rossi, Hydrodynamic instabilities in open flows, in Hydrodynamics and Non Linear Instabilities, C. Godreche and P. Manneville e5ditors, Cambridge University Press, Cambridge, 1998.
- [6] C.J. Farrugia, F. T. Gratton, and R. B. Torbert, Space Science Rev. 95, 443 (2001).
- [7] F.T. Gratton, L. Bender, C. J. Farrugia, and G. Gnavi, J. Geophys. Res. 109, A04211 (2004).
- [8] J. Contin, F. T. Gratton, and C. J. Farrugia, J. Geophys. Res. 108, 1227 (2003).
- [9] F.T. Gratton, G. Gnavi, C. J. Farrugia, and L. Bender, J. Planet. & Space Sci. 51, 769 (2003).
- [10] A. Miura, J. Geophys. Res. 89, 801 (1984).
Publication Dates
-
Publication in this collection
01 Mar 2005 -
Date of issue
Dec 2004
History
-
Received
08 Feb 2004 -
Accepted
14 May 2004


















































