A metric property of umbilic points

A metric property of umbilic points

Ronaldo Garcia^I; Jorge Sotomayor^II

^IInstituto de Matemática e Estatística, Universidade Federal de Goiás Caixa Postal 131, 74001-970 Goiânia, GO, Brasil

^IIInstituto de Matemática e Estatística, Universidade de São Paulo Cidade Universitária, 05508-090 São Paulo, SP, Brasil

^{Correspondence}

ABSTRACT

In the space of cubic forms of surfaces, regarded as a G-space and endowed with a natural invariant metric, the ratio of umbilic points with a negative index to those with a positive index is evaluated in terms of the asymmetry of the metric, defined here. This work contains also a section with references pertinent to umbilic points and one with a discussion of a connection of the above defined ratio with that reported in 1977 by Berry and Hannay in the domain of Statistical Physics.

Key words: Umbilic point, principal curvature lines.

RESUMO

No espaço das formas cúbicas de superfícies, consideradas como um G-espaço e munido de uma métrica invariante, é avaliado o quociente dos volume das formas cúbicas correspondentes aos umbílicos de índice negativo pelo volume daquele correspondente aos umbíicos de índice positivo. Esta avaliação é expressa em termos da assimetria da métrica, definida neste artigo. Este trabalho contém também uma seção com referências a trabalhos relacionados aos pontos umbílicos e outra com uma comparação do quociente acima citado com o obtido em 1977 por Berry e Hannay no domínio da Física Estatística.

Palavras-chave: ponto umbílico, linhas de curvaturas principais.

1 UMBILIC POINTS, INVARIANT METRICS AND VOLUME RATIOS

At an umbilic point p of an oriented C³ surface S embedded in an oriented Euclidean 3-space 3 the principal curvatures coincide. In a neighborhood of such point, S can be written in a Monge chart as the graph z = h(x,y) of a function of the form

The frame (x,y;z) is positive and adapted to S at p. This means that the plane orthonormal frame (x,y) is attached to the tangent plane, positively oriented, and the z-axis is along the unit positive normal to S at p.

Any other such presentation as the graph Z = H(X,Y) of a function

differs by a rotation

x = cosqX-sinqY, y = sinqX+cosqY, z = Z,

linking the positively oriented frames (X,Y;Z) and (x,y;z), adapted to the surface at p.

The functions are related by H(X,Y) = h(x,y); substitution leads to

Thus the group (2) of rotations in the plane acts linearly, to the right, on the four dimensional space of real cubic forms

identified with line vectors u = (a, b, b¢, a¢) in 4.

Denote by W(q) the matrix of the linear transformation in 4, corresponding to the frame rotation by an angle q. That is U = uW(q), with U = (A,B,B¢,A¢) and u = (a, b, b¢, a¢).

From equation 2, we get

The space 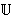4 of umbilic intrinsic cubic forms of surfaces is defined as the G-Space ⁴, endowed with the above action of the group G = (2).

The quadratic form

is invariant under G = (2). That is T (U) = T (u) if U = uW(q). Thus it is defined on 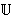4. It characterizes the transversal umbilic points, as those with T (u) ¹ 0.

It is well known that the Index I (u) of a transversal umbilic u is I (u) = sign(T (u)). See (Bruce and Fidal 1989) and (Gutierrez and Sotomayor 1982, 1983) where the identification of T ¹ 0 with the transversality to the manifold of umbilic 2-jets is made.

The index of an isolated umbilic counts the number of turns made by a principal direction at a point of the surface that makes a small circuit around the umbilic, (Spivak 1980) and (Smyth and Xavier 1992).

According to (Spivak 1980) and (Struik 1988), the differential equation of principal lines around p in this chart is defined as a variety in the projective bundle. In the chart (x, y, [dx : dy]), the variety is given by the equation:

where the functions L, M and N are:

and therefore T (u) = .

THEOREM 1. (Gutierrez and Sotomayor 1982, 1991, Bruce and Fidal 1989). Let p be an umbilic point and consider the Monge chart as in equation (1). The transversality condition T ¹ 0 holds if and only if the surface P ¹ 0 in equation 5 is regular along the projective line x = y = 0 covers a punctured neighborhood of p. Then it defines a cylinder whose projection, with the projective line removed, covers twice a punctured neighborhood of p, one for each of the two open cylinders –one for each direction field– resulting from the removal of the projective line. The covering is orientation preserving or reversing according toT >0 orT <0. See Figure 1 for an illustration of one of the cylinders and its projection.

A metric in 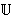4 is a positive definite quadratic form such as q(u) = uQu*, Q being a positive definite real 4×4 symmetric matrix, invariant under the action of (2). That isQ = W(q)QW(q)*, for all q.

PROPOSITION 1. Any metric q(u) = uQu*, invariant under the action of (2), is given by Q of the form:

where b > 0, and b( a + b) - a² > 0, which gives the positivity of Q.

PROOF. The proof follows by solving the equation Q = W(q)QW(q)* first for q = p/2 and q = p/4 and finally checking that it holds for all q, the proof follows.

A criterion for the positivity of a symmetric matrix, consists of the positivity of all principal minors (Gantmacher 1960), Chap. X, page 306. Notice that for Q in (6) the positivity of the second third principal minors imply that of the other two.

REMARK 1. Another way to obtain the expression of Q in (6) consists in projecting the 10 dimensional space 10 of 4 × 4 symmetric matrices M via the averaging along the orbits of W(q):

Denoting by m_ij the entries of the symmetric matrix M, integration in expression (7) gives:

for the invariant symmetric matrix (M). The other entries of Q in (6) are also corroborated by integration in (8).

For the identity matrix I , (I) has a = 1/4, b = 7/4.

PROPOSITION 2. The planes 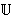₁ : a = 3b¢, a¢ = 3b and 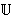₂ : a = -b¢, a¢ = -b are invariant under the action of (2). These spaces are mutually orthogonal, relative to q.

The quadratic forms r₁ = r₁₁² + r₁₂²and r₂ = r₂₁² + r₂₂², where

r₁₁ = (a + b¢)/8, r₁₂ = -(a¢ + b)/8, r₂₁ = (a - 3b¢)/24, r₂₂ = (a_¢ - 3b)/24,

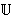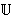are invariant under the action of(2). Also r₁and r₂vanish respectively on₂ and ₁.

Furthermore, the symmetric matrices R₁and R₂which define r₁and r₂generate the lines b = a and a = -b/3, which form the border of the admissible region b(a + b) - a² > 0, in Proposition 1.

PROOF. The invariance of the planes is straightforward.

The plane

₁ is spanned by u_1,1 = (3, 0, 1, 0) and u_1,2 = (0, 1, 0, 3);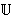 ₂ is spanned by u_2,1 = (-1, 0, 1, 0) and u_2,2 = (0, 1, 0,-1). Scalar multiplication relative to Q of these vectors ends the proof.

The second and third items follow from a straightforward calculation.

Although other possibilities exist, in this work the forms r₁ and r₂ will be used as a reference.

DEFINITION 1. Let q(u) = uQu* be as in Proposition 1. Write, q₁ = r₁/m , q₂ = r₂/m , for positive constants m₁ and m₂, uniquely determined by q_i = q| , i = 1, 2.

The asymmetry of q is defined by the ratio s(q) = m₂/m₁.

Clearly s(q) = m₂/m₁ ranges over all positive reals. Its expression in terms of a, b is given in proposition 3.

THEOREM 2. Let T be the quadratic form in equation 4, giving the index of transversal umbilic points.

Relative to unit ball B(1, q) = {q(u) < 1} of any invariant metric q in 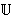4, the ratio of the volume V_ of the cone C_, where T is negative, to that of the volume V₊ of the cone C₊, where T is positive, is given by 9(s (q))², where s(q) is the asymmetry of q, as in Definition 1 and Proposition 3.

Proof. Direct calculation leads to

T = 72(-r₂ + r₁/9).

Therefore, in terms of q₁, q₂,

The proof consists in computing the volume V_ of the solid torus cone

and divide it by the volume V₊ of the solid torus cone

Let u_i1, u_i2 be an orthonormal basis of 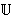_i, i = 1, 2, relative to qi , so that they form a positive orthonormal frame, relative to q, on 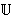4.

In q-orthonormal coordinates (x, y, z,w) relative to the frame u_i1, u_i2, i = 1, 2, it follows that

Let x = r cos q, y = r sin q and z = R cos g, w = R sin g , where 0 < r < 1, 0 < R < 1, 0 < q < 2p, 0 < g < 2p.

The element of volume dV in the metric q is given by dxdydzdw

Therefore, dV = r Rdrd Rdqdg and

Considering the change of coordinates r = t cos b, R = t sin b, 0 < b < p/2, 0 < t < 1, we obtain

Therefore, as it is well known, the volume of the unit ball in a four dimensional Euclidean space is given by V₁ = ò _q<1dV = p²/2. See (Courant and John 1989), pg. 459.

Take tan b₀ = , the volume of the solid torus cone C₊ is given by,

Analogously, the volume of the solid torus cone C_ is equal to

Therefore

PROPOSITION 3. In terms of Q, as in equation 6, s(q) is calculated as follows:

PROOF. In fact, by the uniqueness of the simultaneous diagonalization of the quadratic forms q and T , see (Gantmacher 1960) pg. 314, equation 9 implies that the eigenvalues of matrix MT , of T, relative to Q, the matrix of q, are 72()² and -72m.

Separate direct calculation of these relative eigenvalues, which are those of the matrix M_TQ^-1, gives and - .

Equating the ratios of the eigenvalues in both calculations gives 9

² = 3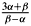, which amounts to equation (10).

2 AT THE CROSSROADS OF GEOMETRY AND GLOBAL ANALYSIS

The Geometric local properties of umbilic points, regarded as singularities, have been studied focusing on the three following main aspects:

i) Topological, related to the Index sign of the principal line fields around the umbilic.

ii) Focal, describing the patterns, Hyperbolic Elliptic, of normal rays envelopes. This aspect is related to Geometric Optics, Catastrophe Theory and Lagrangian Geometry. See (Thom 1972) and (Wall 1977).

iii) Darbouxian, which counts the number of principal lines separatrices approaching the umbilic, and, more generally, describes locally the foliations by principal lines.

These aspects and their different types are discriminated and analyzed in terms of suitable algebraic conditions in the G-space 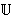4.

A coherent differential geometric and topological picture of the set morphology and inclusion relationships between the different sorts of umbilic types has been established by Porteous, see (Porteous 1994), and previous reference quoted there. See also (Zeeman 1976), for the focal aspect, and (Darboux 1896), (Gutierrez and Sotomayor 1982, 1983, 1991) and (Bruce and Fidal 1989), for the Darbouxian types.

The globalization to the whole surface of the local analysis of Darboux, in the context of Structural Stability and Genericity of principal foliations, was carried out in (Gutierrez and Sotomayor 1982, 1983, 1991).

An additional extension led Gutierrez, Garcia, Sotomayor and others, to expand the study of umbilic points and also principal foliations to surfaces and hypersurfaces in 4. See (Gutierrez et al. 1997), (Garcia and Sotomayor 2000) and (Garcia 2001).

Other foliations of interest in Classical Differential Geometry, such as asymptotic lines and lines of mean curvature, defined also by quadratic differential equations similar to (5), have been studied in (Garcia and Sotomayor 1997) and (Garcia et al. 1999) and (Garcia and Sotomayor 2001, 2002, 2003).

There remain deep open problems related to the structure of principal foliations around isolated umbilic points on smooth surfaces, in the non-transversal case. See (Mello and Sotomayor 1999), (Smyth and Xavier 1992) and (Ivanov 2002).

3 UMBILIC POINTS IN RANDOM SURFACES

In the domain of Statistical Physics, but still connected to Geometry and Topology, Berry and Hannay (Berry and Hannay 1977) carried out a quantitative statistical study of the proportions in which the different types of umbilic types are distributed in random surfaces, such as those modeling an ocean or a lake. An issue here is to study how the presence of umbilic points in a random surface influences the reflection on it of electromagnetic short waves. Although this work is more related to the focal interpretation of umbilic points, it considers explicitly also their Darbouxian and Index aspects.

This paper is the outcome of an initial attempt to provide a mathematical formulation and a proof, in the tradition of Geometry and Classical Analysis, that could correspond to the conclusions of (Berry and Hannay 1977), reported in the tradition of Statistical Physics.

Theorem 2 suggests a disagreement with the report of the calculations in (Berry and Hannay 1977) which claim that the statistical ratio is always 1, irrespective of the statistic anisotropy present in the evaluation. The asymmetry of the invariant metric, used to make evaluations in this work, may be considered as a geometric counterpart for the statistic anisotropy.

Considering only the local aspect of surfaces at umbilic points, this discrepancy may be due to the fact that in the calculations made in (Berry and Hannay 1977), the cubic forms are regarded as vectors in 4, with a fixed frame, and not as elements of the G-space 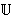4. The effect of this is that the same umbilic on a surface is counted multiple times, one for each rotated frame.

ACKNOWLEDGMENTS

The first author was partially supported by FUNAPE/UFG. Both authors are fellows of CNPq. This work was done under the project PRONEX/FINEP/MCT-Conv. 76.97.1080.00 - Teoria Qualitativa das Equações Diferenciais Ordinárias and CNPq-Grant 476886/2001-5.

Manuscript received on May 13, 2003; accepted for publication on August 5, 2003; contributed by JORGE SOTOMAYOR*

AMS Classification: 53C12, 34D30, 53A05, 37C75.

• BERRY M AND HANNAY J. 1977. Umbilic points on Gaussian Random Surfaces, J Phys A 10: 1809-1821.
• BRUCE B AND FIDAL D. 1989. Onbinary differential equations and umbilic points, Proc Royal Soc Edinburgh 111A: 147-168.
• COURANT R AND JONH F. 1989. Introduction to Calculus and Analysis,Vol. II, NewYork: Springer Verlag.
• DARBOUX G. 1896. Leçons sur la Théorie des Surfaces, vol. IV. Sur la forme des lignes de courbure dans la voisinage d'un ombilic, Note 07, Paris:Gauthier Villars.
• GANTMACHER FR. 1960. Matrix Theory, Vol. I, Chelsea.
• GARCIA R. 2001. Principal Curvature Lines near Darbouxian Partially Umbilic Points of Hypersurfaces Immersed in R⁴, Computational and Applied Mathematics 20: 121-148.
• GARCIA R AND SOTOMAYOR J. 1997. Structural stability of parabolic points and periodic asymptotic lines, Matemática Contemporânea 12: 83-102.
• GARCIA R AND SOTOMAYOR J. 2000. Lines of Axial Curvature on Surfaces Immersed into R ⁴, Differential Geometry and its Applications 12: 253-269.
• GARCIA R AND SOTOMAYOR J. 2001. Structurally stable configurations of lines of mean curvature and umbilic points on surfaces immersed in R³, Publ Matemátiques 45: 431-466.
• GARCIA R AND SOTOMAYOR J. 2002. Lines of Geometric Mean Curvature on surfaces immersed in R³, Annales de la Faculté des Sciences de Toulouse 11: 377-401, arXiv:math.DS/0302194 v1, 2003.
• GARCIA R AND SOTOMAYOR J. 2003. Lines of Harmonic Mean Curvature on surfaces immersed in R³, Boletim da Soc Bras de Matemática (Bull Bras Math Soc) 34: 303-331, arXiv:math.DS/0302196 v1, 2003.
• GARCIA R, GUTIERREZ C AND SOTOMAYOR J. 1999. Structural Stability of Asymptotic Lines on Surfaces Immersed in R3, Bulletin de Sciences Mathématiques 123: 599-622.
• GUTIERREZ C AND SOTOMAYOR J. 1982. Structurally Stable Configurations of Lines of Principal Curvature, Asterisque 98-99: 185-215.
• GUTIERREZ C AND SOTOMAYOR J. 1983. An Approximation Theorem for Immersions with Structurally Stable Configurations of Lines of Principal Curvature, Lect Notes in Math 1007: 332-368.
• GUTIERREZ C AND SOTOMAYOR J. 1991. Lines of Curvature and Umbilic Points on Surfaces, 18^th Brazilian Math. Colloquium, Rio de Janeiro, IMPA, Reprinted with update as Structurally Stable Configurations of Lines of Curvature and Umbilic Points on Surfaces, Lima, Monografias del IMCA, 1998.
• GUTIERREZ C GUADALUPE I, TRIBUZY R AND GUÍÑEZ V. 1997. Lines of curvature on surfaces immersed in R⁴, Bol Soc Bras Mat 28: 233-251.
• IVANOV VV. 2002. The analytic conjecture of Carathéodory, Siberian Math 43: 251-322.
• MELLO LF AND SOTOMAYOR J. 1999. A note on some developments on Carathéodory Conjecture on umbilic points, Expo Math 17: 49-58.
• PORTEOUS IR. 1994. Geometric Differentiation, Cambridge Univ Press.
• SMYTH B AND XAVIER F. 1992. A sharp geometric estimate for the index of an umbilic point on a smooth surface, Bull. London Math Soc 24: 176-180.
• SPIVAK M. 1980. Introduction to Comprehensive Differential Geometry, Vol. III Berkeley, Publish or Perish.
• STRUIK D. 1988. Lectures on Classical Differential Geometry, Addison Wesley Pub. Co., Reprinted by Dover Publications, Inc.
• THOM R. 1972. Stabilité Structurelle et Morphogénèse,W. A. Benjamin, Inc.
• WALL TCT. 1977. Geometric properties of generic differentiable manifolds, Springer Lecture Notes in Mathematics 597: 707-774.
• ZEEMAN EC. 1976. The umbilic bracelet and the double cusp catastrophe, Springer Lecture Notes in Mathematics 525: 328-366.

Publication Dates

• Publication in this collection
  04 Nov 2003
• Date of issue
  Dec 2003

History

• Accepted
  05 Aug 2003
• Received
  13 May 2003