Abstracts
In this article, we show the relation between the Schwartz kernels of the Dirichlet-to-Neumann operators associated to the metrics g0 and h = F* (e²φ g0) on the circular annulus A R, and the Schwarzian Derivative of the argument function f of the restriction of the diffeomorphism F to the boundary of A R.
annulus; Dirichlet-to-Neumann Functional; Schwarzian Derivative
Neste artigo mostramos a relação entre os núcleos de Schwartz dos operadores Dirichlet-to-Neumann associados à métrica g0 e h = F* (e²φ g0), no anel circular A R, e a Derivada Schwarziana da função argumento f, da restrição do difeomorfismo F à fronteira de A R.
anel; Funcional Dirichlet-Neumann; Derivada Schwarziana
MATHEMATICAL SCIENCES
Injectivity of the Dirichlet-to-Neumann Functional and the Schwarzian Derivative
Fernando A.F.C. SilvaI; Pedro A.G. VenegasII; Ramón O.M. AhumadaI
IUniversidade Federal de Pernambuco, CCEN Departamento de Matemática, Av. Prof. Luiz Freire s/n Cidade Universitária, 50740-540 Recife, PE, Brasil
IIUniversidade Federal da Paraíba, CCEN Departamento de Matemática, Cidade Universitária 58059-970 João Pessoa, PB, Brasil
Correspondence to Correspondence to: Ramón Orestes Mendoza Ahumada E-mail: ramon@dmat.ufpe.br
ABSTRACT
In this article, we show the relation between the Schwartz kernels of the Dirichlet-to-Neumann operators associated to the metrics g0 and h = F* (e2φ g0) on the circular annulus AR, and the Schwarzian Derivative of the argument function f of the restriction of the diffeomorphism F to the boundary of AR.
Key words: annulus, Dirichlet-to-Neumann Functional, Schwarzian Derivative.
RESUMO
Neste artigo mostramos a relação entre os núcleos de Schwartz dos operadores Dirichlet-to-Neumann associados à métrica g0 e h = F* (e2φ g0), no anel circular AR, e a Derivada Schwarziana da função argumento f, da restrição do difeomorfismo F à fronteira de AR.
Palavras-chave: anel, Funcional Dirichlet-Neumann, Derivada Schwarziana.
INTRODUCTION
Let  denote the space of all Riemannian metrics on a compact manifold,
denote the space of all Riemannian metrics on a compact manifold,  with
with  boundary, and denote by
boundary, and denote by  the space of continuous linear operators acting on C∞(∂Ω).
the space of continuous linear operators acting on C∞(∂Ω).
The Dirichlet-to-Neumann functional  is a mapping from
is a mapping from  into
into  such that, for each,
such that, for each,  ,
,  takes Dirichlet boundary values to Neumann boundary values. More precisely, if
takes Dirichlet boundary values to Neumann boundary values. More precisely, if  is the unique solution of the Dirichlet problem
is the unique solution of the Dirichlet problem  in Ω,
in Ω,  , then,
, then, where
where  (resp.
(resp.  ) is the Laplace-Beltrami operator (resp. unit interior normal vector field) associated to the metric. The study of this functional goes back to the seminal paper of (Calderón 1980).
) is the Laplace-Beltrami operator (resp. unit interior normal vector field) associated to the metric. The study of this functional goes back to the seminal paper of (Calderón 1980).
It is known (Lee and Uhlmann 1989) that  is in fact an elliptic self-adjoint pseudo-differential operator of order one, whose principal symbol is
is in fact an elliptic self-adjoint pseudo-differential operator of order one, whose principal symbol is  ,
,  , and
, and  .
.
Let  be the group of diffeomorphism of
be the group of diffeomorphism of  . The semi-direct product
. The semi-direct product  (Polyakov 1987) of the groups
(Polyakov 1987) of the groups  and
and  defined by
defined by
provides a natural right action on  , given by
, given by
where  denotes the pull-back of F.
denotes the pull-back of F.
The main obstruction to injectivity, in the two-dimensional case, is the semidirect product of the groups of diffeomorphisms that restricts to the identity on the boundary, and the Abelian group of real-valued functions that equals zero on it. In fact, as formula (2.1) shows, the Dirichlet-to-Neumann Functional is constant on the orbits determined by  ; this is a normal subgroup of
; this is a normal subgroup of  .
.
With respect to the determination of the metric  from the Dirichlet-to-Neumann Operator, we recommend the papers (Lee and Uhlmann 1989), (Lassas and Uhlmann 2001) and (Lassas et al. 2003). In these papers, they solve, in a more general setting, the problem of recovering the manifold and the metric.
from the Dirichlet-to-Neumann Operator, we recommend the papers (Lee and Uhlmann 1989), (Lassas and Uhlmann 2001) and (Lassas et al. 2003). In these papers, they solve, in a more general setting, the problem of recovering the manifold and the metric.
In the case of a fixed annulus, all metrics can be written as  for
for  coming from the pull-back of the euclidean metric in the annulus of radius
coming from the pull-back of the euclidean metric in the annulus of radius  and
and  . We prove, in this special case, that the equality of the Dirichlet-to-Neumann Operators associated to both metrics
. We prove, in this special case, that the equality of the Dirichlet-to-Neumann Operators associated to both metrics  and
and  gives us a relation involving the Schwarzian derivative of
gives us a relation involving the Schwarzian derivative of  (
(  the lifting to
the lifting to  of the restriction to the boundary of the diffeomorphism
of the restriction to the boundary of the diffeomorphism  ).
).
Furthermore, we also show that the conformal factor restricted to the boundary of the annulus is determined by  .
.
More precisely, we shall prove in Section 2 that, if  is the annulus
is the annulus
 is conformal to the euclidean metric,
is conformal to the euclidean metric,  , where
, where  and
and  ; the equality of the Schwartz kernels of
; the equality of the Schwartz kernels of  and of
and of  implies that the argument function
implies that the argument function  , of the restriction of
, of the restriction of  to
to  , satisfies the differential equation
, satisfies the differential equation
and  denotes the Schwarzian Derivative of
denotes the Schwarzian Derivative of  . It follows that, if
. It follows that, if  , then,
, then,  and
and  equals zero on the boundary.
equals zero on the boundary.
2 GEOMETRIC FORMULATION
Here on we will denote by  the Schwartz kernel of
the Schwartz kernel of  . We start with two lemmas.
. We start with two lemmas.
LEMMA  . Given a two-dimensional compact manifold
. Given a two-dimensional compact manifold  with
with  boundary and,
boundary and, ,
,
 and,
and, we have
we have
Proof. See (Gómez and Mendoza 2006).
LEMMA  . Let
. Let  be a two-dimensional compact manifold with
be a two-dimensional compact manifold with  boundary,
boundary,  where
where  ,
,
 ,
,  and
and  the unitary vector field to, with respect to the metric. Then,
the unitary vector field to, with respect to the metric. Then,
where  denotes the real, valued function on
denotes the real, valued function on  such that
such that  .
.
Proof. Let  ,
,
changing variables  we get:
we get:
where  denotes the unique real, valued function defined on
denotes the unique real, valued function defined on  such that
such that
and  is the tangent unitary vector field on
is the tangent unitary vector field on  such that
such that  and
and  . The above equation means at every point
. The above equation means at every point  the following:
the following:  and
and  belong to the same one-dimensional tangent space
belong to the same one-dimensional tangent space  ; consequently, the first one is a real multiple of the second. In fact, this multiple is unique and it is equal to
; consequently, the first one is a real multiple of the second. In fact, this multiple is unique and it is equal to  .
.
For the second equality,
finishing the proof.
The next Lemma establish, a relation between  and the Green function
and the Green function  of the Laplacian
of the Laplacian  with Dirichlet condition on
with Dirichlet condition on  (Guillarmou and Sá Barreto 2009).
(Guillarmou and Sá Barreto 2009).
LEMMA  . The Schwartz kernel
. The Schwartz kernel  of
of  is given for y, y' ∈ ∂Ω, y ≠ y', by
is given for y, y' ∈ ∂Ω, y ≠ y', by
where,
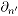 are, respectively, the inward pointing vector fields to the boundary in variable
are, respectively, the inward pointing vector fields to the boundary in variable 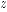 and
and 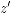 .
.
Proof. Let  be the distance function to the boundary in
be the distance function to the boundary in  ; it is smooth in a neighborhood of
; it is smooth in a neighborhood of  and the normal vector field to the boundary is the gradient
and the normal vector field to the boundary is the gradient  of
of  . The flow
. The flow  of
of  induces a diffeomorphism
induces a diffeomorphism  defined by,
defined by, and we have
and we have  . This induces natural coordinates
. This induces natural coordinates  near the boundary, these are normal geodesic coordinates. The function
near the boundary, these are normal geodesic coordinates. The function  is the unique solution of the Dirichlet problem
is the unique solution of the Dirichlet problem  in, and
in, and  can be obtained by taking
can be obtained by taking
where  is any smooth function on
is any smooth function on  such that
such that  . Now, using Greens formula and
. Now, using Greens formula and  , where
, where  is the Dirac mass on the diagonal, we obtain for
is the Dirac mass on the diagonal, we obtain for 
We have Taylor expansion  near the boundary. Let
near the boundary. Let  and take
and take  supported near. Thus, pairing with
supported near. Thus, pairing with  gives
gives
Now taking  with support disjoint to the support of
with support disjoint to the support of  , thus
, thus  , and differentiating (2.2) in x, we see, in view of the fact that Greens function
, and differentiating (2.2) in x, we see, in view of the fact that Greens function  is smooth outside the diagonal, that
is smooth outside the diagonal, that
which proves the claim.
Let  be a Riemannian manifold, and let us denote by
be a Riemannian manifold, and let us denote by  the geodesic distance between
the geodesic distance between  , and we denote
, and we denote  . If
. If
does not depend on x, we have the following result:
COROLLARY  . If
. If  then
then  for
for  .
.
PROOF. Using the equalities of the Dirichlet-to- Neumann operators and Lemma 2.2 we have
On the other hand, since
then, taking the limit when  in (2.3), the demonstration follows.
in (2.3), the demonstration follows.
REMARK 2.5. From LEMMA 2.2 and COROLLARY 2.4 we have the following equation,
The set of solutions of equation (2.4) is a group with multiplication law given by composition of functions, that is, if  and
and  are solutions of the equation (2.4), then,
are solutions of the equation (2.4), then,  is solution of (2.4). In fact,
is solution of (2.4). In fact,
In what follows, we use an explicit formula for the Greens Function of  on the annulus
on the annulus  (Bârza and Guisa 1998). There,
(Bârza and Guisa 1998). There,  is given in polar coordinates by:
is given in polar coordinates by:
and it is conformal to the euclidean metric, with conformal factor .
.
Then, the normal derivative of  with respect to
with respect to  on, is
on, is  , is:
, is:
Analogously, the normal derivative of, with respect to  on, is
on, is  :
:
The Greens function of  is given by
is given by
where  ,
,  ,
,  ,
,  ,
,  ,
,  .
.
LEMA  . The Schwartz kernel of Λg0,
. The Schwartz kernel of Λg0,  being of the form, is
being of the form, is
The equality above is in the distributions sense.
PROPOSITION 2.7 . Let p ,q Î ¶ Ar then,
where  denotes the geodesic distance between
denotes the geodesic distance between  and
and  with respect to the Euclidean metric in ∂ Ar.
with respect to the Euclidean metric in ∂ Ar.
Proof. In order to prove equation (2.8), we write
Then, the sequence  has the following property:
has the following property:  for all
for all  where
where  is a constant that depends only on k and. In fact,. Hence, the series
is a constant that depends only on k and. In fact,. Hence, the series  represents a
represents a  function. On the other hand, using the Fourier series of the function, with, we have that
function. On the other hand, using the Fourier series of the function, with, we have that
that is,
which implies:
the equality being in the distributions sense.
Then, multiplying (2.6) by  and taking the limit as
and taking the limit as  , we get the following:
, we get the following:
Analogously, we get (2.9).
REMARK  . It follows from the proof of the Proposition (2.6) that the Schwartz kernel of
. It follows from the proof of the Proposition (2.6) that the Schwartz kernel of  can be written as:
can be written as:
where  is a
is a  function given by
function given by
TEOREMA  . Let
. Let  be a metric as in,
be a metric as in,  where,
where,  ,
,  and.
and. If,
If, then,
then,
where  denotes the Schwarzian Derivative of
denotes the Schwarzian Derivative of  (see
(see  and the line right after it).
and the line right after it).
Proof. Using the equality of the Dirichlet-to-Neumann operators, it follows from Lemma 2.2 that
Writing,  and using (2.8), we have that
and using (2.8), we have that
On the other hand, we have from Corollary 2.4 and (2.8) that  on the boundary. Hence,
on the boundary. Hence,
We obtain, then,
Since the left hand side of the equation (2.12) is the  component of the Schwartz kernel, then if we take
component of the Schwartz kernel, then if we take  , we get
, we get
In what concerns the right hand side of the equation (2.12), we use Taylor expansion of order  of the expression in brackets, for
of the expression in brackets, for  near
near  ; we get, with
; we get, with  ,
,
which can be written as follows,
Since the limit exists, when d ® 0, we obtain from (2.12) and (2.13) that
which implies:
or, more precisely:
Let us λ= λ (R) denote, the expression
Then, it, follows that
where
From equations (2.14) and (2.15) we have that
which implies:
The right-hand side of (2.18) is called the Schwarzian Derivative  of
of  (Navas 2007).
(Navas 2007).
REMARK  . The numerical study of
. The numerical study of  defined in (2.16) is done in Mendoza et al. 2009.
defined in (2.16) is done in Mendoza et al. 2009.
COROLLARY  . The solution of the equation
. The solution of the equation  for
for  is
is  .
.
Proof. Making the change of variables:  , the equation (2.18) becomes
, the equation (2.18) becomes
Since,f(θ + 2π) =f (θ + 2π) we have that  and
and  are periodic of period 2π. Then, integrating (2.20) between
are periodic of period 2π. Then, integrating (2.20) between  and 2π we obtain
and 2π we obtain
On the other hand,
that is,
which implies that  . Because there is
. Because there is  such that
such that  , we get
, we get  . Therefore,
. Therefore, .
.
It follows that  restricted to the exterior boundary is a rotation and
restricted to the exterior boundary is a rotation and  equals zero there. The same conclusion holds for the restriction of
equals zero there. The same conclusion holds for the restriction of  to the interior boundary.
to the interior boundary.
The general solution of the equations (2.18) can be obtained using the formulas of Chuaqui et al. 2003, page 1.
ACKNOWLEDGMENTS
We thank Gustavo Tamm and Henrique Araújo for helping us to solve and to understand the non-linear ordinary differential equation (2.18).
Manuscript received on October 1, 2008; accepted for publication on August 11, 2010
- BÂRZA I AND GUISA D. 1998. Explicit Formulas for Green's Functions on the Annulus and on the Möbius Strip. Acta Appl Math 54: 289-302.
- CALDERÓN AP. 1980. On an inverse boundary value problem. Seminar on Numerical Analysis and its applications to continuum physics. Soc Bras Mat, Rio de Janeiro, RJ, Brasil, p. 65-73.
- CHUAQUI M, DUREN P AND OSGOOD B. 2003. The Schwarzian derivative for harmonic mappings. J d'Analyse Math 91(1): 329-351.
- GÓMEZ P AND MENDOZA R. 2006. Uniqueness for the Dirichlet-Neumann elliptic functional. Inverse Problem 22: 1575-1578.
- GUILLARMOU C AND SÁ BARRETO A. 2009. Inverse Problems for Einstein Manifolds. Inverse probl Imag 3:1-15.
- LASSAS M AND UHLMANN G. 2001. On Determining a Riemannian manifold from the Dirichlet-to-Neumann map. Ann Sci École Norm Sup 34(5): 771-787.
- LASSAS M, TAYLOR M AND UHLMANN G. 2003. The Dirichlet-Neumann map for complete Riemannian manifold with boundary. Comm Anal Geom 11: 207-221.
- LEE J AND UHLMANN G. 1989. Determining anisotropic real analytic condutivities by boundary measurements. Comm Pure Appl Math XLII: 1097-1112.
- MENDOZA R, ROJAS J, CAMPOS M AND BARRETO R. 2009. Injetividade do Funcional de Dirichlet-Neumann Elíptico via Matemática Intervalar. Temas, SBMAC 10: 31-40.
- NAVAS A. 2007. Grupos de difeomorfismos del círculo. Ensaios Matemáticos 13, SBM.
- POLYAKOV AM. 1987. Gauge Fields and Strings. Harwood Academic Publishers, Chur.
Publication Dates
-
Publication in this collection
28 Feb 2011 -
Date of issue
Dec 2010



























































