Stochastic multi-scale analysis of homogenised properties considering uncertainties in cellular solid microstructures using a first-order perturbation

Stochastic multi-scale analysis of homogenised properties considering uncertainties in cellular solid microstructures using a first-order perturbation

Khairul Salleh Basaruddin^*; Nur Saifullah Kamarrudin; Ishak Ibrahim

School of Mechatronic Engineering, Universiti Malaysia Perils, 02600 Ulu Pauh, Perils, Malaysia

ABSTRACT

Randomness in the microstructure due to variations in microscopic properties and geometrical information is used to predict the stochastically homogenised properties of cellular media. Two stochastic problems at the micro-scale level that commonly occur due to fabrication inaccuracies, degradation mechanisms or natural heterogeneity were analysed using a stochastic homogenisation method based on a first-order perturbation. First, the influence of Young's modulus variation in an adhesive on the macroscopic properties of an aluminium-adhesive honeycomb structure was investigated. The fluctuations in the microscopic properties were then combined by varying the microstructure periodicity in a corrugated-core sandwich plate to obtain the variation of the homogenised property. The numerical results show that the uncertainties in the microstructure affect the dispersion of the homogenised property. These results indicate the importance of the presented stochastic multi-scale analysis for the design and fabrication of cellular solids when considering microscopic random variation.

Keywords: stochastic multi-scale, perturbation method, cellular media, macroscopic property.

1 INTRODUCTION

Applications of cellular materials in various areas have been increasing in recent decades; these applications are not limited to functional uses but also include structural uses. Designing a microstructure to obtain certain desired macroscopic properties in cellular materials is a new approach that was recently introduced to the structural engineering mechanics field due to the abundant research into multi-scale modelling. Numerous studies are available on multi-scale analysis in the context of the approximation of an effective elastic constant of heterogeneous media; these studies are based on periodic homogenisation theory (Bensoussan et al. 1978, Sanchez-Palencia 1980, Guedes and Kikuchi 1990), specifically in the scope of fibre-reinforced composites (Terada et al. 2000, Matsuda et al. 2003) and cellular materials, such as honeycomb (Guedes and Kikuchi 1990), plate-fin (Tsuda et al. 2010) and wafer-fin structures (Saha et al. 2007). In addition to periodic microstructure, one study has demonstrated the accuracy of homogenisation theory even for random microstructures by comparison with experimental results from porous media (Takano et al. 2003, 2010). Moreover, the homogenisation method was also widely used in the field of biomechanics for such applications as determining the homogenised elastic constants of cortical (Parnell et al. 2006) and trabecular bones (Hollister et al. 1994). However, these studies, which characterise the mechanical properties of inhomogeneous materials, assume that the cells have a periodically regular array and the mechanical property of a constituent is consistent throughout the volume; or, in short, the microstructures were treated in a deterministic sense. Unfortunately, randomness occurs at the micro-scale level.

It is well understood that the mechanical properties of heterogeneous materials depend on the microscopic properties of their constituents. Because the microscopic properties have been obtained primarily by experiments and the results have been statistically evaluated, the mechanical macroscopic properties demonstrate some variance. The variance in the experimental results is even greater for more complex microstructures, such as bone, in which the Young's modulus was found to be in the range of approximately 1-20 GPa (trabecular) or 5-26 GPa (cortical) (Guo 2001). Random variation in the microscopic properties in engineering materials can result from defects that occur during the fabrication or degradation effects, such as the existence of inclusions and microcracks in the adhesive bonding of multi-joint materials.

Conversely, the dispersion of the macroscopic properties is also tightly linked to the randomness in the geometry of the microstructure. Due to fabrication inaccuracies, most cellular materials have non-periodic structures with practically random spatial arrangements. In some cases, the microstructure, which resembles a corrugated-core sandwich plate, is not necessarily fabricated in a perfectly aligned position, as illustrated in Fig. 1(a). The microstructures may have randomly misaligned layers, as depicted in Fig. 1(b). Matsuda et al. (2011) investigated this problem using deterministic homogenisation theory applied to non-linear time-dependent elastic-viscoplastic materials. The random misalignment of the internal structure was found to affect the viscoplastic properties both macroscopically and microscopically. The degradation mechanism of the component material is also one factor that contributes to the uncertainty of the periodicity in the microstructure. In the case of a honeycomb structure, there are frequent deviations from the regular structure caused by the process in which the individual cells nucleate and grow naturally. Then, new rearrangements take place when the cells impinge on one another. Finally, the cells have a dispersion of size and vary in the number of edges per cell (Gibson and Ashby 1997). Therefore, the uncertainties in the microstructure that affect the macroscopic properties of the heterogeneous materials can be classified into two major factors: microscopic properties and geometrical information.

The randomness and various uncertainty factors that exist in the microstructure demonstrate the necessity of estimating the macroscopic properties in a stochastic nature. A few computational schemes of stochastic homogenisation have been introduced in recent years based on perturbation (Koishi et al. 1996, Kaminski and Kleiber 2000) and spectral (Jardak and Ghanem 2004, Xu and Graham-Brady 2005) methods for specific problems. For instance, Kaminski and Kleiber (1996) used a stochastic finite element perturbation-based method (Kleiber and Tran 1992) to investigate the uncertainties associated with structural interface defects in fibre-reinforced composites by considering the variation in the Young's modulus in a plane stress condition. Xu and Graham-Brady (2005) evaluated the global and local behaviour of random elastic media based on the concept of a stochastic representative volume element (SRVE). Determining the size of the representative volume elements (RVEs) to incorporate the random field into the microstructure of heterogeneous materials (Ostoja-Starzewski 1998, Gitman et al. 2005) is another approach that was introduced to investigate the stochastic response in a multi-scale problem. Most of the methods, however, focus on a matrix-inclusion model, whereas, to our knowledge, no one has performed a stochastic process when considering cellular materials.

In this study, we applied a stochastic homogenisation analysis to predict the dispersion of the homogenised properties of three-dimensional (3D) cellular media in which the microstructure is modelled to have a random variation in the microscopic properties and geometrical information. Our approach is based on the concept of the first-order perturbation proposed by Koishi et al.(1996), which was later developed by Sakata et al.(2008). Koishi et al.(1996) and Sakata et al.(2008) demonstrated the validity of the theoretical formulation by comparing this formulation with a stochastic finite element method and using a Monte Carlo simulation, respectively. However, both researchers tested the numerical algorithm using a fibre-reinforced composite. Therefore, it is critical to adapt the stochastic process considering cellular materials and to incorporate various uncertainty factors into these processes.

2 OUTLINE OF STOCHASTIC HOMOGENISATION USING A FIRST-ORDER PERTURBATION

Consider a unit cell Y representing an inhomogeneous solid material. This cell could contain various types of random microstructures, such as fibres, particles, or polycrystalline or porous materials. The effective elastic properties or homogenised properties of the representative structure can be obtained using classical homogenisation theory. However, because of the random nature in the microstructure, the homogenised properties D^H could be influenced by the uncertain parameters, such as the morphology of the microstructure A, the material types M and the volume fraction V as well as the variables geometrical information X and mechanical properties of constituents D. This relationship can be summarised in eq. (1).

where i is the number of constituent materials and F is a function of D^H. If we apply this method to porous or cellular materials, i in eq. (1) can be neglected. The formulation of the homogenised properties of cellular solids can be generalised in eq. (2).

If we assume that the mechanical properties of constituent D have a small random fluctuation, the stochastic microscopic property response is written as the sum of the deterministic term D* and a stochastic term that is denoted by α.

Next, by taking this fluctuation into account, the homogenised property is formulated as a function of α. If this function is approximated in expanded form, then the approximation of the homogenised property is written as

Applying the first-order perturbation method to this calculation and assuming that the stochastic variable α is normally distributed with a mean value of zero, the expected value (Exp) and variance (Var) of the homogenised property are computed in eq. (5) and (6), respectively (Koishi et al. 1996).

The order of '0' determines the deterministic term, whilst '1' corresponds to the first-order differential for the stochastic variation α at α = 0. cov[α,α] represents the covariance of α. The derivations of the above equations are based on the assumption that only one random variable was considered. However, the present formulation can also be adopted for more than one random variable.

Using the perturbation-based stochastic homogenisation method, the coefficient of variance (COV) should generally be smaller than approximately 0.15 (Kaminski and Kleiber 2000). This result means that the present method is not applicable to problems with great variation in the input variables. Moreover, in the first-order perturbation, the approximation of the variance is largely influenced by the value of the stochastic variable. Considering the fluctuations of the microscopic properties, approximation using a first-order perturbation can result in accurate results for the Young's modulus variation, but estimations using the Poisson's ratio variation require higher order expansions (Sakata et al. 2008). Furthermore, higher order approximation does not always improve the accuracy of the stochastic estimation especially for Young's moduli that exhibit variations (Sakata et al. 2008). Therefore, in this paper, to evaluate the influence of the uncertainties in cellular media microstructure on the dispersion of homogenised properties, we employed a first-order, perturbation-based stochastic homogenisation method.

3 GENERALISED RANDOMNESS PROBLEMS IN CELLULAR SOLID MICROSTRUCTURES

There are two main uncertainty factors that have a major influence on the stochastically homogenised properties of cellular materials, as described by eq. (2). In this paper, we evaluate randomness problems in microstructures that are caused by the uncertainty regarding the mechanical properties of the constituent and the geometrical information. For the first problem, the uncertainties that arise due to microscopic properties were applied to adhesive honeycomb-structured material. Then, in the next problem, the fluctuation in microscopic properties was combined with uncertainty in the geometry due to variation in the periodicity in a corrugated-core plate sandwich. We used a voxel element type to discretise the finite element model in all analyses.

3.1 Fluctuation of the microscopic properties in adhesive materials

Defects within the adhesive materials due to fabrication inaccuracies (i.e., a poor heat treatment process and inclusions of foreign bodies) and degradation or environmental effects (voids, porosities or cracks) normally affect the mechanical properties of bulk adhesive. Consider the periodic microstructure of a stacked-plate aluminium honeycomb, as shown in Fig. 2. The variation in the microscopic properties was assumed to originate in the adhesive joint. The constituent materials of the aluminium and adhesive were applied as an isotropic material. The fluctuation was defined as only existing in the Young's modulus, whilst the Poisson's ratio is deterministic. Therefore, the stochastic response of the Young's modulus E in the adhesive is written as follows:

where E⁰ is the deterministic Young's modulus of the adhesive. The mechanical properties of the adhesive and aluminium are taken as follows: E_adh=2.2 GPa with standard deviation 0.033 GPa, v_adh=0.35, E_alu=70 GPa and v_alu=0.3 (the Young's modulus and the Poisson's ratio, respectively).

3.2 Misalignment of the corrugated-core sandwich plate microstructure

Generally, corrugated-core sandwich plates are fabricated in a randomly aligned position rather than in a perfectly aligned position, as shown in Fig. 1. The red dotted box indicates the unit cell Y. Therefore, when analysing the randomness behaviour in the microstructure, such laminate misalignment should be considered. Accordingly, in this problem, the fluctuation in microscopic properties was combined with a random variation in the misaligned microstructure to obtain an expectation and a dispersion of the homogenised properties in the corrugated-core sandwich plate.

Figure 3(a) illustrates the misaligned position of the corrugated-core unit cell. This process was repeated for N layers in axis-2. Each unit cell with width w has a misalignment l distance in axis-1. Considering the whole substructures, the Y-periodic boundary condition holds in line AB and EF, BC and DE and AD and CF. Then, this periodicity was extended to three dimensions along the axis-3 direction. Let d=l/w be the misalignment ratio for this problem. Although d should be randomly misaligned, we assumed that d was determined by the probability function f(d), as shown in Fig. 3(b). In this function, the probability of d decreased when d increased, and f(d) = 0 at d=0.5. The total probability Pr is the integration of f(d) with respect to d, where it is equal to 1. We take d_i as discrete variables; then, the stochastically homogenised property corresponding to each d is written as D^H_i. Therefore, by considering the probability of d (denoted as Pr_d), the effective stochastically homogenised property D^H(eff.) is computed as follows.

The 3D unit cell was divided into eight node-voxel elements, as shown in Fig. 3(c). A base metal with a corrugated core microstructure was assumed to be an isotropic material with a random variation in the Young's modulus; this is the same formulation described in problem 3.1. To simplify the problem, the Poisson's ratio was set as deterministic. The microscopic material constants used were E=130 GPa with a standard deviation of 1.95 GPa and v = 0.3.

4 COMPUTATIONAL IMPLEMENTATION

Based on deterministic homogenisation theory, the geometrical information X in eq. (2) is replaced by the characteristic displacement χ and is written as

where | Y| is the volume of the unit cell Y. Then, this equation is discretised using finite element methods as

where χ is the solution of the microscopic equation and is written in the following linear algebraic form.

Because the random fluctuations arise in the microscopic property D, the stiffness matrix K and vector F^kl are approximated using a first-order perturbation.

When the random quantities are inserted into K and F^kl in eq. (11), the linear algebraic equation should be rewritten. Therefore, χ is also expressed in an approximate form.

By equating the order of α, the solution of the zeroth and first orders of χ is calculated as

and

Finally, the zeroth and first orders of the stochastic variation of the homogenised properties can be calculated by equating the order of α, as written in eq. (17) and (18), respectively.

Each order of D^H is applied to eq. (5) and (6) to obtain the expected value and the variance of the homogenised property.

5 RESULTS AND DISCUSSION

5.1 Influence of the microscopic property variation

Table 1 shows the results of the expectation and the variance of the homogenised properties of the hexagonal honeycomb structure considering the fluctuation of the Young's modulus in the adhesive material. The greatest expected value was found at D^H ₃₃₃₃. D^H ₁₁₁₁ has the greatest variance. In a first-order perturbation approach, the results are influenced by the stochastic variable α. Next, to evaluate the influence of α on the stochastically homogenised properties, we tested the model with - 0.25< α < 0.25. The relationship of the six diagonal components of the stochastically homogenised property matrix and the stochastic variable α is shown in Fig. 4. Figure 4 confirmed that the relationship between homogenised properties and α in case of microscopic property variation is linear. This result suggests that the first-order perturbation method gives an accurate estimation in the case that the homogenised property is a linear function of the stochastic variable.

Because we used a first-order perturbation, the expected value obtained is equal to the deterministic value of the homogenised property. However, the capability of illustrating the dispersion of the macroscopic property is an advantage of this algorithm. Using a higher order expansion may improve the accuracy of the results for multi-phase materials. However, for porous or cellular materials, the first-order approximation is sufficient for the problem of Young's modulus fluctuation because the higher order of the derivative will result in a microscopic property matrix that equals zero. Even Sakata et al.(2008) demonstrated the accuracy of the first-order expansion of stochastic estimation by comparing this estimation with the Monte-Carlo simulation result for a fibre-reinforced composite and by considering the Young's modulus variation in the fibre or matrix. From these results, it appears fair to claim that the present method is capable of characterising the random variations at a microscopic level without having to use the computationally costly Monte-Carlo simulation.

5.2 Effect of variation in the microstructure periodicity

Figure 5(a-c) shows the effect of the misaligned corrugated-core microstructure along axis-2 on the stochastically homogenised Young's moduli. In this figure, the red line indicates the expected value, whilst the blue dotted line represents the standard deviation. The misaligned position reduced the homogenised property of D^H ₁₁₁₁, D^H ₂₂₂₂ and D^H ₃₃₃₃ at d=0.1. However, the increase of d from 0.2 to 0.4 increased the stiffness of D^H₂₂₂₂ to a value greater than that without misalignment when d reaches 0.3. In other words, these results suggest that the appropriate misalignment in the corrugated-core microstructure has strengthened the homogenised property in axis-2. However, the homogenised properties in D^H₁₁₁₁ and D^H ₃₃₃₃ were not sensitive to the increase in d.

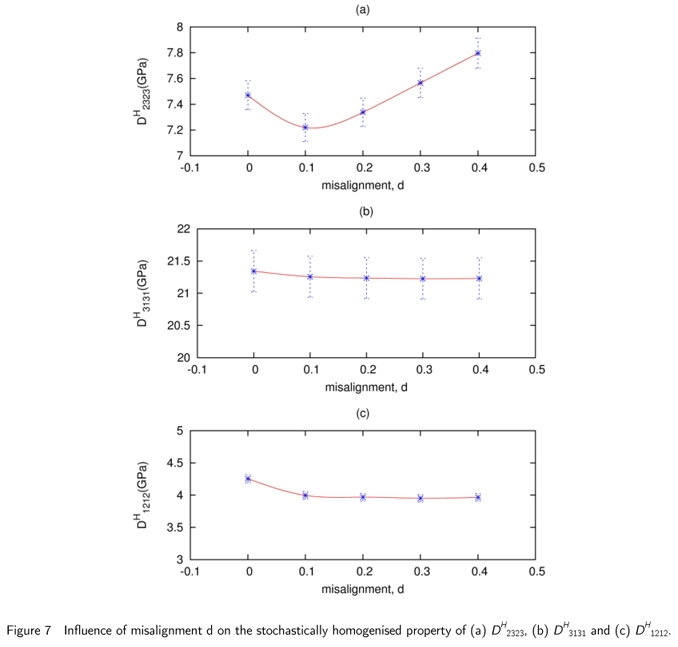
Moreover, the influence of misaligned microstructure on D^H₁₁₂₂, D^H₁₁₃₃ and D^H₂₂₃₃ is shown in Fig. 6(a-c). The figure implies a pattern similar to Fig. 7, in which D^H₁₁₂₂ and D^H₁₁₃₃ were almost not affected over the increment of d, but D^H ₂₂₃₃ increased when d increased. In the same manner, Fig. 7(a-c) shows the effects on D^H₂₃₂₃, D^H₃₁₃₁ and D^H₁₂₁₂. Although D^H₂₃₂₃ shows the same trend as D^H₂₂₂₂ with respect to the d increment, the increasing rate was less than that of the latter. Whilst D^H₃₁₃₁ and D^H₁₂₁₂ appeared almost constant, they were subjected to misalignment d.

Next, to investigate the effect of misalignment as a probability function, we calculated the relative error between the results of d=0 and with probability of d in the expected value and the standard deviation of the homogenised property. Table 2 displays the results of the relative error for the expected value and the standard deviation. The positive value of the percentage implies that the homogenised property was reduced due to misalignment behaviour in the microstructure. The expected value and variance of D^H₁₁₂₂ show the greatest difference compared with other components of the stochastically homogenised property matrix. Obviously, the significant effect of the misalignment appeared in the stochastically homogenised property related to axis-2. It also appears that the stochastically homogenised property in axis-3 has less influence than the property in axis-1. Therefore, by considering the probability of d, the misaligned corrugated-core microstructure reduced the expected value and the variance of homogenised properties.

The homogenised properties without the misalignment behaviour means the variation is only due to random microscopic properties. In order to investigate the influence of random geometry due to misalignment and of random Young's modulus in the homogenised properties, the COV for both conditions was evaluated. It was found that the COV for misalignment variation (0.0154) is slightly higher than that for Young's modulus variation (0.0150). Hence, it proves that the random geometry have much larger influence than the random microscopic properties to the homogenised properties.

6 CONCLUSIONS

To investigate the influence of random variation in the microstructure of a cellular medium, we employed a stochastic multi-scale algorithm using a first-order perturbation that could predict the dispersion of the macroscopic homogenised property. The numerical method was derived based on homogenisation theory and the stochastic finite element method.

Two stochastic problems have been analysed with respect to two major uncertainty factors, which are the microscopic properties and the geometrical information. The fluctuation of the Young's modulus of the adhesive was found to affect the dispersion of the homogenised property of an aluminium-adhesive honeycomb structure, which depends on the existence of the adhesive and its geometry. Next, the uncertainty that arises in the microscopic properties was combined with the varying periodicity of the unit cell in a corrugated-core sandwich plate. The misaligned position in the microstructure was found to have a significant influence on the homogenised property related to axis-2 (misaligned direction). This result suggests that an appropriate misalignment in the microstructure could strengthen the macroscopic homogenised property and the influence of random misalignment to the homogenised properties is much larger than that of random Young's modulus.

Therefore, the results demonstrate the importance of this stochastic multi-scale analysis for the design and fabrication of cellular solids whilst considering microscopic random variations. In this study, more emphasis was put on the uncertainty of the microscopic properties and geometrical information due to the variation in microstructure periodicity. Our future interests involve developing a stochastic process that considers other uncertainty factors in the geometric variation, such as the effects of the unit cell selection and the image-processing technique.

Received in 05 Feb 2013

In revised form 05 Jun 2013

• Bensoussan, A., Lions, J.L., and Papanicolaou, G., 1978. Asymptotic Analysis for Periodic Structures. North-Holland Publishing Company.
• Gibson, L.J. and Ashby, M.F., 1997. Cellular solids: structure and properties. Second. New York: Cambridge University Press.
• Gitman, I.M., Gitman, M.B., and Askes, H., 2005. Quantification of stochastically stable representative volumes for random heterogeneous materials. Archive of Applied Mechanics, 75 (2-3), 79-92.
• Guedes, J. and Kikuchi, N., 1990. Preprocessing and postprocessing for materials based on the homogenization method with adaptive finite element methods. Computer Methods in Applied Mechanics and Engineering, 83 (2), 143-198.
• Guo, X.E., 2001. Mechanical properties of cortical bone and cancellous bone tissue. In: S.C. Cowin, ed. Bone Mechanics Handbook. CRC Press.
• Hollister, S.J., Brennan, J.M., and Kikuchi, N., 1994. A homogenization sampling procedure for calculating trabecular bone effective stiffness and tissue level stress. Journal of Biomechanics, 27 (4), 433-444.
• Jardak, M. and Ghanem, R.G., 2004. Spectral stochastic homogenization of divergence-type PDEs. Computer Methods in Applied Mechanics and Engineering, 193 (6-8), 429-447.
• Kaminski, M. and Kleiber, M., 1996. Stochastic structural interface defects in fiber composites. International Journal of Solids and Structures, 33 (20-22), 3035-3056.
• Kaminski, M. and Kleiber, M., 2000. Perturbation based stochastic finite element method for homogenization of two-phase elastic composites. Computers & Structures, 78 (6), 811-826.
• Kleiber, M. and Tran, D.H., 1992. The stochastic finite element method: basic perturbation technique and computer implementation. Chichester: John Wiley & Sons.
• Koishi, M., Shiratori, M., Miyoshi, T., and Miyano, A., 1996. Stochastic homogenization method for composite materials with uncertain microstructures. Transactions of Japan Society of Mechanical Engineer Series A, 62, 2264-2269.
• Matsuda, T., Kanamaru, S., Yamamoto, N., and Fukuda, Y., 2011. A homogenization theory for elastic-viscoplastic materials with misaligned internal structures. International Journal of Plasticity, 27 (12), 2056-2067.
• Matsuda, T., Ohno, N., Tanaka, H., and Shimizu, T., 2003. Effects of fiber distribution on elastic-viscoplastic behavior of long fiber-reinforced laminates. International Journal of Mechanical Sciences, 45 (10), 1583-1598.
• Ostoja-Starzewski, M., 1998. Random field models of heterogeneous materials. International Journal of Solids and Structures, 35 (19), 2429-2455.
• Parnell, W.J., Grimal, Q., Abrahams, I.D., and Laugier, P., 2006. Modelling cortical bone using the method of asymptotic homogenization. Journal of biomechanics, 39, S20.
• Saha, G.C., Kalamkarov, A.L., and Georgiades, A. V., 2007. Asymptotic homogenization modeling and analysis of effective properties of smart composite reinforced and sandwich shells. International Journal of Mechanical Sciences, 49 (2), 138-150.
• Sakata, S., Ashida, F., Kojima, T., and Zako, M., 2008. Three-dimensional stochastic analysis using a perturbation-based homogenization method for elastic properties of composite material considering microscopic uncertainty. International Journal of Solids and Structures, 45 (3-4), 894-907.
• Sanchez-Palencia, E., 1980. Non-homogeneous media and vibration theory. Springer-Verlag.
• Takano, N., Fukasawa, K., and Nishiyabu, K., 2010. Structural strength prediction for porous titanium based on micro-stress concentration by micro-CT image-based multiscale simulation. International Journal of Solids and Structures, 52 (2), 229-235.
• Takano, N., Zako, M., and Okuno, Y., 2003. Multi-scale finite element analysis of porous materials and components by asymptotic homogenization theory and enhanced mesh superposition method. Modelling and Simulation in Materials Science and Engineering, 11, 137-156.
• Terada, K., Hori, M., Kyoya, T., and Kikuchi, N., 2000. Simulation of the multi-scale convergence in computational homogenization approaches. International Journal of Solids and Structures, 37 (16), 2285-2311.
• Tsuda, M., Takemura, E., Asada, T., Ohno, N., and Igari, T., 2010. Homogenized elastic-viscoplastic behavior of plate-fin structures at high temperatures: Numerical analysis and macroscopic constitutive modeling. International Journal of Mechanical Sciences, 52 (5), 648-656.
• Xu, X.F. and Graham-Brady, L., 2005. A stochastic computational method for evaluation of global and local behavior of random elastic media. Computer Methods in Applied Mechanics and Engineering, 194 (42-44), 4362-4385.

Publication Dates

• Publication in this collection
  03 Feb 2014
• Date of issue
  Oct 2014

History

• Accepted
  05 June 2013
• Received
  05 Feb 2013