Stress effects on the kinetics of hydride formation and growth in metals

Abstract

Although metal hydrides are considered promising candidates for solid-state hydrogen storage, their use for practical applications remains a challenge due to the limitation imposed by the slow kinetics of hydrogen uptake and release, which has driven the interest in using metal nanoparticles as advanced materials of new hydrogen-storage systems since they display fast hydrogenation and dehydrogenation kinetics. Nevertheless, the understanding of the adsorption/release kinetics requires the investigation of the role played by the stress which appears to accommodate the misfit between the metal and hydride phases. In this paper, we present a continuum theory capable of assessing how the misfit stress affects the kinetics of hydride formation and growth in metallic nanoparticles. The theory is then applied to study the kinetics of adsorption/release in spherical particles. This work extends Duda and Tomassetti (2015, 2016) by considering stress-dependent hydrogen mobility.

Keywords
diffusion-induced stress; configurational forces; hydrogen-storage systems; phase transformation

1 INTRODUCTION

When immersed in a hydrogen gas atmosphere, metals such as palladium and magnesium, can soak up hydrogen like a sponge by forming metal hydrides. In fact, as first reported by Graham (1866), ‘’at room temperature and atmospheric pressure, palladium can absorb up to 900 times its own volume of hydrogen. That means, if you were to pump hydrogen into a bottle, it would take enormous pressure to store the same amount easily absorbed in a palladium bed of the same volume” (Wolf and Mansour, 1995). For this reason, metal hydrides are considered promising candidates for solid-state hydrogen storage, although their use for practical applications remains a challenge due to the limitation imposed by the slow kinetics of hydrogen absorption and desorption (Sakintuna et al., (2007), Jain et. al. (2010), Rusman and Dahari (2016)).

The kinetics of hydrogen absorption and desorption by a storage metallic material is the outcome of a sequence many steps. For instance, the steps involved in hydrogen absorption includes: surface adsorption and dissociation of hydrogen molecules, and surface penetration of hydrogen atoms; diffusion of hydrogen atoms within the host metal; phase transformation from a low-hydrogen-content phase ($\alpha$-phase) at low H2 pressures to a high-hydrogen-content phase (hydride phase or $\beta$-phase). Hydrogen desorption occurs in the reverse order. Hence, the resulting kinetics can only be as fast as the slowest step, called the rate-determining step, the identification of which is crucial for understanding and improving the kinetics properties of metal hydrides. The latter has been achieved through several approaches such as catalyzing, compositing, and nanoscaling (Wang et al. (2016)). In particular, the use of metal nanoparticles as advanced materials of new hydrogen-storage systems has been considered as a good alternative since they display fast hydrogenation and dehydrogenation kinetics. However, there is a growing recognition that the performance of these systems can be affected significantly by the strain and stress generated during the processes of hydrogen uptake and release (Baldi et al. (2014) and Narayan et al. (2016)).

It is well known that hydrogen absorption/desorption is accompanied by the generation of stress. In fact, during hydride formation/decomposition, the $\alpha$ and $\beta$ phases are separated by a sharp and coherent interface which results in the generation of stress to accommodate the strain mismatch between the phases. Further, stress is also generated to accommodate inhomogeneous hydrogen-induced lattice expansion during hydrogen diffusion in both phases. It is worth mentioning that the hydrogen-induced lattice expansion is also responsible for relaxation phenomenon known as the “Gorsky Effect” (e.g., Vӧlkl, 1972).

In this paper, we present a continuum theory capable of assessing how the stress affects the kinetics of hydrogen uptake and release in metals under the assumption that the $\alpha -\beta$ transformation is the rate-determining step. This assumption has been discussed in Drozdov et al. (2015a, 2015b), who indicate that the assumption of fast diffusion in both and phases are justifiable. The theory is suitable to describe the behavior of metal-hydrogen systems near the transition chemical potential at which the $\alpha$ and $\beta$ phases would coexist in absence of stress. It is based on the constitutive hypothesis that -in each of the phases- the bulk hydrogen concentration is constant and given by corresponding value in at the transition chemical potential. As Gurtin and Voorhees (1993) observes, this assumption is discussed by Mullins and Sekerka (1963). This work extends the works of Duda and Tomassetti (2015, 2016) by considering stress-dependent hydrogen mobility, an issue whose relevance has been addressed in the literature (e.g., Gronbeck and Zhdanov (2011) and Zhdanov (2010)). As in Gronbeck and Zhdanov (2011), we assume that hydrogen mobility is an increasing function of the mean stress. For related developments, see also Gurtin and Voorhees (1993), Fried and Gurtin (1999) and Feitosa et al. (2015). As in the mentioned works, we restrict our attention to small-strain and isothermal conditions. The resulting quasistatic system of equations form a moving-boundary value problem for diffusion coupled with elasticity. The unknown fields are the displacement and chemical potential, both of which continuous across the moving interface. The resulting free boundary problem is analogous to the so-called quasi-static Stefan problem (e.g., Gurtin (1986)).

The theory is applied to study the kinetics of adsorption/release of hydrogen in a spherical particle, a problem that is amenable to analytical treatment. In this case, the spherical particle is partitioned in the $\alpha$ and $\beta$ phases, separated by a concentric sharp interface, with its inner core occupied by the $\alpha$ ($\beta$) phase during the $\alpha$ to $\beta$ ($\beta$ to $\alpha$) transformation. Hydrogen diffusion takes place in the outer core only. We show that the system displays hysteresis and derive an equation expressing the ratio of the H2 pressures that trigger the $\alpha -\beta$ and $\beta -\alpha$ transformations. This equation can be seen as a generalization of the one obtained in Schwarz and Khachaturyan (2006). We also show that in the course of the $\alpha -\beta$ ($\beta -\alpha$) transformation, hydrogen diffusion slows down (speed up) in the $\beta$ ($\alpha$) phase because that phase is under compressive (tensile) mean stress. Hence, we predict that the presence of stress delays (accelerates) the $\alpha$ to $\beta$ ($\beta$ to$\alpha$) transformation. Most importantly, we provide quantitative results that can be confronted with experimental results to assess the role played by stress on hydrogen uptake and release in single spherical particles. In the case of nanoparticles, these results can be obtained by using nanoplasmonics sensing (see, for instance, Langhammer et. al. (2010) and Syrenova et. al. (2015)). This comparison, however, is outside the scope of this paper.

The paper is organized as follows. In Section 2, we reprise and extend the theory presented in Duda and Tomassetti (2015, 2016) for the problem of the solute-induced diffusion and phase transformation in elastic solids. In Section 3, the general theory is specialized to describe the kinetics of the $\alpha -\beta$ transition in spherical particles. Finally, in Section 4, an analytical study concerning the evolution of the $\alpha -\beta$ interface is presented.

Throughout this paper, the standard notation of continuum mechanics is adopted (Gurtin (1981)) and symbols are defined the first they appear.

2 THE CONTINUUM MODEL

Let ℬ be the reference domain for a solid solution composed of a host elastic solid and an interstitial solute. The domain ℬ is the stage of two interdependent processes taking place at two different scales, namely a macroscopic (mechanical) one due to the deformation of the host solid and a microscopic (chemical) one due to solute diffusion through the interstices of the host solid.

As Figure 1 indicates, ℬ is separated by the moving interface $(t)$ into complementary time-dependent subdomains ${\text{ℬ}}_{\alpha }(t)$ and ${\text{ℬ}}_{\beta }(t)$, occupied by the phases $\alpha$ and $\beta$, respectively.

Figure 1
Two-phase metal-hydride system in a hydrogen gas environment.

Here and henceforth, when writing $f_{\varphi }$ for a given quantity, the index $\varphi$ equals $\alpha$or $\beta$ according whether the quantity is evaluated in subdomains ${\text{ℬ}}_{\alpha }$ and ${\text{ℬ}}_{\beta }$.

The remaining of this section follows closely Gurtin and Voorhees (1993) and Duda and Tomassetti (2015, 2016). See also Fried and Gurtin (1999).

2.1 Bulk equations

We now introduce the field equations of the theory that hold in bulk, it means, away from the interface $$ and the boundary $\partial \text{ℬ}$ of ℬ. These equations, comprised by the force and solute content balances, are given by the local balance relations

$\text{div}T=0and\text{-div}h=\dot{c}$ (1)

where div is the divergence operator, $T$ is the Cauchy stress tensor, $h$ is the solute flux vector, $c$ is the solute content density, and a superposed dot denotes differentiation with respect to time. Notice that body forces, inertia and solute supply have been neglected.

In addition to the aforementioned balances, one should consider as basic an energy imbalance whose local version in bulk admits the representation

$\dot{\omega }-T\cdot \dot{E}+c\dot{\mu }-h\cdot \nabla \mu \le 0$ (2)

where$\omega$is the grand canonical potential density, $E=1/2(\nabla u+\nabla u^T)$ is the infinitesimal strain, given by the symmetric part of the displacement $u$, $\mu$is the chemical potential and $\nabla \mu$ its gradient.

We refer the reader to Fried and Gurtin (1999) for a detailed derivation of the foregoing equations.

2.2 Interface and boundary conditions

We assume that the $\alpha -\beta$ interface is coherent, propagates without dissipation, and that the local chemical equilibrium prevails there. These conditions, together with the force and solute balances localized at the interface, yield the interface conditions of the theory presented in Duda and Tomassetti (2015, 2016).

• Continuity of the displacement and chemical potential

$〚u〛=0,〚\mu 〛=0$ (3)

where $〚A〛=(A_{\alpha }-A_{\beta })$ denotes the jump in a field $A$ across the interface.

• Force and solute content balances

$〚T〛m=0,〚c〛\upsilon -〚h〛\cdot m=0$ (4)

where $\upsilon$ is the velocity of the interface in the direction of the unit normal $m$, which is directed into the phase$\beta$ (see Figure 1).

• Maxwell relation

To describe the motion of the sharp interface an extra condition should be added: the interface motion is dissipationless, this implies that

$〚\omega -(\nabla u)m\cdot Tm〛=0.$ (5)

giving the so-called Maxwell condition.

We consider $\partial \text{ℬ}$ immersed in a reservoir of hydrogen at pressure$p$. Under this assumption the gas pressure can be neglect from the mechanical point of view, and the chemical equilibrium prevails at the metal-gas interface. Thus, we have the mechanical boundary condition

${Tn|}_{\partial \mathcal{B}}=0,$ (6)

where $n$is unit outward normal vector field on $\partial \text{ℬ}$ (see Figure 1). The chemical boundary condition given by

$\mu ={\mu }_{\text{R}}=\frac{1}{2}{\mu }_{{\text{H}}_2}with{\mu }_{{\text{H}}_2}={\mu }_{{\text{H}}_2}^0+k_{B}T\ln \frac{p}{p_0}$ (7)

where ${\mu }_R$is the solute chemical potential in the reservoir, and ${\mu }_{H_2}^0$is the chemical potential of the gaseous hydrogen at the reference pressure $p_0$.

2.3 Constitutive equations

Guided by the dissipation inequality (2), we introduce constitutive equations for $\omega$, $T$, $c$and $h$ in terms of $E$, $\mu$ and $\nabla \mu$. After using the Coleman-Noll procedure, it can be concluded that the conditions must hold (Fried and Gurtin (1999)):

$\omega ={\stackrel{⌢}{\omega }}_{\varphi }(\mu ,E),c=-\frac{\partial {\stackrel{⌢}{\omega }}_{\varphi }(\mu ,E)}{\partial \mu },T=\frac{\partial {\stackrel{⌢}{\omega }}_{\varphi }(\mu ,E)}{\partial E},h=-{\stackrel{⌢}{M}}_{\varphi }(\mu ,E,\nabla \mu )\nabla \mu .$ (8)

The response function ${\stackrel{⌢}{M}}_{\varphi }$is a positive semidefinite tensor-valued function. Therefore from the constitutive point of view, the theory is defined by the response functions ${\stackrel{⌢}{\omega }}_{\varphi }$ and${\stackrel{⌢}{M}}_{\varphi }$, it means, four constitutive functions, two for phase $\alpha$and two for phase $\beta$.

We now present a constitutive specialization suitable for describing situations involving small deviations of the transition chemical potential ${\mu }_{eq}$ at which the phases $\alpha$and $\beta$ would coexist in a stress-free and stable equilibrium, with the corresponding solute content densities given by $c_{\alpha }$and$c_{\beta }$, with $c_{\alpha }>c_{\beta }$ (see Figure 1).

We assume the additive decomposition of the total strain $E$ into its elastic part $E_e$and its stress-free part $E_0$, at phase $\varphi$

$E=E_e+E_{0\varphi },E_{0\varphi }={\widehat{ϵ}}_{\varphi }(c_{\varphi })I$ (9)

observes that $E_{0\varphi }$has a purely dilatational form. We also assume that

${\widehat{ϵ}}_{\alpha }(c_{\alpha })={\eta }_{\alpha }(c_{\alpha }-c_{0\alpha })and{\widehat{ϵ}}_{\beta }(c_{\beta })={\eta }_{\beta }(c_{\beta }-c_{0\beta })+e_T$ (10)

where $e_T$is the transformation strain associated with the possibility of structural transformation from the$\alpha$-phase to the $\beta$-phase, $c_{0\varphi }$ is a reference value of the solute content in the $\varphi$-phase for measuring the strain, ${\eta }_{\varphi }$is a material parameter. We define the misfit strain between the $\beta$ and $\alpha$ phases

${ϵ}_0={\widehat{ϵ}}_{\beta }(c_{\beta })-{\widehat{ϵ}}_{\alpha }(c_{\alpha })$ (11)

The constitutive response for the grand canonical potential is assumed to have the form

${\omega }_{\varphi }(\mu ,E)=-c_{\varphi }(\mu -{\mu }_{eq})+\frac{\lambda }{2}(\text{tr}{E}_e){}^2+G{\left|E_e\right|}^2$ (12)

where $\lambda$ and $G$ represent the Lame´ elastic moduli, assumed be the same for both phases.

From (8)_2,3,4, the response function ${\stackrel{⌢}{M}}_{\varphi }$ is allowed to depend on the stress $T$ and solute content $c$. Therefore, we consider the isotropic specialization given by

${\stackrel{⌢}{M}}_{\varphi }(c_{\varphi },T)=M_{\varphi }{c}_{\varphi }Iwith{M}_{\varphi }=m_{\varphi }{f}_{\varphi }(\sigma )$ (13)

where $m_{\varphi }$ is the stress-free solute mobility, and $f_{\varphi }(\sigma )>0$ accounts for the stress effect on the solute mobility $M_{\varphi }$through the mean stress $\sigma =trT/3$, where $trT$ is the trace of the stress tensor $T$. For instance, if one accounts for the stress-dependency of the activation energy for diffusion, it can be shown that $f_{\varphi }(\sigma )$ can be written as (Gronbeck and Zhdanov, 2011).

$f_{\varphi }(\sigma )=e^{-{\gamma }_{\varphi }\sigma }$ (14)

where ${\gamma }_{\varphi }\ge 0$ describes the effect of the stress on the hydrogen mobility. For ${\gamma }_{\varphi }>0$ notice that the hydrogen mobility increases with the mean stress $\sigma$.

From (8)2 and (12) we have

$c=\{\begin{array}{l}{c}_{\alpha }\text{in}{\mathcal{B}}_{\alpha }(t),\\ c_{\beta }\text{in}{\mathcal{B}}_{\beta }(t),\end{array}$ (15)

thereupon the solute content density $c$ is a piecewise-homogeneous field, and hence $\dot{c}=0$ in bulk. From (8)₃ and (12) the stress tensor $T$ is given by

$T=\lambda (\text{tr}{E}_e)I+2G{E}_e,$ (16)

with

$E_e=E-\{\begin{array}{l}{\widehat{ϵ}}_{\alpha }(c_{\alpha })Iin{\mathcal{B}}_{\alpha }(t),\\ {\widehat{ϵ}}_{\beta }(c_{\beta })Iin{\mathcal{B}}_{\beta }(t).\end{array}$ (17)

From (8)₄ and (13) the solute flux $h$is given by

$h=\{\begin{array}{l}-m_{\alpha }{f}_{\alpha }(\sigma )c_{\alpha }\nabla \mu \text{in}{\mathcal{B}}_{\alpha }(t),\\ -m_{\beta }{f}_{\beta }(\sigma )c_{\beta }\nabla \mu \text{in}{\mathcal{B}}_{\beta }(t).\end{array}$ (18)

A final remark concerning the determination $c_{\alpha }$, $c_{\beta }$ and ${\mu }_{eq}$ is in order. Indeed, classical thermodynamics arguments can be invoked to show that these quantities can be obtained by solving the set of equations

$\stackrel{⌢}{\psi }\text{'}(c_{\alpha })=\stackrel{⌢}{\psi }\text{'}(c_{\beta }),\stackrel{⌢}{\psi }\text{'}(c_{\alpha })=\frac{\stackrel{⌢}{\psi }(c_{\alpha })-\stackrel{⌢}{\psi }(c_{\beta })}{c_{\alpha }-c_{\beta }},{\mu }_{eq}=\stackrel{⌢}{\psi }\text{'}(c_{\beta }),$ (19)

where $\stackrel{⌢}{\psi }$ is the free-energy response in absence of stress. The free-energy response can be obtained by using statistical mechanical methods. See, for instance, Ledovskikh et al. (2006) and references cited therein. Notice that the statistical mechanical treatment for metal-hydrogen systems undergoing phase transition was proposed by Lacher (1937).

2.4 Summary of the governing equations

We now summarize the governing equations of the theory under consideration for the moving boundary-initial value problem. The unknown quantities are: $u(x,t)$, $\mu (x,t)$ and $(t)$

• Bulk equations

$\text{div}T=0,T=\lambda (\text{tr}{E}_e)I+2G{E}_e,E_e=E-{\widehat{ϵ}}_{\varphi }(c_{\varphi })I,E=(\nabla u+\nabla u^T)\text{/}\mathrm{2,}E=(\nabla u+\nabla u^T)\text{/}\mathrm{2,}\text{div}h=0,\text{div}h=0,h=-m\nabla \mu ,m=m_{\varphi }{f}_{\varphi }(\sigma )c_{\varphi },\sigma =(trT)\text{/}\mathrm{3,}$ (20)

with $f_{\varphi }$ given by (14);

• Interface conditions

$\begin{array}{l}〚Tm〛m=\mathrm{0,}(c_{\alpha }-c_{\beta })v=〚h〛\cdot m,\\ 〚u〛=\mathrm{0,}〚\mu 〛=\mathrm{0,}\\ 〚\omega -\left(\nabla u\right)m\cdot Tm〛=\mathrm{0,}\end{array}$ (21)

with $\omega$ given by (12);

• Boundary conditions

$Tn=0,\mu ={\mu }_R$ (22)

Notice that the problem described by equations (20) - (22) is a “Stefan Problem” (e.g. Vuik (1993)) due to the presence of a phase boundary that can move with time. In this case, the so-called Stefan condition is provided by (21)3.

3 SPHERICAL GEOMETRY

In this section, we specialize and solve the equations for spherical particles. In this case, it is worth mentioning that the equations describing the lattice strain in the core-shell particle were earlier derived by other authors. See, e.g., a brief review in the Supporting Information for and Syrenova et. al. (2015).

Let us now consider that $\text{ℬ}$is a solid sphere of radius $R$, divided into two phases $\alpha$ and $\beta$, by a concentric sharp interface $(t)$ of radius $\rho (t)$. The inner region is occupied by the $\alpha$-phase, while the outer region is occupied by the $\beta$-phase

$\text{ℬ}=\{x:|x|\le R\},{\text{ℬ}}_{\alpha }=\{x:0\le |x|<\rho (t)\},{\text{ℬ}}_{\beta }=\{x:\rho (t)<|x|\le R\}.$ (23)

The concentric sharp interface and the normal velocity are

$(t)=\{x:|x|=\rho (t)\},\dot{\rho }(t)=v.$ (24)

The stress, strain and chemical potential fields are obtained from the knowledge of the current position of the interface as will be discussed below. Thanks to spherical symmetry of the problem

$u(x)=u(r)e,e=\frac{x}{\left|x\right|},\mu (x)=\mu (r),r=\left|x\right|$ (25)

and

$E=u\text{'}e\otimes e+\frac{u}{r}(I-e\otimes e),T={\sigma }_{r}e\otimes e+{\sigma }_{\theta }(I-e\otimes e)$ (26)

where $a\text{'}$ denotes $da\text{/}dr$.

According to the equations (16), (17) and (26), the stress components are then given by

$\begin{array}{l}{\sigma }_r{(}_r=\lambda \left(u\text{'}(r)+\frac{2u(r)}{r}\right)+2Gu\text{'}(r)-(3\lambda +2G){\widehat{ϵ}}_{\varphi }(c_{\varphi }),\\ {\sigma }_{\theta }{(}_r=\lambda \left(u\text{'}(r)+\frac{2u(r)}{r}\right)+2G\frac{u(r)}{r}-(3\lambda +2G){\widehat{ϵ}}_{\varphi }(c_{\varphi }),\\ trT{(}_r={\sigma }_r{(}_r+2{\sigma }_{\theta }{(}_r=(3\lambda +2G)\left(u\text{'}(r)+2\frac{u(r)}{r}-{\widehat{ϵ}}_{\varphi }(c_{\varphi })\right).\end{array}$ (27)

Taking (27) into account, the force balance described in (1)1 can be rewritten as

$\frac{d}{dr}\left(\frac{1}{r^2}\frac{d}{dr}(r^{2}u(r))\right)=\mathrm{0,}$ (28)

which general solution is given by

$u(r)=\{\begin{array}{l}{C}_1^{\alpha }rfor0\le r\le \rho ,\\ C_1^{\beta }r+\frac{C_2^{\beta }}{r^2}for\rho \le r\le R.\end{array}$ (29)

From conditions (3)₁, (4)₁ and (6), we have

$\begin{array}{l}{C}_1^{\alpha }={\widehat{ϵ}}_{\alpha }(c_{\alpha })-\frac{{ϵ}_0}{3(2G+\lambda )}\left(3\kappa +4G{\left(\frac{\rho }{R}\right)}^3\right),\\ C_1^{\beta }={\widehat{ϵ}}_{\beta }(c_{\beta })-\frac{4G{ϵ}_0}{3(2G+\lambda )}{\left(\frac{\rho }{R}\right)}^3,\\ C_2^{\beta }=-\frac{\kappa {ϵ}_0{\rho }^3}{(2G+\lambda )}.\end{array}$ (30)

where $3\kappa =2G+3\lambda$is the bulk modulus.

We now consider the determination of the chemical potential. From (27)3 and (29), it follows that $trT$ is piecewise homogeneous. Hence, after using (20), it follows that $\mu$is harmonic in bulk. This implies that $\mu (r)$ satisfies the equation

$\frac{d}{dr}\left(r^2\frac{d}{dr}\mu (r)\right)=\mathrm{0,}$ (31)

the general solution of which is given by

$\mu (r)=\{\begin{array}{l}{D}_1^{\alpha }for0\le r\le \rho ,\\ D_1^{\beta }r+\frac{D_2^{\beta }}{r}for\rho \le r\le R.\end{array}$ (32)

From conditions (3)₂, (7)₁ and (5), we have

$D_1^{\alpha }={\mu }_{\rho },D_1^{\beta }={\mu }_R-\left(\frac{\rho }{R-\rho }\right)({\mu }_{\rho }-{\mu }_R),D_2^{\beta }=\left(\frac{\rho R}{R-\rho }\right)({\mu }_{\rho }-{\mu }_R)$ (33)

where

${\mu }_{\rho }=\mu (\rho )={\mu }_{eq}+{\mu }_0\left(2{\left(\frac{\rho }{R}\right)}^3-1\right),{\mu }_0=\frac{6G\kappa {ϵ}_0{}^2}{(2G+\lambda )(c_{\beta }-c_{\alpha })}$ (34)

Notice that (32) and (33) imply that

$\mu \text{'}(r)=\{\begin{array}{l}0for0\le r\le \rho ,\\ \left(\frac{{\mu }_R-{\mu }_{\rho }}{R-\rho }\right)\frac{\rho R}{r^2}for\rho \le r\le R.\end{array}$ (35)

4 THE EVOLUTION EQUATION OF THE INTERFACE

We now obtain the evolution equation of the interface. From (4)₂, (18) and (34) we have

$\dot{\rho }(t)=-\frac{m_{\beta }{f}_{\beta }(\sigma )c_{\beta }}{c_{\beta }-c_{\alpha }}\left(\frac{{\mu }_R-{\mu }_{\rho }}{R-\rho }\right)\frac{R}{\rho }$ (36)

with ${\mu }_0$ and ${\mu }_{\rho }$ given by (34). The equation (36) has been obtained by assuming that $\alpha$-phase occupies the inner core of the sphere. Otherwise, when the $\beta$-phase occupies the inner core, $c_{\alpha }$($c_{\beta }$) must be replaced by $c_{\beta }$ ($c_{\alpha }$), and $m_{\beta }{f}_{\beta }$ by$m_{\alpha }{f}_{\alpha }$.

After using (14), (27)₃, (29), and (30), we rewrite equation (36) as

$\tau (1-y)y\dot{y}=\exp (A{y}^3)\left(B(2y^3-1)-1\right)$ (37)

where

$y=\frac{\rho }{R},\tau =\left(\frac{c_{\beta }-c_{\alpha }}{c_{\beta }}\right)\frac{R^2}{m_{\beta }({\mu }_{eq}-{\mu }_R)},A=\left(\frac{4\kappa G{ϵ}_0}{2G+\lambda }\right){\gamma }_{\beta },B=\frac{{\mu }_0}{{\mu }_R-{\mu }_{eq}},$ (38)

with ${\mu }_0$ defined in (34). Notice that $\tau$ is a characteristic time.

We are looking for solutions of (37) such that $y(0)=1$and $\dot{y}<0$. This situation describes the beginning of the process which the sphere is in the $\alpha$-phase. The $\beta$-phase nucleates at the boundary and the interface moves toward the interior of the sphere. We refer to this process as the $\alpha \to \beta$ transformation, or process of absorption.

When the specimen is saturated at the beginning of the process, i.e., $y(0)=0$, that means all the sphere is in the $\beta$-phase. If the process continues, i.e., $\dot{y}>0$, the $\alpha$-phase nucleates at the boundary. We denote this process as the $\beta \to \alpha$ transformation, or process of desorption.

We now use equation (37) to determine two salient features of the $\alpha \to \beta$($\beta \to \alpha$) transformation, namely the necessary condition for its occurrence and the time needed for its completion. We begin by noticing that if the solid sphere is initially in the single $\alpha$-phase:

• It remains in the single $\alpha$-phase if

${\mu }_R<{\mu }_R{}^{\alpha \to \beta }:={\mu }_{eq}+{\mu }_0$ (39)

• The $\alpha \to \beta$transformation initiates when

${\mu }_R={\mu }_R{}^{\alpha \to \beta }:={\mu }_{eq}+{\mu }_0$ (40)

On the other hand, if the solid sphere is initially in the single $\beta$-phase:

• It remains in the single $\beta$-phase if

${\mu }_R>{\mu }_R{}^{\beta \to \alpha }:={\mu }_{eq}-{\mu }_0,$ (41)

• The $\beta \to \alpha$ transformation triggered when

${\mu }_R={\mu }_R{}^{\beta \to \alpha }:={\mu }_{eq}-{\mu }_0,$ (42)

Therefore, the absorption/desorption cycle displays a hysteresis, with loop amplitude given by

${\mu }_R{}^{\alpha \to \beta }-{\mu }_R{}^{\beta \to \alpha }=2{\mu }_0=\frac{12G\kappa {ϵ}_0{}^2}{(2G+\lambda )(c_{\beta }-c_{\alpha })}=\frac{4G(1+\nu ){ϵ}_0{}^2}{(1-\nu )(c_{\beta }-c_{\alpha })},$ (43)

where $\nu =\lambda /(\lambda +2G)$ is the Poisson’s ratio. Taking (7) into account, this expression can be written as the following ratio of the gas pressures $p^{\alpha \to \beta }$ and $p^{\beta \to \alpha }$needed to trigger the $\alpha \to \beta$ and $\beta \to \alpha$ transformations

$k_{b}T\ln \left(\frac{p^{\alpha \to \beta }}{p^{\beta \to \alpha }}\right)=4{\mu }_0=\frac{8G(1+\nu ){ϵ}_0{}^2}{(1-\nu )(c_{\beta }-c_{\alpha })}$ (44)

It is worth noticing that for situations in which ${\eta }_{\alpha }={\eta }_{\beta }=:\eta$, $c_{0\alpha }=c_{0\beta }=0$, and$e_T=0$, the foregoing equation simplifies to

$k_{b}T\ln \left(\frac{p^{\alpha \to \beta }}{p^{\beta \to \alpha }}\right)=\frac{8G(1+\nu ){\eta }^2(c_{\beta }-c_{\alpha })}{(1-\nu )}$ (45)

which is identical to the equation obtained by Schwarz and Khachaturyan (2006). Notice that these authors measure solute content in number of solute atoms per interstitial sites, whereas here we use solute number density.

We now derive expressions for the times ${\Gamma }_{\alpha \to \beta }$ and ${\Gamma }_{\beta \to \alpha }$ required for the $\alpha \to \beta$ and $\beta \to \alpha$ transformations to occur. After defining

$f(x)=\frac{(1-x)x}{\exp (A{x}^3)(B(2x^3-1)-1)}$ (46)

and using (37), it follows that ${\Gamma }_{\alpha \to \beta }$ and ${\Gamma }_{\beta \to \alpha }$ are given by

${\Gamma }_{\alpha \to \beta }(y)=\tau |{\displaystyle {\int }_y^{1}f}(x)dx|and{\Gamma }_{\beta \to \alpha }(y)=\tau |{\displaystyle {\int }_0^{y}f}(x)dx|$ (47)

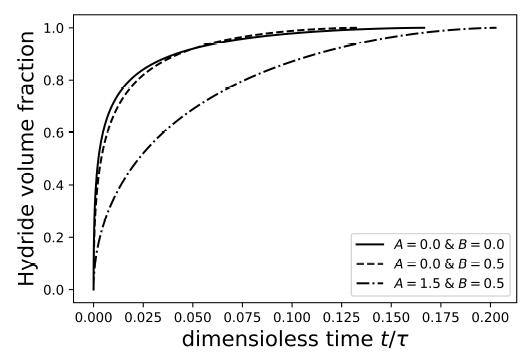
Figure 2 depicts the time dependence of the volume fraction of hydride phase

$\frac{(1-y^3)c_{\beta }}{y^3{c}_{\alpha }+(1-y^3)c_{\beta }}$ (48)

during the $\alpha$ to $\beta$ transformation for $c_{\beta }/c_{\alpha }=4.$ and different values of $A$and $B$. When the mobility is not affected by stress ($A=0$), one can see that stress accelerates the $\alpha$ to $\beta$ transformation. Then, the transformation time is shorter when the role played by stress is accounted for. However, when a stress-dependent mobility is considered, the $\alpha$ to $\beta$ transformation slows down and the corresponding transformation time longer when compared to the case in which the mobility is not affected by stress. Therefore, according to the simple model presented in this paper, stress affect the kinetics of the $\alpha$ to $\beta$transformation in two opposite ways, speeding up and slowing down the phase transformation. These results are presented for illustrative purposes only.

Figure 2
Time evolution of the hydride volume fraction for $c_{\beta }/c_{\alpha }=4.$ and distinct values of A and B.

5 CONCLUSIONS

A continuum theory aimed at describing stress effects on the kinetics of hydrogen uptake and release in metals was presented in this paper. The theory was built upon on the following simplifying assumptions: the $\alpha \text{/}\beta$($\beta \text{/}\alpha$) transformation is the rate-determining step in the absorption (desorption) of hydrogen; the deviation of the hydrogen chemical potential from the transition chemical potential at which the $\alpha$ and $\beta$ phases would coexist in absence of stress is small; in each of the phases, the bulk hydrogen concentration is constant and given by the corresponding value in at the transition chemical potential. As an application of the theory, an analytical treatment was provided to study the kinetics of adsorption/release of hydrogen in a spherical particle. It was shown that the system displays hysteresis and an equation expressing the ratio of the ${\text{H}}_2$pressures that trigger the $\alpha \text{/}\beta$ and $\beta \text{/}\alpha$ transformations was derived. Further, it was show that in the course of the $\alpha \text{/}\beta$($\beta \text{/}\alpha$) transformation, hydrogen diffusion slows down (speed up) in the $\beta$($\alpha$) phase because that phase is under compressive (tensile) mean stress. Hence, it was predicted that the presence of stress delays (accelerates) the $\alpha$ to $\beta$ ($\beta$ to $\alpha$) transformation. To deal with more general geometries and conditions, we believe that a phase-field model of the Cahn-Hilliard type would provide an interesting avenue for exploration. Such a model could be calibrated with the analytical model presented here.

Acknowledgements

Fernando P. Duda gratefully acknowledges financial support by CNPq and FAPERJ. Angela C. Souza would like to point out that this work has been carried out despite the economic difficulties of the author’s country. The authors gratefully thank the referees for their constructive comments which helped improving this paper.

References

• Baldi A. et al. (2014). In situ detection of hydrogen-induced phase transitions in individual palladium nanocrystals. Nature Materials, 13: 1143-1148.
• Duda, F.P. and Tomassetti, G. (2015). Stress effects on the kinetics of hydrogen adsorption in a spherical particle: an analytical model. Int. J. Hydrogen Energy, 40: 17009-17016.
• Duda, F.P. and Tomassetti, G. (2016). On the e_ect of elastic distortions on the kinetics of diffusion-induced phase transformations. J. Elasticity, 122: 179-195.
• Drozdov I.V. et al. (2015a). Modelling and proper evaluation of volumetric kinetics of hydrogen desorption by metal hydrides. Int. J. Hydrogen Energy, 40: 10111-10122.
• Drozdov, I.V., Vaßen, R., & Stöver, D. (2015b). Modelling and evaluation of hydrogen desorption kinetics controlled by surface reaction and bulk diffusion for magnesium hydride. RSC Advances, 5(7), 5363-5371.
• Feitosa, J.L.C. et al. (2015). Stress effects on hydrogen permeation through tubular multilayer membranes: modeling and simulation. Int. J. Hydrogen Energy, 40: 17031-17037.
• Fried, E. and Gurtin, M. (1999). Coherent solid-state phase transitions with atomic diffusion: a thermomechanical treatment. J. Statistical Physics, 95: 1361-1427.
• Graham, T. (1866). XVIII. On the absorption and dialytic separation of gases by colloid septa. Philosophical transactions of the Royal Society of London, 156, 399-439.
• Gronbeck, H. and Zhdanov, V.P. (2011). Effect of lattice strain on hydrogen diffusion in Pd: a density functional theory study. Physical Review B, 84: 0523011-0523014.
• Gurtin, M. (1981). An Introduction to Continuum Mechanics, Academic Press.
• Gurtin, M. E., and Voorhees, P. W. (1993). The continuum mechanics of coherent two-phase elastic solids with mass transport. Proc. R. Soc. Lond. A 440 (1909): 323-343.
• Gurtin, M. E. (1986). On the two-phase Stefan problem with interfacial energy and entropy. Archive for Rational Mechanics and Analysis, 96(3), 199-241.
• Jain, I. P., Lal, C., and Jain, A. (2010). Hydrogen storage in Mg: a most promising material. International Journal of Hydrogen Energy, 35(10), 5133-5144.
• Lacher, J.R. (1937). A theoretical formula for the solubility of hydrogen in Palladium. Proc. Royal Society A, 161: 525-545.
• Langhammer, C. et al. (2010). Size-dependent kinetics of hydriding and dehydriding of Pd nanoparticles. Physical Review Letters, 104: 135502.
• Ledovskikh, A., Danilov, D., Rey, W. J. J., and Notten, P. H. L. (2006). Modeling of hydrogen storage in hydride-forming materials: statistical thermodynamics. Physical Review B, 73(1), 014106.
• Mullins, W. W., and Sekerka, R. F. (1963). Morphological stability of a particle growing by diffusion or heat flow. Journal of applied physics, 34(2), 323-329.
• Narayan, T.C. et al. (2016). Reconstructing solute-induced phase transformations within individual nanocrystals. Nature Materials, 15: 786-784.
• Rusman, N. A. A., and Dahari, M. (2016). A review on the current progress of metal hydrides material for solid-state hydrogen storage applications. International Journal of Hydrogen Energy, 41(28), 12108-12126.
• Schwarz, R.B. and Khachaturyan, A.G. (2006). Thermodynamics of open two-phase systems with coherent interfaces: application to metal-hydrogen systems. Acta Materialia, 54: 313-323.
• Sakintuna, B., Lamari-Darkrim, F., and Hirscher, M. (2007). Metal hydride materials for solid hydrogen storage: a review. International journal of hydrogen energy, 32(9), 1121-1140.
• Syrenova S. et al. (2015). Hydride formation thermodynamics and hysteresis in individual Pd nanocrystals with different size and shape. Nature Materials, 14: 1236{1244.
• Vuik C. (1993). Some historical notes about the Stefan problem, Nieuw Archief voor Wiskunde, 11: 157-167.
• Vӧlkl J. (1972). The Gorsky E_ect. Berichte der Bunsengesellschaft fur physikalische Chemie, 76:797-805.
• Wang, H., Lin, H. J., Cai, W. T., Ouyang, L. Z., & Zhu, M. (2016). Tuning kinetics and thermodynamics of hydrogen storage in light metal element based systems-a review of recent progress. Journal of Alloys and Compounds, 658, 280-300.
• Wolf R. and Mansour K. (1995). The amazing metal sponge: soaking up hydrogen. The Projects in Scientific Computing Archive. www.psc.edu/science/Wolf/Wolf.html
  » www.psc.edu/science/Wolf/Wolf.html
• Zhdanov V.P. (2010). Effect of lattice strain on the kinetics of hydride formation in metal nanoparticles. Chemical Physics Letters, 492: 77-81.

• Available Online: April 03, 2018

Publication Dates

• Publication in this collection
  2018

History

• Received
  30 July 2017
• Reviewed
  24 Feb 2018
• Accepted
  21 Mar 2018