An elementary proof of MinVol(Rn) = 0 for n > 3

Abstracts

In this paper, we give an elementary proof of the result that the minimal volumes of R³ and R4 are zero. The approach is to construct a sequence of explicit complete metrics on them such that the sectional curvatures are bounded in absolute value by 1 and the volumes tend to zero. As a direct consequence, we get that MinVol (Rn) = 0 for n > 3.

minimal volume; smooth gluing; bounded geometry

Neste artigo fornecemos uma demonstração elementar do resultado de que os volumes minimais de R³ e R4 são ambos iguais a zero. A abordagem consiste na construção de uma seqüência de métricas completas explícitas nesses espaços cujas curvaturas seccionais são limitadas em valor absoluto por 1 e os volumes tendem a zero. Como conseqüência direta, estabelecemos que MinVol(Rn) = 0 para n > 3.

volume mínimo; colagem diferenciável; geometria

MATHEMATICAL SCIENCES

An elementary proof of MinVol(Rⁿ) = 0 for n > 3

Jiaqiang Mei^I; Hongyu Wang^II; Haifeng Xu^II

^IDepartment of Mathematics, Nanjing University, 210093 Nanjing, Jiangsu, P.R. China

^IISchool of Mathematical Science, Yangzhou University, 225009 Yangzhou, Jiangsu, P.R. China

^{Correspondence to}

ABSTRACT

In this paper, we give an elementary proof of the result that the minimal volumes of R³ and R⁴ are zero. The approach is to construct a sequence of explicit complete metrics on them such that the sectional curvatures are bounded in absolute value by 1 and the volumes tend to zero. As a direct consequence, we get that MinVol (Rⁿ) = 0 for n > 3.

Key words: minimal volume, smooth gluing, bounded geometry

RESUMO

Neste artigo fornecemos uma demonstração elementar do resultado de que os volumes minimais de R³ e R⁴ são ambos iguais a zero. A abordagem consiste na construção de uma seqüência de métricas completas explícitas nesses espaços cujas curvaturas seccionais são limitadas em valor absoluto por 1 e os volumes tendem a zero. Como conseqüência direta, estabelecemos que MinVol(Rⁿ) = 0 para n > 3.

Palavras-chave: volume mínimo, colagem diferenciável, geometria.

1 INTRODUCTION

The definition of minimal volume of a C^∞ manifold M (without boundary) was first introduced by Gromov (Gromov 1982). Denote (M) the set of all complete smooth Riemannian metrics on M such that the corresponding sectional curvatures are bounded in absolute value by 1. We say that (M, g) has bounded geometry (Cheeger and Gromov 1985) if its metric belongs to (M). The minimal volume of M is a geometric invariant which is defined as For closed surfaces M, by Gauss-Bonnet formula, it's easy to see that

For closed surfaces M, by Gauss-Bonnet formula, it's easy to see that

MinVol(M) = 2π |χ (M)|.

Thus the minimal volume of a closed surface is actually a topological invariant. For the two dimensional plane, Gromov (Gromov 1982) obtained the following estimate

MinVol(

²) < (2 + 2)π.

Bavard and Pansu proved that this is an equality (Bavard and Pansu 1986). B.H. Bowditch (Bowditch 1993) gave a different proof by using spherical isoperimetric inequality. Gromov had shown that MinVol(

ⁿ) = 0 without going into details in (Gromov 1982, Appendix 2). Cheeger and Gromov (Cheeger and Gromov 1985, Example 1.6) showed that ⁿ(n > 4) has a similar solid torus decomposition as ³. Thus ⁿ admits a family of metrics such that the sectional curvatures are bounded and the volumes tend to zero. In this paper, we give a detailed proof of the result in another direct way by constructing the explicit metrics which are different from those in (Cheeger and Gromov 1985, Example 1.6) on the higher dimensional Euclidean spaces.

We state a few results about minimal volume. As stated in (Paternain and Petean 2003), the minimal volume does depend on the smooth structure of the manifold (also see (Bessières 1998)). J. Cheeger and M. Gromov introduced in (Cheeger and Gromov 1986, Gromov 1982) the concepts of F-structure and T-structure and obtained some results about F-structure and minimal volume. They proved that if M admits a polarized F-structure then the minimal volume of M vanishes. Notice that there is a little difference between the original definition of T-structure given in (Gromov 1982) and the later definition given in (Cheeger and Gromov 1986, Paternain and Petean 2003). The graph manifold is a 3-manifold which admit a polarized T-structure. So graph manifold is a special T-manifold. Thus the minimal volume of graph manifold is zero. Furthermore, T. Soma proved in (Soma 1981) that the connected sum of two graph manifold is still a graph manifold. In (Gromov 1982) Gromov pointed out that this result holds for odd dimensional manifolds with T-structures. Paternain and Petean proved in (Paternain and Petean 2003) that the result also holds for the family of manifolds which admit general T-structures and for any dimension greater than 2. The minimal volume is closely related to the collapsing theory in Riemannian geometry. Cheeger, Fukaya and Gromov have developed collapsing theory, and they obtained many important results (Cheeger and Gromov 1986, 1990, Fukaya 1990).

The organization of this paper is as follows: In Section 2, we discuss how to realize smooth gluing of metrics on 2-dimensional surfaces and to construct metrics on Y-pieces (Buser 1992) by a simple method. This method is intuitive even without using uniformization theorem. The "Y-piece" (also called "pair of pants") means a compact topological surface obtained from a 2 dimensional sphere by cutting away the interior of 3 disjoint closed topological disks. In Section 3, we give an explicit construction of a sequence of complete metrics on

³ with bounded curvatures such that the corresponding volumes tend to zero.We just take product metric on Y-piece × S¹ and equip the metric on each piece. So we didn't change the topology of ³. We also apply the similar construction to ⁴. As an immediate corollary, we have MinVol(ⁿ) = 0, for n > 3.

2 CONSTRUCTION OF METRICS ON Y-PIECES

Our goal is going to construct metrics on Y-piece with uniformly bounded curvatures which are independent of the lengthes of the boundary. In order to realize smooth gluing of metrics, we simply require that the metrics on a small tubular neighborhood of the boundary of such Y-piece are product metrics.

To do this, it is sufficient to construct metrics on a disk. A simple way is to glue S² \ D² with a cylinder S¹ × I along the circle (boundary). By viewing S² \ D² and cylinder as surfaces of revolution, what remains to do is to consider smooth gluing of the images of functions and calculate the Gauss curvature of surface of revolution. But here the radius of S¹ must be very small (e.g. ε) for our purpose. Hence, to maintain the curvatures of the surface in [-1, +1], we must insert a good surface. Here we choose a part of pseudo sphere. Then, we prove that the surface after gluing is still smooth, the curvature is uniformly bounded (i.e. independent of ε), and the volume changes a little.

We first use the mollifier to construct a cut-off function (Lemma 2.1). Using this lemma, we can glue smoothly two functions which are tangent at a point (Lemma 2.2). By a result of Dan Henry, we construct a better cut-off function (see Lemma 2.5).

These results are used to construct metrics on disk D² such that the metrics have bounded curvatures and the metrics when restricted in a small neighborhood of ∂ D² are product metrics. See Lemma 2.6 and 2.8.

LEMMA 2.1. There is a smooth function f(x) ∈ [0, b], b > 0 on , such that

and

PROOF. Let

where 0 < δ < . Set

where j(x) is the mollifier function defined on by

and A is equal to

dx, so that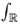 j(x)dx = 1. For any δ > 0, let

Then by calculation, we get

Hence, ø_δ(x) (simply denoted by ø(x)) is the required function.

Moreover, we have

□

since Ae > 1.

LEMMA 2.2. Suppose f(x), g(x) are two smooth functions defined on

and satisfy

f(c) = g(c) and f'(c) = g'(c)

at some point x = c ∈ . Given any δ > 0, there is a smooth function h_δ(x) on

such that

h_δ|_{(-∞, c -2δ]} = f, h_δ|_{[c+2δ, +∞)} = g.

PROOF. By the proof of Lemma 2.1, there is a smooth function φ_δ (x) ∈ [0, 1] such that

Let

Then h_δ is the required function.

□

Still consider functions as in the above lemma. Denote

Let , be the surfaces in ³ generated by the rotation around the x-axis of the graphs of the functionsh_δ and respectively.

Assume that f(x), g(x) > σ > 0 for all x ∈ [c - 1, c + 1], and let δ ∈ (0, 1/2) be small enough. σ is independent of δ. Let

Then we have the following lemma.

LEMMA 2.3. There is a constant C(σ, M₂, N₂) > 0, such that

where

is the Gauss curvature of the surface

Moreover, we have

PROOF. By equation (5),

h_δ(x) >σ.

For x ∈ [c - 2δ, c + 2δ] , by Taylor expansion formula and equations (3)-(4) in Lemma 2.1, we have

where ξ_i 2 (c - 2δ, c + 2δ), i = 1, 2, 3, 4. Hence,

By assumption, C(σ, M, N) is independent of δ.

Since h_δ → as δ → 0, we have

|Vol() - Vol()| → 0, as δ → 0.

In fact, if x < b,

|h_δ - f| = |(g - f)ψ| → 0, as δ → 0;

and if x > b,

|h_δ - f| = |(f - g)φ| → 0, as δ → 0.

□

REMARK 2.4. In Lemma 2.2, if we suppose f"(c) = g"(c) additionally, and we set

by a similar argument as in the proof of Lemma 2.3, we get

< 14(M₃ + N₃).

LEMMA 2.5. There is a smooth function ø(x)(0 < ø(x) < b) defined on

, such that the following conditions are satisfied.

1. ø(x) = b, for x < 0, ø(x) = 0 for x > a;

2. ø^(k)(0) = ø^(k)(a) = 0, for any k ∈ ⁺;

3.

< 15(2³(3!)²b + max {2³(3!)²b, 3b/a}).

PROOF. Let

Here we select 0 < η < 1 such that ø₁(ηa) > ø₂(a - ηa).

By a result of Dan Henry (Henry 1994) (which is available on the net at the address: http://www.ime.usp.br/map/dhenry/danhenry/main.htm) we have

Let p₁(x), p₂(x) be two polynomial functions as in Figure 1 which join ø₁(x), ø₂(x) to a line l C²-smoothly respectively.

Let

It is clear that C_j(a, b) is in a small neighborhood of max{2³(3!)²b, b/((1 - 2η)a)}. Therefore by Remark 2.4, we have

Let us recall some properties of the pseudo sphere. Suppose the parametric equations of pseudo sphere. are

where t ∈ , π), θ ∈[0,2π]. We only consider the part of x > 0, and we have

A direct calculation implies that

LEMMA 2.6. For any given 0 < ε << 1 and 0 < δ << 1, there is a smooth function h_ε,δ(x) on [0, +∞) such that

1. For x ∈ [0, x_2ε- , the parametric equation of h_ε,δ(x) is

where t ∈ , t_ε], t_ε satisfies the equation ln(tan + cos t_ε) = x_2ε- ;

2. For x ∈ [x_2ε + , +∞), h_ε,δ(x) = ε.

Here

PROOF. Suppose f(x) is a smooth function which is defined as

where t ∈ [, π).

It is easy to check that point

belongs to the image of function f(x). Suppose line AB is tangent to f(x) at point A (Fig. 2). B is the intersection point of line AB and x-axis. AC is perpendicular to x-axis. D, E are the midpoints of AB and BC respectively. DF is parallel to x-axis. And x_F = x_B.

It's easy to see that

|AB| = 1.

Thus, we have

and

Define (x) as follows

That is the broken line .

We smooth it by the method in Lemma 2.1 and denote the result smooth function by g(x). Choose

By inequality (4), we have the estimate:

Next, we observe that f(x_2ε) = g(x_2ε) and f'(x_2ε) = g'(x_2ε). According to Lemma 2.2, for any 0 < δ < |CE|, there is a smooth function k_δ(x) on [0, x_2ε + |CE|], such that

Choose point P = (x_P, y_P) ∈ Im f(x) and Q = (x_Q, y_Q) ∈ Img(x) such that

According to Lemma 2.3, we have

< 4(M + N),

where

Since f"(x) = (see (11)), f"(x_2ε) = . Because is a increasing function aboutx for x ∈ (0, 1),

M = f"(x_P)| < .

Then, we get a global smooth function h_ε,δ(x) on [0, +∞) such that

since

REMARK 2.7. Let be the surface in ³ generated by the rotation around the x-axis of the graph of the function h_ε,δ. Then we have the estimate about the sectional curvature:

1. For x ∈ [0, x_2ε -],

2. For x ∈ [x_2ε + ,+∞),

So, we can choose a constant C which is independent of ε and δ, such that

<C.

LEMMA 2.8. For any given 0 < ε << 1 and 0 < δ << 1, there is a smooth surface Σ_{ε, δ} which is generated by rotation of the graph of a smooth function h_ε,δ(x) satisfying the following conditions along x-axis.

1. h_ε,δ(x) =

.

2. For x ∈ h_ε,δ has following parametric equation:

where x ∈ .

3. h_ε,δ(x) = ε, for x ∈

x_2ε and t_εare the same as in Lemma 2.6. Moreover, the sectional curvature of Σ_{ε, δ} is bounded by a constant C which is independent of ε and δ.

PROOF. By Lemma 2.2, 2.3, 2.6 and Remark 2.7.

□

REMARK 2.9. If we let δ (which used in Lemma 2.2-2.3) be small enough in Lemma 2.8, the area of the smooth surface above is less than (2 + )π + 1. If we add two ends to the surface, we will get the required Y-piece as showed in Figure 4.

3 MINIMAL VOLUME OF

³

By Lemma 2.8 and torus decomposition of

³ in (Cheeger and Gromov 1985), we can construct a sequence of complete smooth metrics on ³ with bounded curvatures and the corresponding volumes tend to zero. So the minimal volume of ³ is zero. The completeness of every metric we constructed is followed from Proposition 3.1. Note that the diameters of the Y-pieces we constructed have a positive lower bound. By Hopf-Rinow theorem (Chavel 1993), (³, g_δ) is also geodesic complete.

PROPOSITION 3.1. Let A₁⊂ A₂⊂ ... A_n ⊂ ... be a sequence of compact metric spaces. Suppose d_n is the distance function on A_n, and = d_n for all n. Define function d: A = A_n → by d(x, y) = d_m(x, y), where m is the positive integer such that x, y ∈ A_m. Then (A, d) is a metric space. If additionally we suppose that there is a sequence of concentric balls {B_n} such that B_n ⊂ A_n and diameter(B_n) → ∞ as n → ∞, then (A, d) is a complete metric space.

Before the proof of Proposition 3.2, it maybe useful to keep Figure 5 in mind which describes the construction of metrics on ³.

As in (Cheeger and Gromov 1985, Example 1.4), we decompose

³ into a sequence of solid to ruses,

C₁⊂ C₂⊂ ... ⊂ C_n ⊂ ...,

such that

³ = C_i, where C_i = 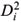× S¹, {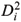} are closed disks. Every solid torus is contractiblein the next. Let

Let A_i denote the axis (i.e. {0} × S¹⊂ 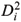× S¹) of C_i. The tubular neighborhood of A_i+1 denotes by

Then, we have

where is a surface with nonempty boundary which consists of three circles.

PROPOSITION 3.2. MinVol(

³) = 0.

PROOF. Our goal is to construct a sequence of complete smooth metrics g_ε on ³ such that

Vol(

³,g_e) → 0, as ε → 0,

and the corresponding curvatures are uniformly bounded by 1, i.e.

< 1.

In Lemma 2.8, we have constructed a surface (disk) Σ with nonempty boundary (circle) ∂ Σ satisfying

and, if restricted on a tubular neighborhood of ∂ Σ in Σ, g_Σ is product metric.

As has been mentioned in Section 2, it is also easy to construct metric on Y-piece () satisfying the similar conditions as above. With such metric, looks like as in Figure 6.

Next, we give the explanation in details as follows.

For , we assign it a metric as in Lemma 2.8. Denote the geometric surface by ,_δ, or simply by , where the means the length of ∂ is pe under the metric given. By Remark 2.9,

Vol () < (2 + 2 ) π + 1,

when δ is small enough.

For , i > 1, define

, for i> 1.

We assign a metric to be a Y-piece (see Fig. 6), and denote it by

Y_i = Y_2i,ε =

which means the lengths of the components of the boundary ∂Y_2i,ε are respectively.

In summary, we use the following notations:

We simply write the last equation as

Then the process of constructing the metric g_ε on ³ is as follows.

According to the torus decomposition of

³, to get a global smooth metric on ³, we have to glue the metrics on the pieces

along boundary circles or the product factors S¹ in certain pairs and certain order. The metrics on such product manifolds are chosen simply as product metrics. means that the circle has length 2π ε under the given metric ε²dθ². The relation between the lengths of the boundary circles must be related to ε. The order and the relation of gluing is stated as follows.

Let ∂Y_i;(1) × I, ∂Y_i;(2) × I, and ∂Y_i;(3) × I denote the three tubular neighborhoods of the three boundary components of Y_i for i > 1.

First, the metrics on ∂Y_i;(3) × I × and ∂Y_i;(1) × I × are chosen to be the following product metrics:

and

The order of gluing here is that we direct glue the first term of (16) and the third term of (17); and direct glue the third term of (16) and the first term of (17).

Second, the metrics on ∂Y_i;(2) × I × and × I × ∂M_iare chosen to be

and

The order of gluing here is that we exchange the variables α and θ in the second (or first) metric, so that they can be glued directly with the first (or second) metric.

At last, what remains to do is to glue the metrics on ∂M₀ × I × and ∂Y_i;(2) × I × .

Thus, we get a sequence of complete metrics g_ε,δ (simply denote by g_ε) on ³ such that

<C,

where C is independent of ε and δ; and we have

and

By scaling and the fact

where n = dimM, we get our metrics (still denote by g_ε) such that the curvature is uniformly bounded by 1, while the volumes of the surfaces are finite, since

Hence,

Therefore,

MinVol(

³) = 0.

□

The proposition is proved.

4 MINIMAL VOLUMES OF

ⁿ FOR n > 3

PROPOSITION 4.1. MinVol(

⁴) = 0.

PROOF. We still use the notations (13) and (14) in the proof of Proposition 3.2. The metric on Y_i(i.e. Y_2i,ε or ) is denoted by g_2,ε (means the second kind metric).

Let the metric on Y_i × × be

where

and f(t) is defined by

It is easy to prove that f(t) is a smooth function. Moreover, f(t) satisfies

Replace the metric on (that is, the surface M_i, see (13)) by the metric and denote the surface (, ) by , where ε(t) means that the length of the boundary circle is a function of t. If we want to realize the smooth gluing (i) as in Figure 7, that is to glue the metric on factor and the metric on boundary , the direct way is to require that the metric on a small tubular neighborhood of∂ is a product metric, and

Thus

ε(t) = f(t)ε.

But, in order to make the volume Vol( × × ) finite, the metric on × × should has the following form

The gluing (ii) is similar to (i). Hence, the metric g_2,ε on Y_i (i.e. ) should be changed to g_2,f(t)ε. That is,

becomes to

To realize the gluing (i) and (ii) while keeping the curvature bounded, we first construct an e related smooth function G_ε(x, t) (simply denoted by G(x, t), see (36)) which is used to construct the smooth surface . After that, we calculate the sectional curvatures and claim that the sectional curvatures are bounded. Then, by calculating the volumes, we complete the proof. For state clearly, we divided the proof into several steps:

Step 1: Construction of functionG(x, t). By Lemma 2.5, there is a C^∞ function ø() (0 < f() < 1) on [-1, +∞) such that

There exists a constant C > 0, such that

In particular,

Let

where t ∈ and = f(t)x. Let0

where h(x) satisfies

For simplicity, let

Then, we have

Then we define the function G(x, t) by

Step 2: Calculation of the sectional curvature of surface Σ_G(x,t). Now for every t, let Σ_G(x,t) be the surface generated by rotation of the graph of the function G(x,t). Σ_G(x,t) is just a part of surface . It can also be used to construct the surface .

On × Σ_G(x,t) × , the metric is given by

where θ, α ∈ [0,2π]. Let

Then by the structure equations, we have

So the curvatures are

Step 3: We claim that:

PROOF. By Equation (32), we have

By Equation (33), we have

By Equation (36), we have

Hence, by Equations (30)-(36), (40)-(42), we have Similarly, we have Then,

Similarly, we have

Then,

if and only if

That is

This implies that f(x)x → +∞, and it is a contradiction since f(x) < . Hence, there is a positive number σ > 0 which is independent of x, t and ε, such that

Therefore,

According to inequalities (43), (44) and (47), curvatures in (38) must be bounded. So the Claim isproved.

□

Step 4: Conclusion. Therefore, for every piece considered above, we have constructed a metric on it.By scaling if necessary, we can make the metric satisfying the condition that the curvature is bounded by 1. Moreover, we have

and

Hence, we have construct a sequence of complete metrics g_ε with bounded curvatures on ³ × , and

Vol(

³ × , g_ε ) → 0, as ε → 0.

Therefore,

MinVol(

⁴) = 0.

THEOREM 4.2. MinVol(

ⁿ) = 0 for n > 3.

PROOF. Every positive integer n can be written in one of the forms:

3k, 3k + 1 = 3(k - 1) + 4, 3k + 2.

Then

ⁿ (n > 3) can be written as

Take product metric on it, then MinVol(

ⁿ) = 0 since MinVol(³) = MinVol(⁴) = 0 and MinVol(²) = (2 + 2)π.

□

ACKNOWLEDGMENTS

This work was partially supported by the National Key Basic Research Fund (China) G1999075104, NSFC Grant 10671171 and NSFC Grant 10401015. We wish to thank Professor Xi fang Cao for his suggestions. The third author would like to thank Professor Detang Zhou for his significant suggestions and discussions about the completeness of the metrics constructed. Also we would like to thank Professor Qing Zhou and Professor Ying Zhang for their important suggestions on metric construction on Y-pieces. We would also like to express our sincere gratitude to the referees for their interest, careful examination and valuable suggestions.

Manuscript received on January 30, 2008; accepted for publication on May 27, 2008; presented by ARON SIMIS

AMS Classification: 53C20

• BAVARD C AND PANSU P. 1986. Sur le volume minimal de R² Ann Scient de l'ÉNS 19: 479-490.
• BESSIÈRES L. 1998. Un théorèm de rigidité différentielle. Comment Math Helv 73: 443-479.
• BOWDITCH BH. 1993. The minimal volume of the plane. J Austral Math Soc (Series A) 55: 23-40.
• BUSER P. 1992. Geometry and Spectra of Compact Riemann Surfaces. Birkhäuser, Boston.
• CHAVEL I. 1993. Riemannian Geometry: A Modern Introduction. Cambridge University Press.
• CHEEGER J AND GROMOV M. 1985. On the Characteristic Numbers of Complete Manifolds of Bounded Curvature and Finite Volume. H.E. Rauch Memorial (Chavel and Farkas Eds.) I: 115-154.
• CHEEGER J AND GROMOV M. 1986. Collapsing Riemannian manifolds while keeping their curvature bounded. J Differential Geom 23: 309-346.
• CHEEGER J AND GROMOV M. 1990. Collapsing Riemannian manifolds while keeping their curvature bounded II. J Differential Geom 32: 269-298.
• FUKAYA K. 1990. Hausdorff convergence of Riemannian manifolds and its applications. Recent topics in differential and analytic geometry. Adv Stud Pure Math 18-I: 143-238.
• GROMOV M. 1982. Volume and Bounded Cohomology. IHÉS 56: 5-99.
• HENRY D. 1994. Useless facts about the derivatives of e^−1/x , x > 0 manuscript on http://www.ime.usp.br/map/dhenry/danhenry/main.htm, Feb.
• PATERNAIN GP AND PETEAN J. 2003. Minimal entropy and collapsing with curvature bounded from below. Invent Math 151: 415-450.
• SOMA T. 1981. The Gromov invariant of links. Invent Math 64: 445-454.

Publication Dates

• Publication in this collection
  25 Nov 2008
• Date of issue
  Dec 2008

History

• Received
  30 Jan 2008
• Accepted
  27 May 2008