lambda - type phase transition for a weakly interacting bose gas

Abstract

It is suggested a general mechanism through which a lambda-type behavior is produced in the specific heat of a Bose gas near the critical temperature Tc . It is essential that the quasiparticle spectra have a gap proportional to the condensate. It works for a general class of quasiparticle spectra, and in particular, for the weakly interacting Bose gas. The introduction of a chemical potential in the theory is briefly discussed.

l - type phase transition for a weakly interacting bose gas

I. Cabrera^a,b, D. Oliva^a,b and H. Pérez Rojas^a,b,c

^aInternational Centre for Theoretical Physics,

P.O. Box 586 34100 Trieste, Italy

b Grupo de Fisica Teórica, ICIMAF,

Calle E No. 309, Vedado, La Habana 4, Cuba

c Helsinki Institute of Physics,

P.O. Box 9 (Siltavuorenpenger 20C), FIN-00014, University of Helsinki, Finland

Received on 22 November, 1999

It is suggested a general mechanism through which a l-type behavior is produced in the specific heat of a Bose gas near the critical temperature T_c . It is essential that the quasiparticle spectra have a gap proportional to the condensate. It works for a general class of quasiparticle spectra, and in particular, for the weakly interacting Bose gas. The introduction of a chemical potential in the theory is briefly discussed.

I Introduction

In his book on Statistical Mechanics, [1] Feynman refers to the l point behavior and expresses his view that perhaps part of the explanation of the l transition involves Bose condensation. One interesting model of the l behavior is the one presented by Ceperley [2], but in it the boson system is simulated by means of Monte Carlo techniques using path integrals. However, although the analytic behavior of the specific heat in a neighborhood of the critical temperature is provided by renormalization group methods, which gives an accurate description of the l behavior (see i.e. [3]), we are not aware of any model exhibiting the mechanism through which the condensate induces the divergence of the specific heat c_v at T_c. There are two phenomena which are expected to be explained by a satisfactory model of the weakly interacting bose gas: 1) the existence of a gapless mode, which is more manifest at T = 0, and 2) the l behavior, which would appear at finite temperatures. In our opinion both phenomena must be manifest even at the one-loop level, as it is in the relativistic models we mention below, but which is not the case in the usual non-relativistic model, i.e., the l behavior cannot be found by using the Bogoliubov's spectrum [4] for the weakly interacting Bose gas near T = 0 by taking it as valid at temperatures near the critical point.

Our aim is to show that if the bosonic quasiparticle spectra has a gap proportional to the condensate, a divergence in c_v appears at the transition temperature, and that this is the case for the weakly interacting Bose gas near the critical temperature.

First of all, we wish to remind the simple model of the temperature self-interacting scalar one-component field f with Z₂ symmetry breaking x = áfñ in relativistic quantum statistics [5]. Here the symmetry breaking parameter plays the role of a field condensate. The effective potential is V(x, T) = + V₁(T, x), where l is the coupling constant, -a² is the negative mass term and V₁(T, x) is the sum of T-dependent tadpole diagrams. On the mass shell V(x_c, T) =W is the thermodynamical potential, x_c being the extremum of V(x, T), where the spectrum is (p) = , M = 2l₁x_c.

In the high temperature limit one has V₁(T, x) = lT²x²/8, the extremum of V(x, T) leading for T < T_c to a temperature-dependent mass M(T) = l₁, T_c = 4a²/l being the critical temperature for symmetry restoration. The infrared contribution to the thermodynamical potential in the limit T 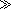M(T) is DW . One obtains then a specific heat Dc_v = -T

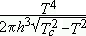c³l₁³ which diverges as t^-1/2, t = |1 - T/T_c|, for T ® T_c, showing a l-type behavior. This expression shows that the divergence appears already at the one-loop approximation of c_v, although it is quantitatively satisfactory for t 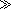l₁ [6], since in the region closer to T_c, the contribution from higher loops must be considered. In that region, renormalization group techniques gives an universal expression for the divergence as t^-a, where a < 1/2 .

In the two component scalar field case, having U(1) symmetry, two modes appear after the symmetry breaking, the massive ₁(p) = c, M = 2l₁x_c, and the massless ₂(p) = pc. The contribution to the thermodynamic potential infrared term is the same as in the previous case (the massless term does not contribute), and c_v diverges as t^-1/2.

Actually, both in the relativistic and non-relativistic cases, with spectra

_r =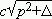 , and

_n = p²/2m + D, respectively. By taking some characteristic small momentum p₀ and introducing x = cp/T, calling h = cp₀/T, it is easy to see that there is a common infrared contribution to W ~ -AT^5/2ò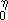x⁴dx/[x² + DT^-1] ~ A¢TD^3/2, where the mass (gap) D ~ b[1 - (T/T_c)^g ], g > 1, and A, A¢, b being constants. Then one can state as a theorem that for T ® T_c , the one-loop thermodynamic potential obtained from such spectra leads to a divergent c_v behavior as ~ D^-1/2.

In the relativistic limit this comes from (A contains a factor ^-3 ):

The the infrared limit of the last expression is

This means that near T_c

From c_V = -T, we get that c_V diverges as D^-1/2.

In the non-relativistic case we have a similar formula, by taking x = p/, since

from which we get the same behavior near T_c.

It is expected that any system having a spectrum of similar infrared properties than the previous ones, would have also a specific heat having an infinite behavior at the critical point. We would like to show that such is the case of the Bogoliubov model of the weakly interacting Bose gas near the critical point. It contains a gap due to the condensate, which leads to a divergence in the specific heat (in the region T/T_c < 1), already at the one-loop approximation.

II Bogoliubov Hamiltonian near the critical point

We will start from the quantized Hamiltonian for a weakly interacting Bose gas expanded in terms of the elementary creation and annihilation operators for spinless boson fields, we shall assume momentum conservation in the interactions and call U(r) the repulsive potential of the two body interaction, U = òòd³reU(r). For p = 0 (zero momentum transfer in the collision) U_o = òd³rU(r). In the temperature interval we are considering the momenta are very small and we can assume that the momentum transfer in each collision is almost zero, p 0. For that reason it is possible to express approximately the matrix elements by using U_o. The scattering length can be written then as a = U₀, and a > 0 since the potential is repulsive.

We shall assume the conditions a/l 1 and ra³ 1 where l is the thermal de Broglie wavelength and r = N/V the particle density. Then, by starting from the fact that the occupation number of the ground state n₀ is a large number, we will write a_o = e^iq, a = e^-iq. From this we would get an infinite set of (non-equivalent) representations as a consequence of breaking the first order gauge symmetry [7] y(x) ® e^iay(x) (y(x) = V^-1/2åe^ikxa_k), and we take the physical representation as the one with q = 0 which leads to,

We assume conservation of the total number of particles N and then å_p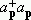 + n₀ = N. The usual procedure for T® 0, by following Bogoliubov [8] [9] (see also [10]), assuming that å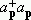= ån_p

N, is to substitute in (5) n by [N - å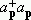]² in the first and n₀ by N in the third term, which leads to the cancellation of a term 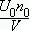å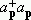 in the last term in curly brackets of (5). This means to neglect one term of second order in ån_p, the number of excited particles. But if ån_p» N, one cannot neglect the term (ån_p)² = å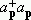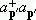. This is the case for temperatures near and below the transition point, and even for very low temperatures; e.g., as in the case n₀

N/2, ån_p

N/2. Thus, we keep n₀(T) explicit in our formulae as a quantity decreasing with increasing temperature. We conclude that Bogoliubov' gapless spectrum is an approximation valid actually for T = 0 , for almost all the system in the condensate.

In our present approach the last term in (5) accounts for the energy due to the interactions of particles with momenta p ¹ 0. Since we are considering an interval of temperatures very near (but below) the transition temperature, we assume that such term is approximately constant and take it as E_N=O(U₀N²/2V). Then the problem we are left with is the diagonalization of the sum of the second and third terms in (5). Our assumptions are valid for a wide range of values of n₀ (if N 10²³ our approximation is good up to, say, n₀ 10²¹). Thus, we keep our calculations in the one-loop approximation and in terms of n₀ and when compared with the standard Bogoliubov's model [8] it differs in the coefficient of the last term in curly brackets, which is changed from 2 to 4. The next step is to make the usual Bogoliubov's canonical transformation a_p = (b_p - a_pb

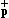_p)/ and a=(b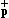-a_pb_-p)/ as a result of which we obtain the diagonalized Hamiltonian,

where E_o = [n_o² - n_o] - a_p and a_p = [4 + - e(p)], and if we denote by K = , then

is the spectrum of the new Bose quasiparticles representing the elementary excitations of the system, where b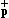,b_p are their creation and annihilation operators. The limit T® 0 does not lead to Bogoliubov's spectrum, in which the gap term 2K is absent, since both models correspond to different approximations. Actually, having only one mode, the present model bears in this respect more resemblance with the Z₂ case than with the U(1) one. In our model the long wavelength limit is obviously not linear in p, as it is usually. We may argue that our limit is not T ® 0 and also that its small value probably makes difficult to identify it among the experimental errors for p ® 0 near T_c. Actually, as K kT is very small (for n₀ 10²⁰, K 10^-12 eV) it can be usually neglected, but it is able to produce the macroscopic effect of a typical l-type divergent specific heat near T_c. As lim_{p® 0} e(p)=2K, the gap parameter K formally behaves as the analog of a rest energy in relativistic dynamics. This gap has the property that it decreases with temperature and goes to zero, as well as E₀, a_p, for T ® T_c.

Below we will calculate the thermodynamic potential by taking the chemical potential m = 0 by assuming that the number of quasiparticles n_q = å_pb

b_p is not conserved (See, however, next section).

The term K is proportional to n₀, the eigenvalue of a₀ with regard to the coherent ground state. In our present approximation, the quasi-particles are actually massive since their effective mass is m^* = [¶²e(p)/¶p²]^-1|_p=0 = m/4. An explicit computation of c_v showing the divergent behavior close to the critical temperature is easily done by doing first the temperature expansion of the thermodynamic potential of the quasiparticles by taking the spectrum (7).

Due to the interaction term, the ground state energy E₀ is different from zero,

where E, F are the usual elliptic integrals.

III The specific heat

We will calculate the thermodynamical potential of the quasiparticles W . The total energy is then U = E₀ + E_N - T(¶W/¶T) + W. We will do also the asymptotic expansion of W close to T_c for T < T_c. By changing to the variable x = it reads W = dxx² ln[1-e-], where M = Kb 1 and we obtain an expansion in terms of it, in the same way done in the case of the effective potential in the temperature scalar model [5]. After some calculations which we outline in the appendix (these are given in detail in [11]), the perturbative expansion of W in powers of K is obtained, and from it the specific heat is given by c_v = - T as,

Here a = ()(). The last term diverges at T_c. We shall write n_o = Nf(T) where f(T) = (1 - N_e/N) and N_e is the density of particles not in the ground state. By starting from N_p = n_q(1 + a)/(1 - a) + a/1 - a, [12] the expression for N_e = ^-3ò d³pN_pis given in the Appendix as an expansion similar to the one for W. Under the assumption Kb 1, the leading terms are

where N_e0 = AT^3/2 and A = (2pmk/h²)^3/2z(3/2), C = p^1/2()AK^1/2/k^1/2z(3/2). This equation for N_e is to be solved by iteration, by taking in M as a first approximation f(T) = [1 - N_e0/N]. It must be observed that as T decreases, the rate of condensation in (10) is stronger than for the ideal gas. We will take N = AT_c^3/2. By evaluating (9) with helium parameters and taking the scattering length as a = 10^-10 cm, the curve for c_v(T) defined in the region T £ T_c is depicted in Fig. 1. Then an infinite l-type behavior of c_v(T) similar to the scalar field case results. Such a behavior cannot be obtained by using the usual Bogoliubov's spectrum [4].

Figure 1:
c_v/Nk-curve obtained from our model, where the l shape is shown. For T > T_c the ideal gas curve has been drawn for comparison.

The more exact part of the c_v curve lies in the region near and below T_c since we assumed E_N constant and Kb 1. But the last condition is valid even for very low T and E_N decreases to small values as T ® 0. Thus, the c_v curve drawn is approximately valid even in that region. The behavior of c_v for T > T_c is beyond the scope of the present letter. Such problem must be investigated by starting from an adequate model of imperfect gas in the region T ³ T_c[13].

IV The introduction of a chemical potential

The absence of a second (gapless) Goldstone mode does not mean an incompleteness of our model, since although we are dealing with a two-dimensional problem, the present representation behaves as similar to the one-dimensional scalar case seen in the Introduction, whose spectrum bears a gap. On the other hand, an investigation of the Bose gas by using the Green's function method made by Beliaev [14] leads to the usual Bogoliubov spectrum in terms of n₀ at strictly T = 0. However, this is made by introducing a chemical potential m, which loses its usual meaning, since the calculation of the Green functions by starting from a density matrix, demands commutation between the Hamiltonian H and the number N of excited particles, which is not the case since the quasiparticle number is not conserved. If m = 0 in [14] our spectrum is reproduced, and the gap cannot be erased in higher loop calculations.

The papers [14] were written before the formulation of Goldstone theorem [15], which lead to a better understanding of the theory of spontaneous symmetry breaking. Actually, Beliaev results are based in the same operator algebra representation used by Bogoliubov, and describe the existence of only one mode, which is gapless. It misses a second mode having a gap, which is expected to appear in usual U(1) gauge invariant models with spontaneous symmetry breaking, as was pointed out in the Introduction. If the chemical potential is understood not in the usual sense, but as a parameter introduced before the breaking of the symmetry, one finds that the representation used by Beliaev is not the unique one: there is a set of infinite unequivalent ones, which is typical of the spontaneously broken symmetry case [7]. One of these is being investigated by two of the present authors (D.O. and H.P.R.) for the temperature case. In it, as in the U(1) scalar relativistic model considered in the introduction, a two mode spectrum is found. One of them is a gapless Goldstone mode and the other one has a gap, the later producing a lambda behavior in the specific heat. Work in this problem is in progress.

V Conclusions

In relativistic models of a scalar bose gas with symmetry breaking, it is found a divergent behavior of the specific heat at the one loop level of the thermodynamic potential, which comes from the temperature dependent mass (gap) in the spectrum, which is consistent qualitatively with renormalization group predictions. By analogy, we consider the usual Bogoliubov model near the critical temperature for the phase transition, and a gap is shown to be present, leading to a divergence in the specific heat. We discussed briefly the introduction of a chemical potential in the model, as done in Beliaev procedure [14], and the absence of a Goldstone mode. We conclude that the model we have discussed, although not being perhaps the final one, is an step towards it, since it is able to describe the property of lambda behavior in the non-ideal Bose gas.

Acknowledgments

The authors would like to thank Professor M. Virasoro, IAEA and UNESCO for hospitality at the International Centre of Theoretical Physics. All authors thank A. Amezaga, A. Cabo, S. Fantoni, S. Giovannazi, F. Hussain, G. A. Mezincescu, C. Montonen, K. Narain A. Polls, A. Smierzi and R. Sorkin for valuable comments and suggestions. H.P.R. would like to thank his former student R. Torres Rivero for having called his attention to the possible relation of the l -type spectrum with some temperature-dependent mass model, and M. Chaichian for several illuminating discussions and hospitality in the High Energy Physics Division, Department of Physics, University of Helsinki. The partial support of the Academy of Finland under Project No. 163394 is greatly acknowledged.

VI Appendix

To make an asymptotic expansion of W in terms of M = Kb, we write W = W(0) + |_M=0M + R(M) , where we stop our expansion in the first-order term and R(M) is certain function of M which we are going to find out by using R(M) = W(M) - W(0) - |_M=0M and = - |_M=0. Then

from which |_M=0 = 4(2m)^3/2VG(3/2)z(3/2)/b^5/2

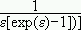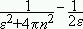³(2p)²) The R(M) function is obtained by calculating ¶R/¶M from these expressions, by using the Matsubara sum = , and performing the adequate regularizations, we get finally (see also [11]),

By a similar procedure,we get,

References

[1] R.P. Feynman, Statistical Mechanics Benjamin, Reading, Mass. (1982).

[2] D.M. Ceperley, Rev. Mod. Phys. 67, 2 (1995).

[3] J.A. Lipa, D.R. Swanson, J.A. Nissen, T. P. Chuy and U.E. Israelsson, Phys. Rev. Lett. 76, 944 (1996).

[4] K. Huang, in Bose-Einstein Condensation, Edited by A. Griffin, D.W. Snoke and S. Stringari, Cambridge University Press (1995).

[5] L. Dolan and R. Jackiw, Phys. Rev. D9, 3320 (1974).

[6] D.A. Kirzhnits and A.D. Linde, Ann. Phys. 101, 195 (1976).

[7] F. Strocchi, Elements of Quantum Mechanics of Infinite Degrees of Freedom, World Scientific, Singapore (1985).

[8] R. K. Pathria, Statistical Mechanics, Pergamon Press (1972).

[9] N. N. Bogoliubov, J. Phys. USSR 11, 23 (1947).

[10] K.A. Brueckner and K. Sawada, it Phys. Rev. 106, 1117 (1957)

[11] I. Cabrera and H. Perez Rojas, Cond-mat 9797044

[12] L.D. Landau and E. M. Lifshitz, Statistical Physics, Pergamon Press (1966).

[13] A.L. Fetter and J.D. Walecka,Quantum Theory of Many Particle Systems, Mc Graw Hill Book Co, (1972).

[14] S.T. Beliaev, JETP 34 , 289 (1958); JETP 34 , 299 (1958).

[15] J. Goldstone, Nuovo Cimento 25, 154 (1961).

Publication Dates

• Publication in this collection
  23 Apr 2002
• Date of issue
  Dec 2001

History

• Received
  22 Nov 1999