New methodologies for the calculation of Green's functions for wave problems in two-dimensional unbounded domains

New methodologies for the calculation of Green's functions for wave problems in two-dimensional unbounded domains

R.T. Couto

Departamento de Matemática Aplicada, UFF - Universidade Federal Fluminense, 24020-140 Niterói, RJ, Brasil. [e-mail]

ABSTRACT

This work describes the application of new methodologies for the evaluation of the inverse Fourier transforms that yield Green's functions for both the wave and Helmholtz equations in the entire bidimensional domain.

Keywords: Wave equation, two dimensions, Green's function.

RESUMO

Este trabalho descreve a aplicação de novas metodologias para o cálculo das transformadas de Fourier inversas que fornecem as funções de Green associadas às equações da onda e de Helmholtz em todo o domínio bidimensional.

Palavras-chave: Equação da onda, bidimensional, função de Green.

1. Introduction

Green's functions for wave problems, both time-dependent and stationary ones, governed by the wave and Helmholtz equations, respectively, in unbounded domains having one, two or three dimensions have well known expressions (cf. reference [1], sections 11.2 and 13.2.2). The Fourier transform serves well in their determination, but the evaluation of the inversion integral in two dimensions - the case considered in this work is the most challenging.

For the Helmholtz equation, reference [9], on pp. 822-824, states that the inversion can be performed by using contour integration together with a change of complex variables of the type given in equation (2.10) below, but does not reveal the steps of the calculation. Later on, reference [4], on pp. 173-176, shows a little more thereof, but still BB BX1 outline which is hard to follow.

It is thus our purpose to offer here a detailed description of this methodology, but, to make an innovation, we apply it to the wave equation. Both retarded (Section 2) and advanced (Section 3) Green's functions are calculated. Green's functions for the Helmholtz equation are also obtained as a by-product (Section 4). We conclude the exposition with final comments (Section 5).

2. Retarded Green's Function for the Wave Equation

Green's function for the wave equation in a boundless two-dimensional domain is the solution of

with and in , and t and t' in . In this section, we consider the retarded or causal Green's function, for which

To solve the problem defined by (2.1) and (2.2), we first take the Fourier transform of (2.1) with respect to t, obtaining

where

To compute the inverse transform ,we modify this formula a little, by splitting the integral in the intervals and and performing the changing of variable in the first integral, obtaining

By using this formula, we avoid negative values of ω, what simplifies the development of the method.

Next, adopting the Cartesian coordinates χ and y of , in terms of which and we take another Fourier transform, now with respect to y, obtaining

where

We then solve the ordinary differential equation (2.5) under the conditions of continuity and finiteness for all x as well as an extra condition (as a consequence of the delta function) which follows from its integration in the infinitesimal interval (x' - ε, x' + ε) {cf. [2], section 12.2}:

The second integral above tends to zero, because it is the integral of a continuous function in a infinitesimal interval, and the last integral is equal to one. Carrying out the first integral and letting , we obtain the jump condition for at :

Notice that (2.5) is a homogeneous differential equation, except for x = x'; its solution for is thus of the form

In this equation, (decomposition of the square roots in their real and imaginary parts). Also, because of (2.6), it was necessary to consider arbitrary constants for x < x', c₁ and c₂, different from those for x > x' , d₁ and d₂. These constants are to be determined by imposing the finiteness, continuity and jump conditions. Once found , we can begin calculating the inverse Fourier transforms, first:

But before doing so, let us make three observations:

1. For real k and ω (variables introduced in the Fourier transforms), either a or b vanishes, that is, ab = 0. But, in this work, k is not a real variable. In fact, the method described herein consists in evaluating the Fourier inversion integral in (2.8) by considering it as a contour integral along the real axis of k-plane and then deforming this path of integration into another like those in Figure 3 to 5. Clearly, for the complex values of k along the new parths ab ≠0 most often.

2. We need to consider only a > 0. In fact, that we can take a > 0 without loss of generality is obvious, and the results for a = 0 are not necessary for the following reason: The set S of points of the k-plane corresponding to a = 0 are those in the real axis segment between - ω/c and ω/c as well as in the imaginary axis, and Figures 3 to 5 show that any of the new paths of integration contains a finete number of points of S (two points, to be precise). This means that a finite number of values of given by (2.7) with a = 0 are integrated along the new paths [in contrast with the infinite number of values along the real path in (2.8)], and since these values are finite, their contribution to the integral is negligible. As a conclusion, there is no need to consider a = 0.

3. Notice that (2.7) is not valid for k = ω/c (that is, for a = b = 0); but a valid expression for this case is not necessary, because the point k = ω/c never belongs to the new path of integration (c.f. Figures 3 to 5).

Let us now proceed completingthe determination of . In (2.7), we set c₂=d₁= 0 to avoid infinite values for . Requiring continuity at x = x', that is,, we can eliminate d₂, obtaining

By using (2.6), the jump condition, we find , whose substitution in the equation above furnishes the desired solution of (2.5):

or

with Re > 0 (because a > 0).

We now use this result in the inversion Fourier integral given by (2.8):

where X ≡ x - x' and Y ≡ y - y'.

Considering, as already mentioned, the integral in (2.9) as a contour integral along the real axis of the complex plane of k = k_x + ik_y, let us change the variable k to another complex variable ζ = ∅ + iu as follows¹:

from which, as indicated,

These equations with and define a map from the strip of the ς-plane shown in Figure 1 to the whole k-plane. Figure 2 shows that a vertical straight line φ = constant (≠ 0, π/2 or π) is mapped to a hyperbola (in the left half plane if φ = φ₁ < π/₂ or the right one if φ =φ₂ > π/₂) and that a horizontal line u = (= 0) a half ellipse (in the upper half plane if u = u₁ > 0 or the lower one if u = u₂ < 0). In fact, in the k-plane, (2.11) with φ =φ₀ (≠ 0, π/2 or π) or u =u₀ (≠ 0) can be seen respectively as the parametrization of:

• the left (if φ

  ₀ < π/2) or right (if φ

  ₀ > π/2) branch of the hyperbola

• the upper (if

  u

  ₀ > 0) or lower (if

  u

  ₀ < 0) half of the ellipse

Figure 1:
The domain and image of the map defined by (2.10)

Figure 2: The map in (2.10): hyperbolas and half ellipses as images of vertical straight lines and horizontal line segments, respectively. (A line in the φ₀-plane and its image are both drawn with the same pattern and oriented with the same kind of arrow.)

In addition, (2.11) with φ - 0 or π describes the portion of the real axis from ∞ to -(ω/c) or that from (ω/c) to ∞, respectively; with φ - π/2, the imaginary axis; and with u = 0, the portion of the real axis from -ω/c to ω/c.

We will evaluate the integral in (2.9) for the contour C = E1 ∪ H ∪ E₂ depicted in Figure 3, but with R → ∞, where:

• E₁ is the elliptical path given by (2.11) with

  u = u

  ₀

  = cosh

  ^-1(

  cR/ω) and φ varying from 0 to a suitable value φ

  ₀ yet to be determined

• H is the hyperbolic path given by (2.11) with φ = φ

  ₀ and

  u varying from

  u

  ₀ to -

  u

  ₀

• E

  ₂ is the elliptical path given by (2.11) with

  u

  ₀ = -

  u

  _{0 and} φ

  _{varying from} φ

  ₀

  _to π

Figure 3: The contour C used to evaluate the integral in (2.9).

Figure 4: The contour C for cosφ₀ < 0

Figure 5:
The contour along which the integral in the integral in (2.9) leads to the advanced Green's function.

In (2.9) we are faced with the problem of choosing the correct branch of the square root . Let us proceed considering both branches simultaneously (we will reach a point at which consistency will impose the correct one):

Therefore, using this and (2.10), we have that

and also that the exponent appearing in (2.9) can be written as follows:

where

The integral in (2.9) for the contour C can be split in three integrals evaluated on the three paths E₁, H and E₂ which compose C. Thus, using (2.12) and (2.13), we can write (2.9) as follows:

Now is the moment to determine φ₀. This parameter can be found in such a way that two integrals with respect to φ in (2.15) (those evaluated on the elliptical part of C) go to zero as R = (ω/c) coshu₀ = ∞ (that is u₀ = ∞), thereby considerably simplifying the calculations. Indeed, using (2.13), we see that these two integrals will tend to zero as u₀ ∞ if Re f (φ, ±u₀) - ±(o;/c) g(φ) sinh u₀ = ∞, what will occur if g(φ) < 0 in the Hist integral with respect to φ and g(φ) > 0 in the second one. This imposes the requirements

Looking at (2.15) and (2.13), we see that we actually do not need φ₀, but g(φ₀) and g'(φ₀). In order to calculate g'(φ₀), we need to develop (2.16a). Considering (2.14), we have

from which, solving for sin φ₀ [the positive value is taken, because φ₀ ∈ (0, π)] and the calculating cos φ₀, we obtain

where

(Notice that cosφ₀ may be negative, that is, π₀ > φ/2; in this case, the contour C is like that in Figure 4.) Therefore, using these results, we get

In this, in view of (2.16b), we choose the plus sign. Since this sign is the lower one in the appearing in (2.17), in each "±" and related to the two branches of , the lower sign is also the correct one. The substitution of (2.16a) and g'(φ₀) = ρ in (2.13) then yields

With this and the fact that the first and third integrals tend to zero, we can rewrite (2.15) as

Using (2.4) to calculate of this result, we obtain

Defining T ≡ t - t', recognizing {cf. [8], equation (6.28)} that the last pair of braces encloses an integral representation of the delta function

and changing to the variable v = cosh u, we can proceed the calculation as follows:

where U(τ) is the unit step function (equal to 0 for τ < 0 and to 1 for τ > 0). We thus obtain the final result

which, except for the multiplicative constant (due to little differences in the form of the wave equation considered), is the same expression obtained in references [1, equation (11.2.21)] and [9, p. 842, equation (7.3.15)], by using another method (by-integrating the corresponding three-dimensional Green's function).

3. Advanced Green's Function for the Wave Equation

In the previous section, it looks like (2.2) is never used. Nevertheless, the Green's function given by (2.20) indeed satisfies that causality condition:

We then may ask: To obtain the advanced Green's function, satisfying

what should be modified in the calculational method described above? The answer is simple but subtle: it is the contour used to perform the inversion integral (2.9) that needs modification. Incidentally, a contour formed with the hyperbolic and elliptical arcs that arise in the change of variable given by (2.10) is either compatible with (2.2) or (3.1). The contour in Figure 3 is compatible with (2.2). If we want (3.1) to be satisfied, the contour C to be used is that in Figure 5. Let us confirm this.

The integral in (2.9) for the contour in Figure 5 can be written in the form of (2.15) with a few obvious changes:

where f(φ, u) is still given by (2.13) and (2.14), remembering that the "±" indicates the use of both branches of in the first integral above and g(φ) < 0 in the third one, meaning that g(φ₀) - 0, as in the previous case, but now g'(φ₀) < 0. In this case, it is the upper sign in the in (2.17) the correct one. Let us then proceed from (3.2) with: (a) the upper sign in "±"; (b) u₀ → ∞, thereby making the first and third integrals go to zero; and (c) (from (2.18) with -ρ in place of ρ). By doing this, we obtain (2.19), but with -ρ in place of ρ, which, developed as before, leads to a result similar to (2.20):

This is the advanced Green's function, satisfying (3.1):

4. Green's Function for the Helmholtz Equation

Green's function for the Helmholtz equation can be easily obtained from the previous results. In fact, with the definitions of the new constants K ≡ ω/c and (in the present context, the parameters t' and ω are irrelevant) as well as , (2.3) takes the common form of the equation whose solution is the Green's function for the Helmholtz equation:

Moreover, we have seen that (2.19), as it stands or with -ρ in place of ρ, provides an integral representation for . But these two forms, except for multiplicative constants, can be recognized as known integral representations for the first or the second Hankel function of order zero (cf. equations (10) and (11) in [11], §6.21). We thus see that (4.1) has the two well-known elementary solutions

The decision to use either one, or a linear combination of the two, depends on whether the physical problem at hand involves only outgoing or incoming waves (cf. [7], Sec. 9.12, p. 470), or a superposition of these two kinds of waves.

5. Conclusion

The main part of the calculations developed above consists in solving Helmholtz equation (2.3) to obtain its solution in the form of the integral representation given by (2.19). This is actually the derivation of Green's function for Helmholtz equation (4.1), as explained in section 3 (where that integral representation is recognized as the first Hankel function of order zero). In the literature, there exists two alternative methods for the calculation of this Green's function by directly solving the two-dimensional Helmholtz equation as well as two indirect methods, in which that equation is not solved. With the intention of highlighting how different is the method described here, we present below a summary of all methods.

In this work, Green's function for the two-dimensional Helmholtz equation is obtained by directly solving this equation. First, a Fourier transform is used to reduce the two-dimensional problem into a one dimension problem which is relatively easy to solve. Next, by considering the corresponding inverse Fourier transform integral as a contour integral in the complex plane and performing a suitable change of the complex variable of integration, that integral can be considerably simplified [4]. It then becomes possible to identify this simpler integral with the forementioned Hankel function as well as to perform the calculations beyond equation (2.19).

The first already existing direct method is given in reference [10], where the calculation begins with the application of the two-dimensional Fourier transform. Then, in order to evaluate the inverse Fourier double integral, contour integration in the complex plane is used to perform one of the integrals, being necessary to carry-out a detailed analysis to determine the correct prescription for circumventing the real poles of the integrand. Thereafter, the resulting integral, by means of a few manipulations, is converted into a known integral representation of that Hankel function, thus ending the calculation.

The other direct method can be found in references [5], equations (5.1.14) to (5.1.16), or [6], section 1.2.2, where symmetry considerations are used to turn the problem one-dimensional, depending only on , in which a nonhomoge-neous Bessel equation of order zero, exhibiting a delta function δ(ρ) on the right-hand side, has to be solved. The general solution of this differential equation is well known for ρ ≠ 0. Therefore, it only remains to determine the arbitrary constants; this is accomplished by imposing the correct conditions for ρ → 0 and ρ → ∞ (to satisfy this condition at infinity - the radiation condition - it is easier to work with the general solution formed with the Hankel functions.)

One way of obtaining Green's function for the two-dimensional Helmholtz equation without solving directly this differential equation is by employing the method of descent (see reference [3], Ch. VI, §12, 3), by means of which the solution of the two-dimensional problem is obtained by integrating the solution of the corresponding easier three-dimensional problem with respect to the variable which spans the dimension being eliminated (the Cartesian variable z, in the case).

Another indirect way (see reference [1], section 13.2.2) is as follows: one first relates Green's function for the wave equation, , to Green's function for the Helmholtz equation, , and then, having determined the former (e.g., by descenting from the easier three-dimensional problem), he uses this relationship to calculate the latter. The first step is accomplished by noting that the stationary wave described by Helmholtz equation is a particular case of the generic wave motion described by the wave equation, from which it follows that .

The importance of a new method for a problem already solved resides in the method itself, for it is likely to have other applications. This is true even if such method becomes more involved in that particular problem, because this may not happen in others. In fact, greater generality often requires more elaboration.

Recebido em 29 Setembro 2012

Aceito em 16 Abril 2013

• [1] G. Barton, "Elements of Green's Functions and Propagation: Potentials, Diffusion, and Waves", Oxford University Press, 1989.
• [2] E. Butkov, "Mathematical Physics", Addison-Wesley Publishing Company, Reading, Massachusetts, 1973.
• [3] R. Courant, D. Hilbert, "Methods of Mathematical Physics", Volume II, Third Printing, Interscience Publishers/John Wiley & Sons, New York, 1966.
• [4] B. Davies, "Integral Transforms and Their Applications", Texts in Applied Mathematics 41, Third Edition, Springer-Verlag, New York, 2002.
• [5] D.G. Duffy, "Green's Functions with Applications", Studies in Advanced Mathematics, Chapman & Hall/CRC Press LLC, Boca Raton, Florida, 2001.
• [6] E.N. Economou, "Green's Functions in Quantum Physics", Third Edition, Springer Series in Solid-State Sciences 7, Springer-Verlag, Berlin, 2006.
• [7] F.B. Hildebrand, "Advanced Calculus for Applications", Second Edition, Prentice-Hall, Englewood Cliffs, 1976.
• [8] H.P. Hsu, "Applied Fourier Analysis", HBJ Publishers, San Diego, 1984.
• [9] P.M. Morse and H. Feshbach, "Methods of Theoretical Physics", McGraw-Hill Book Company, New York, 1953.
• [10] R. Toscano Couto, Green's functions for the wave, Helmholtz and Poisson equations in a two-dimensional boundless domain, Rev. Bras. Ens. Fis., 35, No. 1 (2013), 1304.
• [11] G.N. Watson, "A Treatise on the Theory of Bessel Functions", Second Edition, Cambridge University Press, London, 1944.

1

Instead of (2.10), we could have performed the change of variables

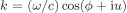, or even

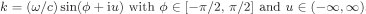, and only a few modifications in the development would be necessary.

Publication Dates

• Publication in this collection
  28 May 2013
• Date of issue
  Apr 2013

History

• Received
  29 Sept 2012
• Accepted
  16 Apr 2013