Classification of homogeneous quadratic conservation laws with viscous terms

Classification of homogeneous quadratic conservation laws with viscous terms

Jane Hurley Wenstrom^I; Bradley J. Plohr^II

^IDivision of Science and Mathematics, Mississippi University for Women Columbus, MS 39701

^IIComplex Systems Group, MS-B213, Theoretical Division Los Alamos National Laboratory, Los Alamos, NM 87544, E-mails: [e-mail] / [e-mail]

ABSTRACT

In this paper, we study systems of two conservation laws with homogeneous quadratic flux functions. We use the viscous profile criterion for shock admissibility. This criterion leads to the occurrence of non-classical transitional shock waves, which are sensitively dependent on the form of the viscosity matrix. The goal of this paper is to lay a foundation for investigating how the structure of solutions of the Riemann problem is affected by the choice of viscosity matrix.

Working in the framework of the fundamental wave manifold, we derive a necessary and sufficient condition on the model parameters for the presence of transitional shock waves. Using this condition, we are able to identify the regions in the wave manifold that correspond to transitional shock waves. Also, we determine the boundaries in the space of model parameters that separate models with differing numbers of transitional regions.

Mathematical subject classification: 35L65, 35L67.

Key words: nonlinear non-strictly-hyperbolic conservation laws, Riemann problems, viscous profiles.

1 Introduction

In this paper, we study the Riemann initial-value problem for a class of systems of two conservation laws

the initial data being

We assume that the flux function F is a homogeneous quadratic function that is strictly hyperbolic away from the origin, i.e., F¢(U_*) has distinct real eigenvalues l₁(U_*) < l₂(U_*) for all states U_*¹ 0. The origin is therefore an isolated umbilic point (a point at which the Jacobian is a multiple of the identity matrix). The present and previous studies [18, 19, 22, 10, 11, 12, 21, 7] have been motivated by the observation that a general system of two conservation laws can be approximated by such a quadratic system in the neighborhood of an isolated umbilic point.

We seek scale-invariant weak solutions of Riemann problems comprising (continuous) centered rarefaction waves and (discontinuous) centered shock waves (see, e.g., Ref. [23] for a general discussion of Riemann problems). In order for solutions to be unique, shock waves are required to satisfy an admissibility criterion. One criterion is the characteristic criterion of Lax [13], which imposes certain inequalities relating shock and characteristic velocities. An alternative is the viscous profile criterion of Gelfand [5], which requires each shock wave to be the limit, as e ® 0⁺, of traveling wave solutions of a particular family of parabolic systems

for which the original system (1.1) is an approximation. A comparison of these admissibility criteria appears in Section 2. The previous studies of the Riemann problem for quadratic models with isolated umbilic points have used either the Lax criterion or the viscous profile criterion with Q being the identity matrix. Here and in related work [6, 25] we explore more general possibilities for the viscosity matrix Q.

There exist shock waves satisfying the Lax criterion but not the viscous profile criterion, as well as shock waves satisfying the viscous profile criterion but not the Lax criterion. Among the latter are transitional [9] (or undercompressive [22]) shock waves. Transitional shock waves are constrained by one more condition than are Lax shock waves, and their end states must lie in special regions of state space. Moreover, the set of transitional shock waves is sensitively dependent on the viscosity matrix Q appearing in system (1.3). As a result, Q and its associated transitional waves play a key role in solving Riemann problems. In fact, the arrangement of transitional regions determines the qualitative structure of solutions of Riemann problems for quadratic models [6, 25]. The main results of the present paper are (1) a characterization of the set of transitional waves in terms of model parameters and coefficients of viscosity and (2) a corresponding classification of models.

In keeping with the view of quadratic models as approximations to general models near umbilic points, we limit our investigation to viscosity matrices that are constant. Furthermore, the discussion in Section 2 motivates our assumption that Q be symmetric and strictly positive definite when the quadratic model is put in the normal form of Schaeffer-Shearer [18]. To characterize the set of transitional waves for such a model, we first construct its fundamental wave manifold [8], which parameterizes all shock wave solutions, and we determine various important subsets (see Section 3). Then, in Section 4, we derive a condition, involving the parameters of the model and the coefficients of viscosity, that determines whether, and where, transitional shock waves occur. Finally, in Section 5, we use this condition to classify quadratic models according to the occurrence of transitional shock waves.

2 Shock wave admissibility criteria

A centered shock wave is a discontinuous solution of the form

where the propagation velocity s and the states U_- and U₊ are constant. For this wave to be a weak solution, the quantities s, U_-, and U₊ must be related by the Rankine-Hugoniot condition

As is well known, not all shock waves represent physically relevant solutions. We therefore impose an admissibility criterion to select appropriate solutions.

2.1 The characteristic and entropy criteria

The Lax characteristic criterion [13] was developed for weak shock waves in systems of conservation laws that are strictly hyperbolic and genuinely nonlinear. This criterion guarantees that the initial-value problem for the linearization of the conservation laws around the shock wave solution (2.1) is well-posed [23].

For the system of two conservation laws (1.1), the Lax criterion requires that, of the four characteristics on the two sides of a shock wave, precisely three impinge on the wave. This means that one of the following sets of inequalities is satisfied:

or

A shock wave satisfying inequalities (2.3) (respectively, inequalities (2.4)) is called a Lax 1-shock wave (resp., Lax 2-shock wave).

Because genuine nonlinearity fails for system (1.1) at certain states, the admissibility criterion must allow for composite waves, which consist of shock waves adjoining rarefaction waves of the same family (see, e.g., Ref. [15]). Therefore we regard the Lax criterion as allowing sonic Lax 1-shock waves (respectively, 2-shock waves), for which one or both of the inequalities l₁(U₊) < s < l₁(U_-) (resp., l₂(U₊) < s < l₂(U_-)) is replaced by an equality.

Lax also introduced the entropy criterion [14]. An entropy h for system (1.1) is a smooth real-valued function such that h¢ F¢ = q¢ for some q, called the entropy flux. Because a smooth solution U of the parabolic system (1.3) satisfies

the entropy is required to be compatible with the viscosity matrix [23, p. 399] in the sense that the quadratic form h¢¢(U_*)(·, Q(U_*)·) is strictly positive definite for all states U_*; when Q(U) º I, this means that h is strictly convex. A solution U for the conservation laws is admissible with respect to the entropy h provided that

in the sense of distributions. For the shock wave solution (2.1), this condition reduces to

2.2 The viscous profile criterion

The viscous profile criterion [5] for viscosity matrix Q requires a shock wave to be the limit, as e ® 0⁺, of a traveling wave solution of the parabolic equation (1.3). This criterion is appropriate when parabolic terms model physical effects that have been neglected in the hyperbolic system (1.1).

A traveling wave solution takes the form

where (x) U_± as x ® ± ¥. To be a solution of Eq. (1.3), must satisfy the ordinary differential equation

The state U_- is automatically an equilibrium point for the dynamical system (2.9), and, by the Rankine-Hugoniot condition (2.2), the state U₊ is too. A shock wave is said to have a viscous profile if there exists an orbit for system (2.9) that leads from U_- to U₊; a shock wave with a viscous profile is said to be admissible. For a system of two conservation laws, the question of shock wave admissibility is resolved by studying the family (2.9) of planar dynamical systems.

The properties of the viscosity matrix remain to be specified. Majda and Pego [16] introduced a criterion which, in the context of strictly hyperbolic systems of two conservation laws, reduces to the following: a viscosity matrix Q is strictly stable for system at a state U_* when

(a) the eigenvalues of Q(U_*) have positive real parts and

(b)

_j(U_*) Q(U_*) r_j(U_*) > 0 for j = 1, 2.

Here r_j(U_*) and _j(U_*), for j = 1,2, are right and left eigenvectors, respectively, of F¢(U_*) corresponding to the eigenvalue l_j(U_*), normalized so that _j(U_*) r_j(U_*) = 1. Strict stability implies that the initial-value problem for the linearization

of Eq. (1.3) around U_* is uniformly well-posed in L² as e ® 0⁺.

A homogeneous quadratic model with an isolated umbilic point is strictly hyperbolic except at the origin, so we require Q to be strictly stable at each state U_*¹ 0. Bearing in mind that the models considered in this paper arise as approximations to general models near umbilic points, we also approximate Q by its value at the origin and thereby assume that Q is constant. In Section 3.4 we prove the following result:

Proposition 2.1. Suppose that system (1.1) is written in the normal form of Schaeffer-Shearer [18] and equipped with a constant viscosity matrix Q that has eigenvalues with positive real parts. Then the following statements are equivalent.

(1) The viscosity matrix Q is strictly stable for system (1.1) at each state U_*¹ 0.

(2) The symmetric part of Q is strictly positive definite.

(3) The function h(U) = |U|² is an entropy for system (1.1) that is compatible with Q.

Therefore we assume that the symmetric part of Q is strictly positive definite. Motivated by Theorem 4.1 below, we also require Q to be symmetric.

2.3 Types of shock waves

Shock waves can be classified in various ways. One natural way is according to the signs of the velocities of the characteristic families for the states U_- and U₊ relative to the shock velocity s, i.e., according to the signs of l₁(U_-) - s, l₂(U_-) - s, l₁(U₊) - s, and l₂(U₊) - s. For example: a Lax 1-shock wave has signs (+,+,-,+); a Lax 1-shock wave that is sonic on the right has signs (+,+,0,+); and a crossing shock wave, through which both families of characteristics cross, has signs (-,+,-,+).

Alternatively, shock waves can be classified in a manner related to the viscosity matrix Q. To this end, let µ_j(U_*,s), j = 1,2, denote the eigenvalues of

labeled so that Re µ₁(U_*,s) < Re µ₂(U_*,s). If U_* is an equilibrium point for system (2.9), then µ₁(U_*,s) and µ₂(U_*,s) are the eigenvalues of the linearization of system (2.9) around the solution (x) º U_*. Moreover, the signs of Re µ₁(U_*,s) and Re µ₂(U_*,s) determine the type of the equilibrium point U_*:

• repeller if 0 < Re µ₁(U_*,s);

• saddle point if Re µ₁(U_*,s) < 0 < Re µ₂(U_*,s);

• attractor if Re µ₂(U_*,s) < 0;

• repeller-saddle if Re µ₁(U_*,s) = 0 < Re µ₂(U_*,s);

• saddle-attractor if Re µ₁(U_*,s) < 0 = Re µ₂(U_*,s).

(As a consequence of Proposition 2.2 below, these are the only possibilities. Also, the names repeller-saddle and saddle-attractor might not reflect the topological type of an equilibrium that is degenerate.) A shock wave can be classified by the signs of Re µ₁(U_-,s), Re µ₂(U_-,s), Re µ₁(U₊,s), and Re µ₂(U₊,s), or equivalently by the equilibrium types of U_- and U₊.

Of course, if Q(U) º I and the states U_- and U₊ are strictly hyperbolic, these two classification schemes coincide. From the perspective of the latter scheme: for a Lax 1-shock wave, U_- is a repeller and U₊ is a saddle point; for a Lax 1-shock wave that is sonic on the right, U_- is a repeller and U₊ is a repeller-saddle; and for a crossing shock wave, U_- and U₊ are saddle points. This is the classification used systematically in Ref. [20].

More generally, the two classification schemes coincide when the states U_- and U₊ are strictly hyperbolic and the viscosity matrix Q is strictly stable at U_- and U₊, as seen from the following result.

Proposition 2.2. Suppose that the state U_* is strictly hyperbolic and the viscosity matrix Q is strictly stable at U_*. Then the signs of Re µ₁(U_*,s) and Re µ₂(U_*,s) coincide with the signs of l₁(U_*) - s and l₂(U_*) - s, respectively.

Proof. Let R(U_*) denote the matrix with columns being the right eigenvectors r₁(U_*) and r₂(U_*), and let L(U_*) denote the matrix with rows being the left eigenvectors 1(U_*) and 2(U_*). Then L(U_*) = R(U_*)^-1. Consider the matrix obtained by multiplying the matrix (2.11) on the right by R(U_*) and on the left by L(U_*). Taking its trace and determinant yields the following identities:

Notice that

By assumption, l₁(U_*) and l₂(U_*) are real and distinct, and det Q(U_*), 1(U_*) Q(U_*) r₁(U_*) and 2(U_*) Q(U_*) r₂(U_*) are positive. Also, µ₁(U_*,s) and µ₂(U_*,s) are complex conjugates if they are not real. Now by considering the several possibilities, one easily verifies, using identities (2.12) and (2.13), that Re µ₁(U_*,s) and Re µ₂(U_*,s) have the same signs as l₁(U_*) - s and l₂(U_*) - s, respectively.

Remark. The coincidence of the shock classification schemes is proved for non-sonic Lax shock waves in n-component conservation laws in Ref. [16, Theorem 2.4] using a different argument; as pointed out to us by Prof. K. Zumbrun, this argument can be augmented to cover sonic waves.

2.4 Non-classical shock waves

Certain shock waves that are admissible under the Lax criterion are also admissible under the viscous profile criterion. However, not all Lax shock waves possess viscous profiles. Moreover, there are shock waves that do not satisfy the Lax criterion and yet have viscous profiles. Such non-classical shock waves, especially transitional shock waves, play an important role in the construction of solutions of Riemann problems.

A transitional [9], or undercompressive [22], shock wave is one with a viscous profile that joins two saddle points. According to Proposition 2.2, a transitional shock wave is an admissible crossing shock wave. In contast to a Lax shock wave, a transitional shock wave is not associated with a particular characteristic family. By the Wave Structure Theorem of Ref. [20], a transitional wave group can only appear in a solution of a Riemann problem situated between the 1- and 2-family wave groups. (In addition to strict hyperbolicity, Ref. [20] assumes that Q(U) º I, but by virtue of Prop. 2.2, the Wave Structure Theorem extends to the situation where, for each state U_* in the Riemann solution, Q(U_*) is strictly stable.) The appearance of transitional shock waves is one of the distinguishing features of viscous profile solutions of Riemann problems. The occurrence of these shock waves in homogeneous quadratic models is discussed in detail in Section 4.

Another type of non-classical shock wave, an overcompressive shock wave [22, 3], has a viscous profile that leads from a repeller to an attractor. Consequently all characteristics impinge on it: l₂(U₊) < s < l₁(U_-). For generic quadratic models, overcompressive waves appear in Riemann solutions only for a subset of Riemann data (U_L, U_R) of codimension one. In this context, overcompressive waves do not play the important role in solving Riemann problems that transitional waves do.

3 Homogeneous quadratic models

In this section, we focus attention on homogeneous quadratic models withisolated umbilic points.

3.1 Schaeffer-Shearer normal form

Using the notation U = (u, v)^T, we can write any system of two conservation laws with a homogeneous quadratic flux as

This system has six free parameters, a₁, b₁, c₁, a₂, b₂, and c₂. Schaeffer and Shearer [18] showed that if the origin is an isolated umbilic point, then such a system can be transformed, by means of invertible linear transformations of state space and the (x, t)-plane, into a system of the form

This normal form has only two free parameters, a and b, subject to the condition that

A defining feature of the normal form (3.2) is that the Jacobian F¢ is symmetric, and therefore F^T equals the gradient, C¢, of a homogeneous cubicpolynomial C.

Schaeffer and Shearer classified quadratic models with isolated umbilic points by dividing the (a, b)-plane into four regions. This classification of models into Cases I-IV is based on the structure of rarefaction curves through the origin. The division of the parameter plane is illustrated in Figure 1. In detail, the curves separating the regions are as follows:

• Case I/II boundary: 4a - 3b² = 0.

• Case II/III boundary: a - b² - 1 = 0.

• Case III/IV boundary: -32b⁴ + [27 + 36(a - 2) - 4(a - 2)²] b² + 4(a - 2)³ = 0.

3.2 Fundamental wave manifold for homogeneous quadratic models

Many of the computations presented in this paper are based on the construction of the fundamental wave manifold , which was introduced for quadratic models by Marchesin and Palmeira [17] and extended to general systems of conservation laws in Ref. [8]. Points of represent shock wave solutions of the conservation laws. To each point in is associated a dynamical system, viz., Eq. (2.9); whether or not a connection exists determines whether or not the shock is admissible.

The wave manifold is constructed by considering solutions (U_-, U₊, s) of the Rankine-Hugoniot condition (2.2). Although the solution set has a singularity at each point where U_- = U₊, this singularity can be removed by introducing a new set of variables (, R, j, s). Here = (, )^T is the midpoint (U_- + U₊) between U₊ and U_-, whereas R Î and j Î (-p/2, p/2] represent the separation and orientation of the vector U₊ - U_-. More precisely, (, R, j, s) is mapped to (U_-, U₊, s) through the relationships

This map remains smooth if each point (, R, p/2, s) is glued to the point (, - R, -p/2, s), so we regard its domain as ² × M₂ × , where M₂ is the Möbius strip parameterized by R and j. Expressed in terms of (, R, j, s), the Rankine-Hugoniot condition contains an explicit factor R, corresponding to trivial solutions U₊ = U_-, which we eliminate. The remaining equation defines .

For homogeneous quadratic models, the Rankine-Hugoniot condition is equivalent to

with F¢ being linear. Solutions satisfy either R = 0 or

where a, b, , and are homogeneous quadratic polynomials in cos j and sin j defined by

The wave manifold comprises points (, , R, j, s) satisfying Eqs. (3.6) and (3.7).

For the normal form (3.2), we have

The vector (a(j), b(j)) never vanishes, by virtue of condition (3.3); indeed, the resultant of a and b is -(a - b² - 1)². Therefore Eqs. (3.6) and (3.7) are solved by

where k Î and

For the normal form (3.2),

In summary, for a homogeneous quadratic model with an isolated umbilic point, points in the wave manifold can be parameterized by the variables (R, k, j) Î × ×(-p/2, p/2], with each point (R, k, p/2) glued to the point (-R, k, -p/2). The shock wave corresponding to (R, k, j) is obtained in the following way:

Equations (3.19) and (3.20) define two projections from to state space: the U_--projection p_- (R, k, j) (u_-, v_-) and the U₊-projection p₊: (R, k, j) (u₊, v₊).

We now define some additional expressions that will be used later:

For the normal form (3.2), (j) º a - b² - 1 is a nonzero constant,

and B (j) is simply (a - b²) - 1) 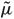(j).

Remark. Even for general quadratic models, and are homogeneous cubic polynomials, and B is a homogeneous quadratic polynomial, in cos j and sin j.

3.3 Subsets of the fundamental wave manifold

3.3.1 Characteristic manifold

The characteristic manifold, denoted by C, is the R = 0 slice of the wave manifold. For a point in C, U_- = U₊ = and s is an eigenvalue of F¢() (see Eq. (3.5) with the factor R removed). If a state is such that the eigenvalues of F¢() are real and distinct, then there are two different points in C that project to . In this case, C is a two-fold covering of a sufficiently small neighborhood of .

3.3.2 Sonic loci

The right sonic locus, S_R, is the closure in of the set of nontrivial shock waves such that l_i(U₊) = s, i = 1 or 2; the left sonic locus, S_L, is defined analogously by the condition l_i(U_-) = s, i = 1 or 2. For homogeneous quadratic models, the right sonic locus (respectively, left sonic locus) consists of points (R, k, j) that satisfy

(See Ref. [24, pp. 316-317] for the proof.) The left sonic locus is the reflection, through the (k, j)-plane, of the right sonic locus, which is characterized as follows.

Lemma 3.1. If 4a ¹ 3b², S_R is a ruled surface, in that its intersection with each plane j = const. is a line. If 4a = 3b², S_R is the union of such a ruled surface with the plane j = -tan^-1(b/2). The same is true of S_L.

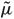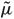Proof. The resultant of and = B/(a - b² - 1) is (4a - 3b²)². Therefore, if 4a ¹ 3b², the vector ((j), B(j)) never vanishes. Thus, for each j, the solution set of Eq. (3.28) (with the upper sign) is a line. If, on the other hand, 4a = 3b², then (j) = [(b/2) cos j + sin j]² and (j) = 3(j) cos j, so that the solution set is the union of the plane (j) = 0 with the ruled surface

3.3.3 Inflection locus

A point in the inflection locus lies in the characteristic manifold C and corresponds to a point in state space at which genuine nonlinearity fails. In general [8], the inflection locus is the common intersection of the sonic loci S_L and S_R with C. From Eq. (3.28) we see that points in the inflection locus have R = 0 and either k = 0 or B(j) = 0. Thus the inflection locus comprises the j-axis (R = 0 and k = 0) and the lines with R = 0 and j = j_i; here j_i is an inflection angle, i.e., a root of B(j) = 0. The formula (3.27) for 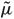= B/(a - b² - 1) shows that the number of inflection angles is three if 4a < 3b², two if 4a = 3b², and one if 4a > 3b².

3.3.4 Double sonic locus

The double sonic locus is the closure of the set of nontrivial shock waves that lie on both S_Land S_R. According to Eqs. (3.28) and Lemma 3.1: if 4a ¹ 3b², the double sonic locus comprises the lines with k = 0 and j = j_d, where j_d is a double sonic angle, i.e., a root of (j); and if 4a = 3b², the double sonic locus is the plane j = -tan^-1(b/2). The formula (3.18) for (j) shows that there are no double sonic angles if 4a < 3b² and there are two if 4a > 3b².

3.4 Proof of Proposition 2.1.

The following proof is an application of the formalism just developed.

Proof of Proposition 2.1. To prove the equivalence of statements (1) and (2), we invoke results from Ref. [24]. Proposition 5.12 of this reference states that the viscosity matrix Q is strictly stable for system (1.1) at a state U_* if and only if the quantities tr[-s I + F¢(U_*)] and tr{Q^-1[-s I + F¢(U_*)]} have the same (nonzero) sign for each eigenvalue s of F¢(U_*). Moreover, Theorem 5.8 of this reference implies that if U_* and s are associated with a point (k, j) on the characteristic manifold C for a homogeneous quadratic model, then

here (j)₂₁ and (j)₂₂ are the (2,1)- and (2,2)-components, respectively, of the matrix

where

Consequently the criterion for strict stability of Q at U_* is that, for each corresponding point (k, j) on C, k (j) and k[-(j)₂₁¢(j) + (j)₂₂(j)] have the same nonzero sign. For a quadratic model written in the normal form (3.2), is a nonzero constant. Therefore the viscosity matrix Q is strictly stable at U_* if and only if k ¹ 0 and (j)₂₂ > 0 for each corresponding point (k, j) on C. Thus the viscosity matrix Q is strictly stable at all states U_*¹ 0 if and only if

for all j, or equivalently that the symmetric part of Q is strictly positive definite.

Because a quadratic model in the normal form (3.2) has a symmetric Jacobian matrix F¢, the equivalence of statements (2) and (3) is well known (see, e.g., Ref. [23, p. 398]). Indeed, a function h is an entropy if and only if F¢ is symmetric with respect to the quadratic form h¢¢, and h is compatible with Q if and only if the symmetric part of Q is strictly positive definite with respect to h¢¢; but if h(U) = |U|², then h¢¢ (U) º I.

4 Transitional regions

In this section, we describe the subset of corresponding to transitional shock waves. This subset depends sensitively on the viscosity matrix Q. We consider quadratic models in Schaeffer-Shearer normal form (3.2) equipped with symmetric, strictly positive definite viscosity matrices. Admissibility of shock waves is not affected when Q is multiplied by a positive scale factor, so we are free to write

where k > 0 and k > j².

4.1 Transitional waves

In general, it is difficult to characterize the set of transitional shock waves. However, the following result greatly simplifies this characterization for homogeneous quadratic models with isolated umbilic points. The proof of this result, which is based on a theorem of Chicone [2], is due to Freistühler and Zumbrun [4].

Theorem 4.1. Suppose that system (1.1) is written in the normal form of Schaeffer-Shearer and equipped with a constant, symmetric, positive definite viscosity matrix Q. Then the orbit for any transitional wave is a straight line segment.

Proof. Multiplying the dynamical system (2.9) by Q^-1/2 and substituting = Q^-1/2, we obtain the system

According to Ref. [18], there exists a homogeneous cubic polynomial C of U such that F = (C¢)^T. Let

Then

Thus the dynamical system (2.9) is linearly equivalent to a quadratic gradient system. For such a system, a saddle-saddle connection lies along a straight line [2].

4.2 Straight-line connections

From Ref. [9], we have the following result concerning straight-line connections (for any type of shock wave).

Proposition 4.2. Let F be quadratic, and suppose that s, U_-, and U₊¹ U_- satisfy the Rankine-Hugoniot condition. Then the straight line segment between U_- and U₊ is a connecting orbit if and only if there is a constant s ¹ 0 such that DU = U₊ - U_- satisfies

The orbit is traversed from U_- to U₊ if and only if s < 0.

In terms of coordinates for , DU = R(cos j, sin j)^T, so that Eq. (4.5) holds for some s if and only if

The right-hand side of Eq. (4.6) resembles µ(j), defined by Eq. (3.23), except that F¢¢(0) is replaced with Q^-1F¢¢(0). We therefore denote the right-hand side of Eq. (4.6) by µ_Q(j). A root j_v of µ_Q(j) is called a viscosity angle. Thus if two states U_- and U₊ are connected by a straight-line connection, then that orbit lies at a viscosity angle in state space. The expression µ_Q(j) is a homogeneous cubic polynomial in cos j and sin j; in the generic case that the discriminant of µ_Q is nonzero, the number of viscosity angles is either one or three.

Proposition 4.2 also states that the straight-line connection is oriented from U_- to U₊ when s < 0. Again because DU = R(cos j, sin j)^T, where j = j_v is a viscosity angle,

which we write as

Thus the orbit orientation condition s < 0 can be written

If

_Q(j_v) = 0, the viscosity angle j_v is said to be exceptional [9]. At an exceptional viscosity angle, no straight-line connections are possible, since s = 0. Exceptional viscosity angles occur when a viscosity angle coincides with a root of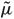 _Q(j). This situation is avoided in the generic case that the resultant of µ_Q and 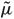_Q is nonzero.

4.3 Geometry of transitional surfaces

We define the transitional surface to comprise points in the wave manifold corresponding to transitional shock waves, i.e., shock waves with viscous profiles that connect saddle points. For the quadratic models and viscosity matrices we consider, any transitional shock wave has a straight-line connection (Theorem 4.1). To determine the transitional surface, we seek straight-line connections joining saddle points.

In the previous subsection, we saw that a point in corresponds to a shock wave with a straight-line connection if and only if j = j_v is a viscosity angle. A cross-section j = j_v of the wave manifold is called a viscosity plane; there are from one to three viscosity planes. Thus the transitional surface is contained in the union of the viscosity planes and may consist of several disjoint connected components. However, not every viscosity plane contains a component of the transitional surface. We say that a viscosity angle is active if the corresponding viscosity plane contains a component of the transitional surface.

Consider the shock types of points of . The signs of l₁(U_-) - s and l₂(U_-) - s are nonzero except at the characteristic manifold C and the left sonic locus S_L, and the signs of l₁(U₊) - s and l₂(U₊) - s are nonzero except at C and the right sonic locus S_R. Therefore the shock type is constant throughout connected regions of \(C È S_L È S_R ). As transitional waves have the shock type (-, +, -, +), one consequence is the following.

Lemma 4.3. Each connected component of the transitional surface is a connected component of a viscosity plane with the sonic loci and the characteristic manifold removed.

According to Lemma 3.1, either S_L and S_R contain the viscosity plane (if 4a = 3b² and j_v = -tan^-1(b/2)) or they intersect the viscosity plane in two lines through the origin that are reflections of each other through the line R = 0. In the former case, no transitional waves lie in the viscosity plane. Otherwise, after removing C, S_L, and S_R from the viscosity plane, several open sectors remain. In the following lemma, we determine which of these open sectors can be a component of the transitional surface.

Lemma 4.4. Any component of the transitional surface contained in the viscosity plane j = j_v intersects the line (j_v) along which k = 0 and j = j_v.

Proof. Depending of the configuration of S_L, S_R, and C in the viscosity plane, two, four, or six open sectors remain. These possibilities are illustrated in Figure 2.

Case (a):C, S_L, and S_R intersect the viscosity plane in a single line (i.e., j_v is an inflection angle). The two remaining open half planes both intersect the line (j_v).

Case (b):C, S_L, and S_R intersect the viscosity plane in three distinct lines. Consider a point with R ¹ 0 lying on (j_v). Since k = 0, the velocity s = (j_v)k of the associated shock wave is zero. For the same reason, the U_- and U₊ projections of this point are

Thus U₊ = -U_-, which implies that F¢(U₊) = -F¢(U_-), l₁(U₊) = -l₂(U_-), and l₂(U₊) = -l₁(U_-). With these restrictions on the shock and characteristic velocities, this point can correspond to one of only three possible shock wave types: transitional (-, +, -, +), overcompressive (+, +, -, -), or totally expansive (-, -, +, +).

If neither of the open sectors intersecting the line (j_v) contains transitional shock waves, then one of the other open sectors must. Thus a sector containing transitional waves is separated from a sector containing either overcompressive or totally expansive waves by a single sonic surface. This, however, is impossible since, in crossing a single sonic surface, only one of the four quantities l₁(U_-) - s, l₂(U_-) - s, l₁(U₊) - s, or l₂(U₊) - s changes sign.

Case (c):C, S_L, and S_R intersect the viscosity plane in two distinct lines. In this case, the sonic loci both intersect the viscosity plane in the line k = 0, i.e., j_v is a double sonic angle ((j_v) = 0). Also, B(j_v) ¹ 0, since otherwise the entire viscosity plane is sonic according to Eq. (3.28). Thus there is no open sector containing the line (j_v). We show that no transitional shock waves occur in this viscosity plane.

Consider two points A and B in the open quadrants of the viscosity plane that are reflections across k = 0. According to Eqs. (3.19) and (3.20), the U_- and U₊ projections of A and B satisfy

Because (j_v) = 0, the velocity of a shock wave corresponding to any point in this viscosity plane is zero. From Eqs. (4.11) and (4.12), we also deduce that l₁() = -l₂(), l₁() = -l₂(), l₂() = -l₁(), and l₂() = -l₁(). With these restrictions on the characteristic velocities, we see that if the point A corresponds to a transitional shock wave, then the point B must also correspond to a transitional shock wave. However, this is impossible because A and B are separated only by the left and right sonic loci. Therefore there are no transitional shock waves and the viscosity plane does not contain a component of the transitional surface in case (c).

Lemma 4.4 does not specify which of the two open sectors containing (j_v) is a component of the transitional surface. Recall from Eq. (4.8) that the sign of s, which determines the orientation of the connecting orbit, depends on the sign of R. The open sector that gives rise to correctly oriented orbits is the transitional sector, determined by condition (4.9). In Section 5.2, we determine sgn 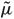_Q(j_v) precisely.

In the following theorem, we present the conditions that a viscosity angle must satisfy in order to be active.

Theorem 4.5. A viscosity angle j_v is active if and only if the following conditions are satisfied:

(1)

_Q(j_v) ¹ 0, i.e., j_v is not exceptional; and

(2)(j_v) > 0.

Moreover, the corresponding transitional sector is the one containing the line (j_v) along which k = 0 and j = j_v and having sgn R = -sgn 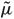_Q (j_v).

Proof. Suppose that j_v is active; we examine the viscosity plane associated with this angle. Condition (1) holds, as otherwise no straight-line connection occurs at j_v by virtue of Prop. 4.2 and Eq. (4.8). Condition (2) is established as follows.

From Lemma 4.4, we know that only an open sector containing the line (j_v) can possibly be a component of the transitional surface. Consider the projections U₊ and U_- = -U₊ of a point on this line with R ¹ 0. As its shock speed is zero, this point corresponds to a transitional shock wave if and only if the eigenvalues of F¢(U_-) have opposite signs and the eigenvalues of F¢(U_-) have opposite signs. As F¢(U_-) = -F¢(U₊), this condition amounts to det(F¢(U₊)) < 0. We show that this inequality is equivalent to

Because F is a homogeneous quadratic polynomial, we have that F¢(U₊) = F¢¢(0)U₊. As R ¹ 0, the condition det(F¢(U₊)) < 0 is equivalent to

Inserting a rotation matrix does not affect the determinant; therefore the inequality (4.14) is satisfied if and only if the following inequality holds:

The entries of the matrix appearing in this inequality may be identified by differentiating expressions (3.8)-(3.9) with respect to . Thus we can rewrite inequality (4.15) as

which reduces to

The left-hand side is - (j_v), so that det(F¢(U₊)) < 0 if and only if condition (2) holds.

Conversely, if conditions (1) and (2) hold, then a point with R ¹ 0 on the line (j_v) provides an example of a transitional shock wave, so that the viscosity angle j_v is active.

When j_v is active, Lemma 4.4 and Eq. (4.9) determine the transitional sector.

4.4 Projection to state space

The transitional region is the U_--projection of the transitional surface into state space, i.e., the image of the transitional surface under the linear map p_- defined by Eqs. (3.19) and (3.20) with the lower sign. By the foregoing results, the transitional region consists of from one to three wedges centered at the origin, called the transitional wedges, which are associated to the different viscosity angles.

As restricted to a viscosity plane j = j_v, the projections p₊ and p_- defined by Eqs. (3.19) and (3.20) can be written as

and

respectively. Suppose that the matrices P₊ and P_- appearing, respectively, in Eqs. (4.18) and (4.19) are nonsingular; this is true if and only if a(j_v) cos(j_v) + b(j_v) sin(j_v) ¹ 0, i.e.,

Then the map U_-

U₊ = P₊(P_-)^-1U_-, defined for states U_- in the transitional wedge corresponding to viscosity angle j_v, is called the transitional map for j_v. (See Fig. 3.) A state U_- in the transitional wedge and its image U₊ under the transitional map are the end states of a transitional shock wave with a straight-line orbit.

There are situations, however, in which µ(j_v) = 0. For instance, if Q = I, then µ = µ_Q vanishes at any viscosity angle. When µ(j_v) = 0, no transitional map is defined. Instead, the transitional wedge for j_v degenerates to a ray, and the preimage under P_- of a point U_- on this ray is a line segment in the transitional plane j = j_v, which is mapped via P₊ to a line segment of states U₊, as in Figure 4. (By Eq. (3.11), the kernels of P₊ and P_- never coincide when µ(j_v) = 0.) There is a transitional shock wave from U_- to each state U₊ lying on this line segment.

Remark. An angle j satisfying µ(j) = 0 is called a bifurcation angle; such angles determine the secondary bifurcation loci in the wave manifold.

5 Classification of models

In this section, we determine the boundaries in the parameter plane that separate models with differing numbers of transitional regions. Many of the calculations presented in this section were carried out with the aid of the Maple software package [1].

5.1 Classification according to the active region criterion

Motivated by Theorem 4.5, we now determine the parameter values such that the condition (j_v) > 0 is satisfied. Recall that a viscosity angle j_v is a root of µ_Q(j). Usually, each j_v can be regarded as a function of the parameters of the model and the viscosity matrix. For each j_v, there are curves in the parameter plane which separate those models for which (j_v) > 0 from those for which (j_v) < 0. These curves represent those models for which (j_v) = 0, i.e., where (j) and µ_Q(j) have coincident roots. In order to determine these curves, we consider the resultant of (j) and µ_Q(j), which vanishes if and only if the two polynomials have at least one coincident root. Direct calculation using Maple shows that

Each of the two factors of resultant (µ_Q, ) can vanish, and in Figure 5 we have drawn the zero-sets of these two factors in the (a, b)-plane for a particular viscosity matrix. In this figure, we have also drawn the curve representing the zero-set of the discriminant of µ_Q, which we compute below; upon crossing this curve from left to right, the number of roots of µ_Q changes from three to one. The relative placement of the three curves remains the same as we vary the viscosity matrix because the three curves do not intersect, as we verify in the following two lemmas.

Lemma 5.1. The curves 4a - 3b² = 0 and a - (b² + bj + k) = 0 do not intersect.

Proof. Substituting a = 3b²/4 into the expression b² + bj + k - a yields

which is positive if k - j² > 0. Hence, the two curves do not intersect.

Lemma 5.2. The curves a - (b² + bj + k) = 0 and discriminant(µ_Q) = 0 do not intersect.

Proof. Using Maple, we find that the discriminant of µ_Q to be

After substituting a = b² + bj + k, expression (5.3) simplifies to

which is positive if k - j² > 0. Hence, the two curves do not intersect.

In fact, the proofs above establish that the curves 4a - 3b² = 0, a - (b² + bj + k) = 0, and discriminant(µ_Q) = 0 are ordered from left to right, in the sense that, for each b, the a coordinates of points on the curves are so ordered. Therefore we can make the following definition.

Definition 5.3. We denote the region where a < 3b²/4 by I_Q; the region where 3b²/4 < a < b² + bj + k by II_Q; the region where b² + bj + k < a and discriminant(µ_Q) > 0 by III_Q; and the region where discriminant(µ_Q) < 0 by IV_Q.

Remark. The I_Q/ II_Q boundary is exactly the Schaeffer-Shearer Case I/II boundary; and when Q = I, the II_Q/ III_Q and III_Q/ IV_Q boundaries reduce to the Case II/III and Case III/IV boundaries, respectively.

We now proceed to the main result of this section.

Theorem 5.4. In region I_Q, there are three active viscosity angles; in region II_Q, there are two active viscosity angles; and in regions III_Q and IV_Q, there are no active viscosity angles.

Proof. Within each region, the number of roots of µ_Q(j) and the ordering of the roots of (j) and µ_Q(j) along (-p/2, p/2] are the same. To prove the theorem, we choose a simple representative model within each region and examine the roots of the two polynomials for this model.

We let b = 0 and Q = I, i.e., k = 1 and j = 0. For these parameter values, we have a < 0 in region I_Q, 0 < a < 1 in region II_Q, 1 < a < 2 in region III_Q, and a > 2 in region IV_Q. The roots of µ_Q(j) are

and the values of (j) at these angles are

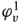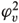In region I_Q, we have (j) > 0 for j = , , and ; hence, all three viscosity angles are active. In region II_Q, we have (j) > 0 for j = 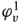and j = only; hence, only two of the viscosity angles are active. In region III_Q, we have (j) < 0 for j = 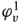, , 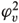and j = ; hence, there are no active viscosity angles. Finally, in region IV_Q, we have (j) < 0 for the only viscosity angle j = 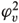; hence, there are no active viscosity angles.

In the region in the parameter plane corresponding to three real viscosity angles, we can order the angles as 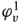< < 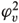within (-p/2, p/2]. Upon crossing the 4a - 3b² boundary, it is 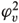that no longer satisfies condition (4.13).

5.2 Classification according to the orientation criterion

We now determine precisely which open sector in a viscosity plane is a transitional surface. Recall from Theorem 4.5 that the open sector satisfying the condition sgn R = -sgn 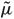_Q(j_v) corresponds to properly oriented transitional shock waves. To use this condition, we must determine the sign of 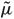_Q evaluated at a viscosity angle j_v. Using Maple, we have the following expression:

Because the viscosity angles are exactly the roots of µ_Q, the zero-set of the resultant of µ_Q and 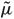_Q comprises curves on which 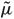_Q(j_v) = 0 for some viscosity angle j_v, i.e., some viscosity angle is exceptional. The first factor vanishes at the Schaeffer-Shearer Case I/II boundary, which is the I_Q/II_Q boundary. The second factor never vanishes since Q is positive definite, and the third factor plays no role, according to the following lemma.

Lemma 5.5. The expression

vanishes only in the region in the parameter plane where there are no active viscosity angles.

Proof. Expression (5.10) is a quadratic polynomial in a, the discriminant of which is

Therefore expression (5.10) has imaginary roots (and hence never vanishes) except when the discriminant (5.11) is zero. The discriminant vanishes when

For this value of b, expression (5.10) vanishes when

We are concerned only with active viscosity angles; therefore we shall determine whether this value of a satisfies a < b² + bj + k when Eq. (5.12) holds.

After some algebraic manipulations, we see that a < b² + bj + k if and only if

However, the left-hand side can be rewritten as

which is manifestly positive. Therefore any value of a that would make the factor (5.10) vanish lies in the region where there are no viscosity angles.

From this lemma, we see that

_Q(j_v) changes sign only across the Case I/II boundary. At this boundary, we have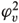 coinciding with a root of 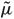_Q(j). (See the discussion after Theorem 5.4.) For a > 3b²/4, j²_v does not correspond to a transitional region, so we do not consider it; however, when a < 3b²/4, we do need to consider 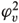. As in the proof of Theorem 5.4, we choose the simple representative model, b = 0 and Q = I, and examine the sign of 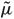_Q(j) at each of the viscosity angles. When a < 3b²/4, we have 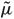_Q(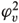) < 0, since a < 0. The other two viscosity angles 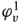and give 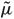_Q > 0 for models with transitional shock waves.

Therefore for all models with transitional shock waves, we have

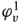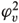_Q(j) > 0 for and , and for models where is an active viscosity angle, we have 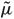_Q(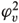) < 0. This concludes the proof of the following theorem.

Theorem 5.6. For all models, in the viscosity planes corresponding to

and

, the transitional sector is the sector containing the ray on which k = 0 and R < 0. For models having

as an active viscosity angle, in the viscosity plane corresponding to

, the transitional sector is the sector containing the ray on which k = 0 and R > 0.

6 Conclusion

We have studied a class of quadratic models to understand how perturbations of the viscosity term affect solutions of the Riemann problem. We considered models in the Schaeffer-Shearer normal form, and used the viscous profile criterion as the admissibility criterion. The viscosity matrices we used were symmetric and positive definite.

Our primary focus has been the behavior of transitional shock waves. For these models, we have precisely described the transitional surface — the subset of the wave manifold that corresponds to transitional shock waves. The transitional surface consists of components that lie in planes that correspond to active viscosity angles. We have derived a condition for determining exactly when a viscosity angle is active. With this condition, we have determined the boundaries (in terms of the model and viscosity matrix parameters) where the number of active viscosity angles changes.

The results we have concerning the effects of viscous terms on the solutions of Riemann problems are primarily analytical. However, they have immediate implications for numerical analysis. In finite-difference solvers for conservation laws, the use of artificial viscosity is common. From our work, we can see that different viscosity matrices will result in different solutions; hence, care must be chosen to ensure that the form of the viscosity accurately reflects the physics of the problem being modeled.

Acknowledgments. This work has been supported in part by: the Henry Luce Foundation through a Clare Boothe Luce Professorship; the NSF under Grant DMS-9732876; the DOE under Grant DE-FG02-90ER25084; MCT under Grant PCI 650009/97-5; FAPERJ under Grant E-26/150.936/99; CNPq under Grant 451055/00-4; and FINEP under Grant 77970315-00.

We thank Profs. H. Freistühler and K. Zumbrun for providing the proof of Theorem 4.1. We also thank Prof. D. Marchesin and the Instituto de Matemática Pura e Aplicada for generous support during the writing of the manuscript.

Received: 26/VI/06. Accepted: 25/I/07.

#676/06.

• [1] B. Char, K. Geddes, G. Gonnet, B. Leong, M. Monagan and S. Watt, Maple V Library Reference Manual Springer-Verlag, New York-Heidelberg-Berlin, 1991.
• [2] C. Chicone, Quadratic gradients on the plane are generically Morse-Smale. J. Differential Equations, 33 (1979), 159-166.
• [3] H. Freistühler, Instability of vanishing viscosity approximation to hyperbolic systems of conservation laws with rotational invariance. J. Differential Equations, 87 (1990), 205-226.
• [4] H. Freistühler and K. Zumbrun, Private communication, 1996.
• [5] I. Gelfand, Some problems in the theory of quasi-linear equations. Uspekhi Mat. Nauk, 14 (1959), 87-158.
• [6] J. Hurley, Effects of Viscous Terms on Solutions of Riemann Problems PhD thesis, State Univ. of New York at Stony Brook, 1995.
• [7] J. Hurley and B. Plohr, Some effects of viscous terms on Riemann problem solutions. Mat. Contemp., 8 (1995), 203-224.
• [8] E. Isaacson, D. Marchesin, C.F. Palmeira and B. Plohr, A global formalism for nonlinear waves in conservation laws. Comm. Math. Phys., 146 (1992), 505-552.
• [9] E. Isaacson, D. Marchesin and B. Plohr, Transitional waves for conservation laws. SIAM J. Math. Anal., 21 (1990), 837-866.
• [10] E. Isaacson, D. Marchesin, B. Plohr and J.B. Temple, The Riemann problem near a hyperbolic singularity: the classification of quadratic Riemann problems I. SIAM J. Appl. Math., 48 (1988), 1009-1032.
• [11] E. Isaacson and J.B. Temple, The Riemann problem near a hyperbolic singularity II. SIAM J. Appl. Math., 48 (1988), 1287-1301.
• [12] E. Isaacson and J.B. Temple, The Riemann problem near a hyperbolic singularity III. SIAM J. Appl. Math., 48 (1988), 1302-1312.
• [13] P. Lax, Hyperbolic systems of conservation laws II. Comm. Pure Appl. Math., 10 (1957), 537-566.
• [14] P. Lax, Shock waves and entropy. In E. Zarantonello, editor, Contributions to Nonlinear Functional Analysis, pages 603-634. Academic Press, New York, 1971.
• [15] T.-P. Liu, The Riemann problem for general 2×2 conservation laws. Trans. Amer. Math. Soc., 199 (1974), 89-112.
• [16] A. Majda and R. Pego, Stable viscosity matrices for system of conservation laws. J. Differential Equations, 56 (1985), 229-262.
• [17] D. Marchesin and C.F. Palmeira, Topology of elementary waves for mixed systems of conservation laws. J. Dynamics Differential Equations, 6 (1994), 427-446.
• [18] D. Schaeffer and M. Shearer, The classification of 2×2 systems of non-strictly hyperbolic conservation laws, with application to oil recovery. Comm. Pure Appl. Math., 40 (1987), 141-178.
• [19] D. Schaeffer and M. Shearer, Riemann problems for nonstrictly hyperbolic 2×2 systems of conservation laws. Trans. Amer. Math. Soc., 304 (1987), 267-306.
• [20] S. Schecter, D. Marchesin and B. Plohr, Structurally stable Riemann solutions. J. Differential Equations, 126 (1996), 303-354.
• [21] M. Shearer, The Riemann problem for 2×2 systems of hyperbolic conservation laws with Case I quadratic nonlinearities. J. Differential Equations, 80 (1989), 343-363.
• [22] M. Shearer, D. Schaeffer, D. Marchesin and P. Paes-Leme, Solution of the Riemann problem for a prototype 2×2 system of non-strictly hyperbolic conservation laws. Arch. Rational Mech. Anal., 97 (1987), 299-320.
• [23] J. Smoller, Shock Waves and Reaction-Diffusion Equations Springer-Verlag, New York, second edition, 1994.
• [24] S. Canic and B. Plohr, Shock wave admissibility for quadratic conservation laws. J. Differential Equations, 118 (1995), 293-335.
• [25] J. Wenstrom, Solutions of Riemann problems with quadratic flux functions. In preparation, 2007.

Publication Dates

• Publication in this collection
  23 July 2007
• Date of issue
  2007

History

• Accepted
  25 Jan 2007
• Received
  26 June 2006